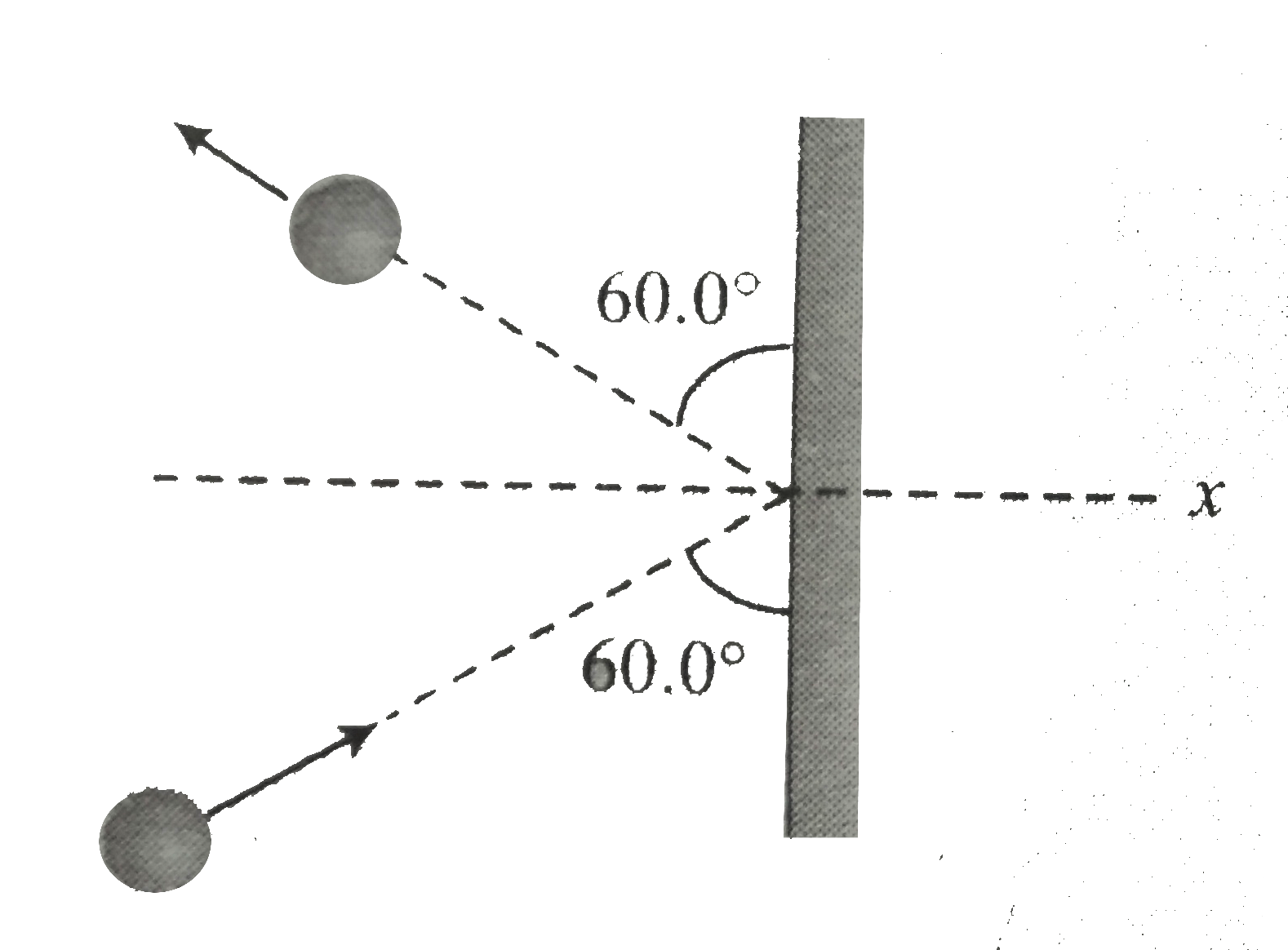
A 3.00kg steel hall strikes the wall with a speed of 10.0m/s at an angle of 60∘ with the surface. It bounces off with the same speed and angle. If the ball is in contact with the wall for 0.200s, what is the average force exerted by the wall on the ball?
To solve the problem, we will follow these steps:
Step 1: Identify the components of velocity
The ball strikes the wall at an angle of 60∘ with the surface at a speed of 10.0m/s. We need to resolve this velocity into two components:
- Perpendicular to the wall: V⊥=Vsin(60∘)
- Parallel to the wall: V∥=Vcos(60∘)
Step 2: Calculate the components
Using V=10.0m/s:
- V⊥=10.0sin(60∘)=10.0×√32=5√3m/s
- V∥=10.0cos(60∘)=10.0×12=5.0m/s
Step 3: Determine the change in momentum
The momentum before and after the collision can be analyzed:
- Initial momentum perpendicular to the wall: pinitial=mV⊥=3.00kg×5√3m/s
- Final momentum perpendicular to the wall: Since the ball bounces back, the final momentum is pfinal=−mV⊥=−3.00kg×5√3m/s
The change in momentum (Δp) is given by:
Δp=pfinal−pinitial=−3.00×5√3−(3.00×5√3)=−6.00×5√3
This results in:
Δp=−6.00×5√3=−30√3kg m/s
Step 4: Calculate the average force
The average force exerted by the wall on the ball can be calculated using the formula:
Favg=ΔpΔt
Given that Δt=0.200s:
Favg=−30√30.200
Calculating this gives:
Favg=−150√3N
The negative sign indicates the direction of the force is opposite to the initial momentum direction. The magnitude of the force is:
Favg≈150×1.732=259.8N
Final Answer
The average force exerted by the wall on the ball is approximately 259.8N.
---
The ball strikes the wall at an angle of 60∘ with the surface at a speed of 10.0m/s. We need to resolve this velocity into two components:
- Perpendicular to the wall: V⊥=Vsin(60∘)
- Parallel to the wall: V∥=Vcos(60∘)
Using V=10.0m/s:
- V⊥=10.0sin(60∘)=10.0×√32=5√3m/s
- V∥=10.0cos(60∘)=10.0×12=5.0m/s
The momentum before and after the collision can be analyzed:
- Initial momentum perpendicular to the wall: pinitial=mV⊥=3.00kg×5√3m/s
- Final momentum perpendicular to the wall: Since the ball bounces back, the final momentum is pfinal=−mV⊥=−3.00kg×5√3m/s
Δp=pfinal−pinitial=−3.00×5√3−(3.00×5√3)=−6.00×5√3
This results in:
Δp=−6.00×5√3=−30√3kg m/s
The average force exerted by the wall on the ball can be calculated using the formula:
Favg=ΔpΔt
Given that Δt=0.200s:
Favg=−30√30.200
Calculating this gives:
Favg=−150√3N
The negative sign indicates the direction of the force is opposite to the initial momentum direction. The magnitude of the force is:
Favg≈150×1.732=259.8N
The average force exerted by the wall on the ball is approximately 259.8N.
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Subjective
23 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Single Correct
141 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.2
23 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
A 3-kg steel ball strikes a wall with a speed of 10.0ms−1 at an angle of 60.0∘ with the surfaces of the wall. The ball bounces off with the same speed and same angle. If the ball was in contact with the wall for 0.2s, find the average force exerted by the wall on the ball.
View SolutionA 0.5kg ball moving with a speed of 12m/s strikes a hard wall at an angle of 30∘ with the wall. It is reflected with the same speed and at the same angle . If the ball is in contact with the wall for 0.25s, the average force acting on the wall is

View Solution
Knowledge Check
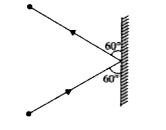
A 3 kg ball strikes a heavy rigid wall with a speed of 10 m/s at an angle of 60∘. It gets reflected with the same speed and angle as shown in Fig. 15.3.11. It the ball is in contact with the wal for 0.20 s, what is the average force exerted on the ball by the wall ?
 AzeroB150 NC150 √3 ND300 N
AzeroB150 NC150 √3 ND300 N
A ball of mass 3kg moving with a speed of 100 m/s, strikes a wall at an angle 60∘ (as shown in figure). The ball rebounds at the same speed and remains in contact with the wall for 0.2 s, the force exerted by the ball on the wall is

View SolutionA 1 kg particle strikes a wall with velocity 1 m/s at an angle of 60o with the wall and reflects at the same angle. If it remains in contact with the wall for 0.1 s, then the force is
View SolutionA ball weighing 10g hits a hard surface vertically with a speed of 5m/s and rebounds with the same speed The ball remains in contact with the surface speed The ball remains in contact with the surface for 0.01s The average force exerted by the surface on the ball is .
View SolutionA ball of mass 0.5kg moving with a velocity of 2m/s strikes a wall normally and bounces back with the same speed . If the time of contact between the ball and the wall is 1 millisecond , the average force exerted by the wall on the ball is
View SolutionA ball of mass 0.5kg moving with a velocity of 2m/s strikes a wall normally and bounces back with the same speed . If the time of contact between the ball and the wall is 1 millisecond , the average force exerted by the wall on the ball is
View SolutionA golf player hits a golf ball of mass 100 g and imparts it a speed of 50 m/s. If golf stick remains in contact with the ball for 0.01 s, then the average force exerted by the golf stick on the ball.
View SolutionA ball is thrown at a speed of 40 m/s at an angle of 600 with the horizontal. Find a. the maximum height reached and b. the range of te ball. Take g=10ms2.
View Solution
