A ball strickes a horizontal floor at an angle θ=45∘ with the normal to floor. The coefficient of restitution between the ball and the floor is e=1/2. The fraction of its kinetic energy lost in the collision is.
58
38
34
14
To solve the problem step by step, we need to analyze the collision of the ball with the floor and calculate the fraction of kinetic energy lost.
Step 1: Break down the initial velocity into components
The ball strikes the floor at an angle of θ=45∘ with the normal. We can denote the initial velocity of the ball as v. The components of the velocity can be calculated as follows:
- The vertical component (along the normal) is vy=vcos(45∘)=v√2.
- The horizontal component (parallel to the floor) is vx=vsin(45∘)=v√2.
Hint: Remember that the angle with the normal is complementary to the angle with the horizontal.
Step 2: Apply the coefficient of restitution
The coefficient of restitution e is given as 12. The coefficient of restitution relates the velocities before and after the collision:
e=Velocity of separationVelocity of approach
The velocity of approach in the vertical direction is vy=v√2. After the collision, the vertical component of the velocity will be v′y (which remains the same in magnitude but changes direction). The horizontal component will change due to the collision, denoted as v′x.
Using the coefficient of restitution:
e=v′xvy⟹12=v′xv√2
From this, we can solve for v′x:
v′x=12⋅v√2=v2√2
Hint: The coefficient of restitution only affects the component of velocity normal to the surface.
Step 3: Calculate the final velocity components
After the collision:
- The vertical component remains v′y=v√2.
- The horizontal component is v′x=v2√2.
Step 4: Calculate the initial and final kinetic energies
The initial kinetic energy Ki is given by:
Ki=12mv2
The final kinetic energy Kf after the collision can be calculated using the new velocity components:
Kf=12m(v′2x+v′2y)=12m⎛⎝(v2√2)2+(v√2)2⎞⎠
Calculating each term:
v′2x=(v2√2)2=v28
v′2y=(v√2)2=v22
Thus,
Kf=12m(v28+v22)=12m(v28+4v28)=12m(5v28)=516mv2
Hint: Kinetic energy is a function of the square of velocity, so ensure you square the components correctly.
Step 5: Calculate the fraction of kinetic energy lost
The fraction of kinetic energy lost ΔK can be calculated as:
ΔK=Ki−Kf=12mv2−516mv2=(816−516)mv2=316mv2
The fraction of kinetic energy lost is:
Fraction lost=ΔKKi=316mv212mv2=3/168/16=38
Final Answer
The fraction of kinetic energy lost in the collision is 38.
---
The ball strikes the floor at an angle of θ=45∘ with the normal. We can denote the initial velocity of the ball as v. The components of the velocity can be calculated as follows:
- The vertical component (along the normal) is vy=vcos(45∘)=v√2.
- The horizontal component (parallel to the floor) is vx=vsin(45∘)=v√2.
The coefficient of restitution e is given as 12. The coefficient of restitution relates the velocities before and after the collision:
e=Velocity of separationVelocity of approach
The velocity of approach in the vertical direction is vy=v√2. After the collision, the vertical component of the velocity will be v′y (which remains the same in magnitude but changes direction). The horizontal component will change due to the collision, denoted as v′x.
e=v′xvy⟹12=v′xv√2
From this, we can solve for v′x:
v′x=12⋅v√2=v2√2
After the collision:
- The vertical component remains v′y=v√2.
- The horizontal component is v′x=v2√2.
The initial kinetic energy Ki is given by:
Ki=12mv2
The final kinetic energy Kf after the collision can be calculated using the new velocity components:
Kf=12m(v′2x+v′2y)=12m⎛⎝(v2√2)2+(v√2)2⎞⎠
Calculating each term:
v′2x=(v2√2)2=v28
v′2y=(v√2)2=v22
Thus,
Kf=12m(v28+v22)=12m(v28+4v28)=12m(5v28)=516mv2
The fraction of kinetic energy lost ΔK can be calculated as:
ΔK=Ki−Kf=12mv2−516mv2=(816−516)mv2=316mv2
The fraction of kinetic energy lost is:
Fraction lost=ΔKKi=316mv212mv2=3/168/16=38
The fraction of kinetic energy lost in the collision is 38.
Topper's Solved these Questions
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Only one option is correct for JEE Advanced
60 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - More a than one option is Correct
1 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Medical entrances gallery
27 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
Similar Questions
A ball stikes a horizontal floor at an angle theta = 30° with normal to the floor . The coefficient of restitution between the ball and floor is. e=13. The percentage of its kinetic energy lost in the collision is nearly
View SolutionA ball is dropped from a certain height on a horizontal floor. The coefficient of restitution between the ball and the floor is 12. The displacement time graph of the ball will be.
View SolutionA particle strikes a smooth horizontal surface at an angle of 45∘ with a velocity of 100m/s and rebounds. If the coefficient of restitution between the floor and the particle is 0.57 then the angle which the velocity of the particle after it rebounds will make with the floor is
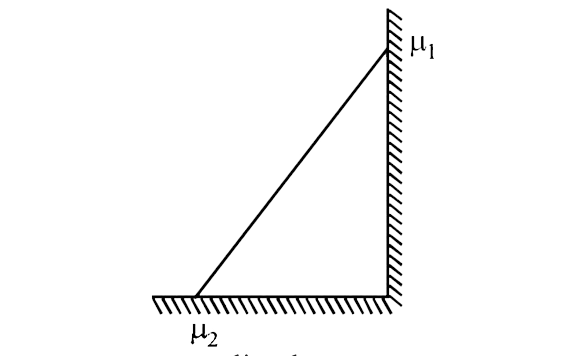
View SolutionIn the figure, a ladder of mass m is shown leaning against a wall. It is in static equilibrium making an angle θ with the horizontal floor. The coefficient of friction between the wall and the ladder is μ1 and that between the floor and the ladder is μ2. the normal reaction of the wall on the ladder is N1 and that of the floor is N2. if the ladder is about to slip. than
View SolutionA ball is dropped from a height h on a floor. The coefficient of restitution for the collision between the ball and the floor is e. The total distance covered by the ball before it comes to the rest.
View SolutionA ball rolls without sliding over a rough horizontal floor with velocity v0=7m/s towards a smooth vertical wall. If coefficient of restitution between the wall and the ball is e=0.7. Calculate velocity v of the ball after the collision.
View SolutionA particle of mass m strikes a horizontal smooth floor with velocity u making an angle θ with the floor and rebound with velocity v making an angle θ with the floor. The coefficient of restitution between the particle and the floor is e. Then
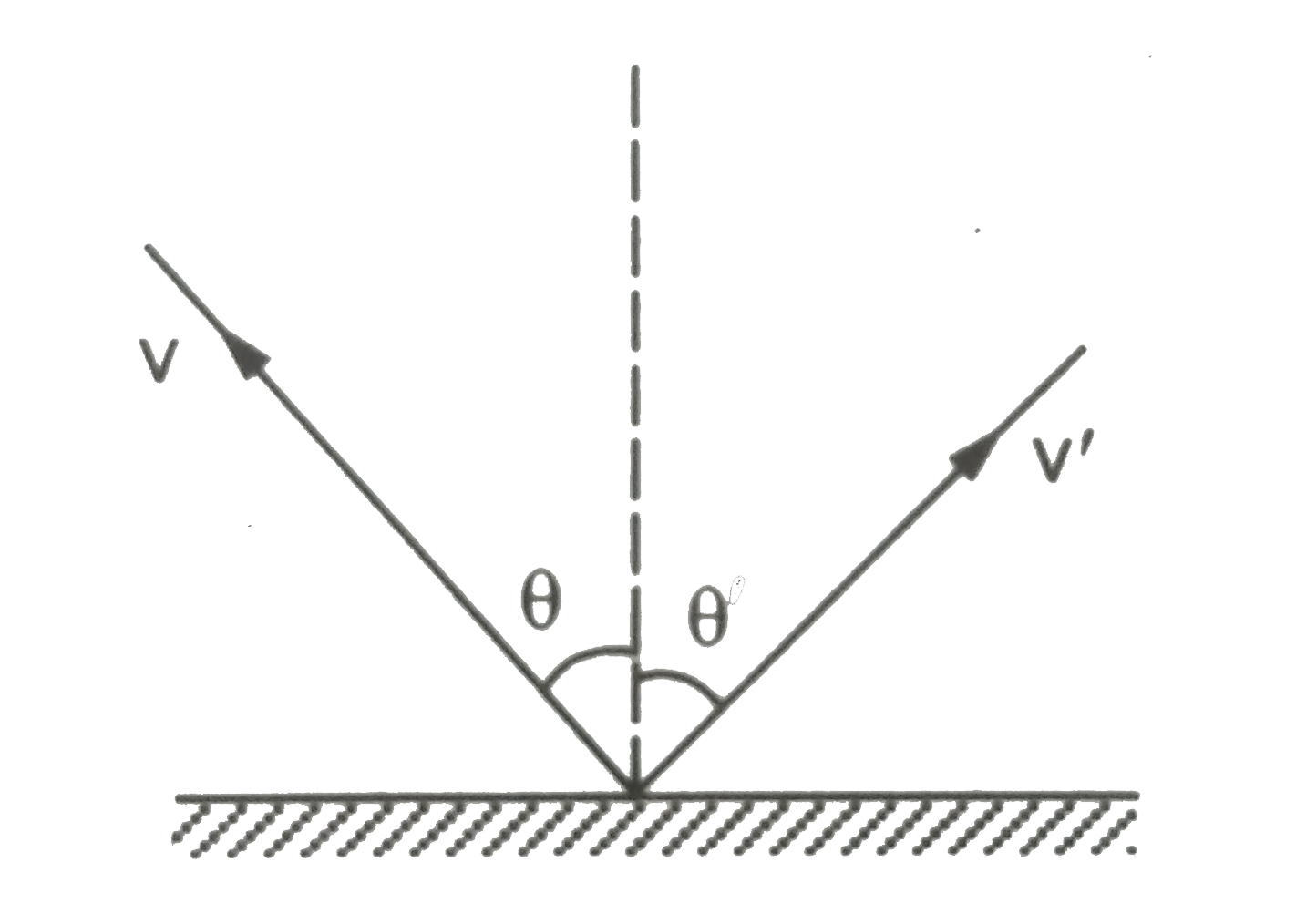
View SolutionA ball of a mass m hits the floor with as speed v making an angle of incidence θ with the normal. The coefficient of restitution is e. Find the speed of the reflected ball and the angle of reflection of the ball.
View SolutionA ball is dropped on a smooth inclined plane and is observed to move horizontally after the impact. The coefficient of restitution between the plane and ball is e. The inclination of the plane is
View SolutionA projectile is fired with a speed u at an angle θ above a horizontal field. The coefficient of restitution of collision between the projectile and the field is e. How far from the starting point does the projectile makes its second collision with the field?
View Solution
