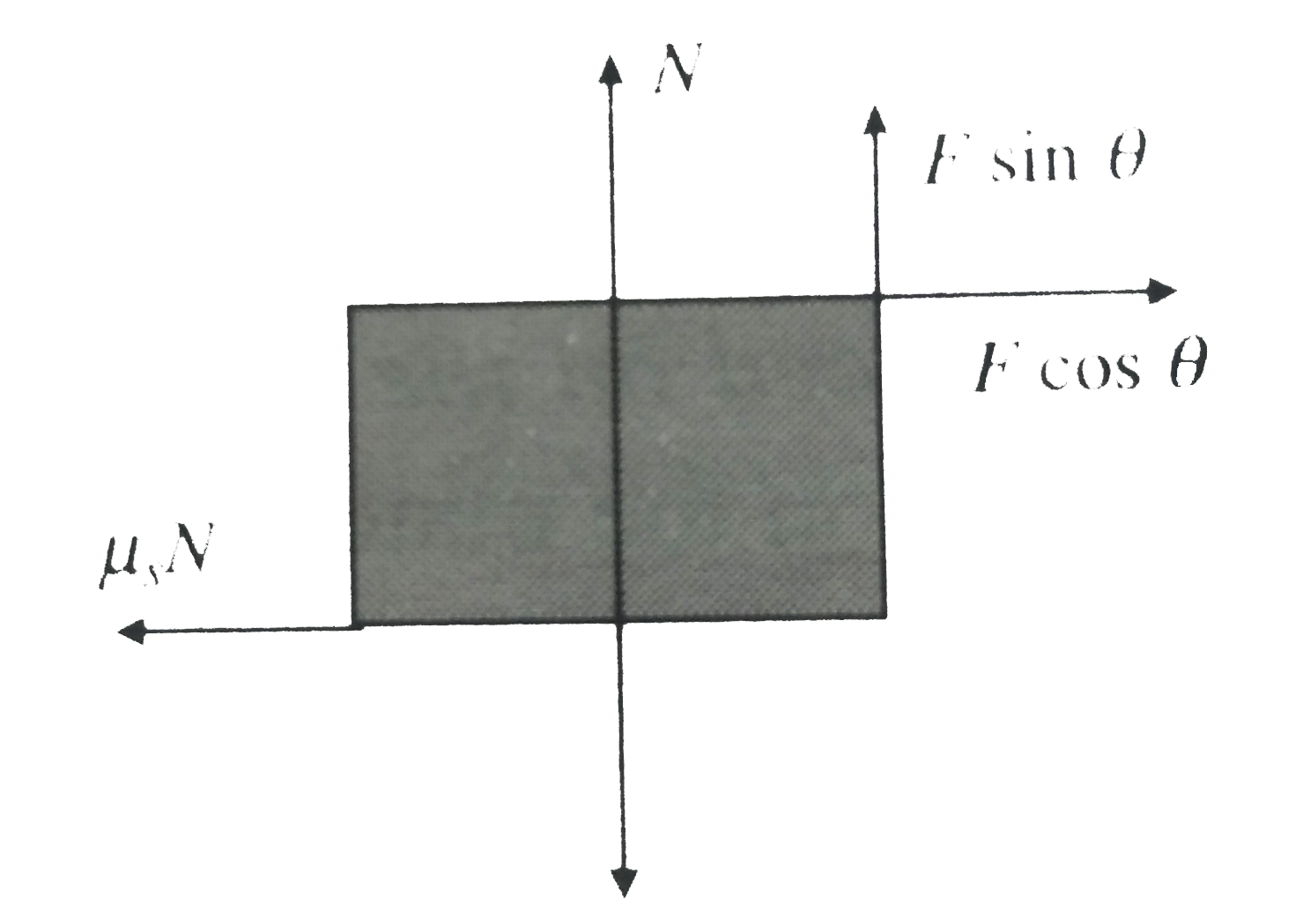
A block of mass m lying on a horizontal surface (coefficient of static friction =μs) is to be brought into motion by a pulling force F At what angle θ with the horizontal should the force F be applied so that its magnitude is minimum ? Also find the minimum magnitude

Let us first calculate the force F required to bring m into motion in terms of angle θ Equation using laws of motion are as follows:
N=mg−Fsinθ
andFcosθ=μ0N
Fcosθ=μ,(mg−Fsinθ)
⇒F=μsmgcosθ+μssinθ
 We have to find the angle θ For which this force F is minimum substituting μs=tanθ (for simplification), we get
We have to find the angle θ For which this force F is minimum substituting μs=tanθ (for simplification), we get
F=mgtanϕcosθ+tanϕsinθ=mgsinϕcos(θ−ϕ)
F is minimum is cos(θ−ϕ)maximum ,Hence F is minimum for θ=ϕ=tan−1μs, and Fmin=mgsinθ
To bring m into motion with least effect force should be applied at an angular tan−1μs and should have a magnitude equal to
Fmin=mgsinϕ=μsmg√1+μ2s
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Solved Examples
12 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.1
25 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A block of mass m is placed at rest on a horizontal rough surface with angle of friction ϕ. The block is pulled with a force F at an angle θ with the horizontal. The minimum value of F required to move the block is
View SolutionA block of mass m is placed at rest on a horizontal rough surface with angle of friction ϕ. The block is pulled with a force F at an angle θ with the horizontal. The minimum value of F required to move the block is
View SolutionA block of mass m is kept on a horizontal table. If the static friction coefficient is μ., find the frictional force acting on the block.
View SolutionA block of mass 1kg is at rest on a rough horizontal surface having coefficient of static friction 0.2 and kinetic friciton 0.15, find the frictional forces if a horizontal force,
(a) F=1N (b) F=1.96N (c) F=2.5N is applied on a block

View SolutionA block of mass m is moving on a rough horizontal surface. μ is the coefficient of kinetic friction between the block and the surface. What is the net force exerted by the surface on the block?
View SolutionA block of mass m is pulled on an incline surface having coefficient of friction μ=1& angle of inclination θ=30∘, with the horizontal, such that required external force is minimum. The angle made by this force with the incline is :
View SolutionA box of mass 200 kg is kept on a rough horizontal floor having coefficient of static friction μs=0.25 and coefficient kinetic friction μk=0.2. Find the work done by a man to slide the body slowly through 20 m with a minimum force. Also find the magnitude of the minimum force.
View SolutionA block of mass m is placed on a horizontla surface. If the block is pulled by applying a force of magnitude F=5mg at an angle θ=37∘, with horizontal as shown in fig. find the acceleration of the block at the given instant.

View SolutionTwo block mandM tied together with an inextensible string are placed at rest on a rought horizontal surface with coefficient of friction μ The block m is pulled with a variable force F at a varying angle θ with the horizontal .The value of θ at which the least value of F is required to move the blocks given by

View SolutionA block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
View Solution
