A car drives along a straight level frictionless road by an engine delivering constant power. Then velocity is directly proportional to
(a) √t
(b) 1√t
(c) √t
(d) None of the above
The correct Answer is:C
To solve the problem, we need to analyze the relationship between the velocity of the car and the time when the engine is delivering constant power.
1. Understanding Power:
The power P delivered by the engine is defined as the product of force F and velocity v:
P=F⋅v
2. Relating Force to Mass and Acceleration:
According to Newton's second law, the force can also be expressed as:
F=m⋅a
where m is the mass of the car and a is its acceleration.
3. Acceleration as a Function of Velocity:
Acceleration a can be defined as the rate of change of velocity:
a=dvdt
Substituting this into the force equation gives:
F=m⋅dvdt
4. Substituting Force in Power Equation:
Now, substituting F into the power equation:
P=m⋅dvdt⋅v
5. Rearranging the Equation:
Rearranging the equation gives:
P=m⋅v⋅dvdt
This can be rewritten as:
vdv=Pmdt
6. Integrating Both Sides:
Now we integrate both sides. The left side integrates with respect to v and the right side with respect to t:
∫vdv=∫Pmdt
This results in:
v22=Pmt+C
where C is the constant of integration.
7. Solving for Velocity:
Rearranging the equation to solve for v:
v2=2Pmt+2C
Taking the square root gives:
v=√2Pmt+2C
8. Identifying Proportionality:
If we consider the term √t in the equation, we can see that as t increases, v is directly proportional to √t.
Thus, we conclude that:
v∝t1/2
Final Answer:
The velocity v is directly proportional to the square root of time t when the engine delivers constant power.
The power P delivered by the engine is defined as the product of force F and velocity v:
P=F⋅v
According to Newton's second law, the force can also be expressed as:
F=m⋅a
where m is the mass of the car and a is its acceleration.
Acceleration a can be defined as the rate of change of velocity:
a=dvdt
Substituting this into the force equation gives:
F=m⋅dvdt
Now, substituting F into the power equation:
P=m⋅dvdt⋅v
Rearranging the equation gives:
P=m⋅v⋅dvdt
This can be rewritten as:
vdv=Pmdt
Now we integrate both sides. The left side integrates with respect to v and the right side with respect to t:
∫vdv=∫Pmdt
This results in:
v22=Pmt+C
where C is the constant of integration.
Rearranging the equation to solve for v:
v2=2Pmt+2C
Taking the square root gives:
v=√2Pmt+2C
If we consider the term √t in the equation, we can see that as t increases, v is directly proportional to √t.
v∝t1/2
The velocity v is directly proportional to the square root of time t when the engine delivers constant power.
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Multiple Correct
25 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Linked Comprehension
55 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Subjective
23 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moved from rest along a straight line by a machine delivering constant power. The ratio of displacement and velocity (s/v) varies with time t as
View SolutionA body is moved from rest along a straight line by a machine delivering constant power. The ratio of displacement and velocity (s/v) varies with time t as
View SolutionTwo cars A andB are racing along straight line. Car A is leading, such that their relative velocity is directly proportional to the distance between the two cars. When the lead of car A is l1=10m , its running 10m/s faster than car B. Determine the time car A will take to increase its lead to l2=20m from car B.
View SolutionTwo cars A andB are racing along straight line. Car A is leading, such that their relative velocity is directly proportional to the distance between the two cars. When the lead of car A is l1=10m , its running 10m/s faster than car B. Determine the time car A will take to increase its lead to l2=20m from car B.
View SolutionA body of mass 2 kg is driven by an engine delivering a constant power of 1 J /s. The body starts from rest and moves in a straight line. After 9 seconds, the body has moved a distance ( in m) ……….. .
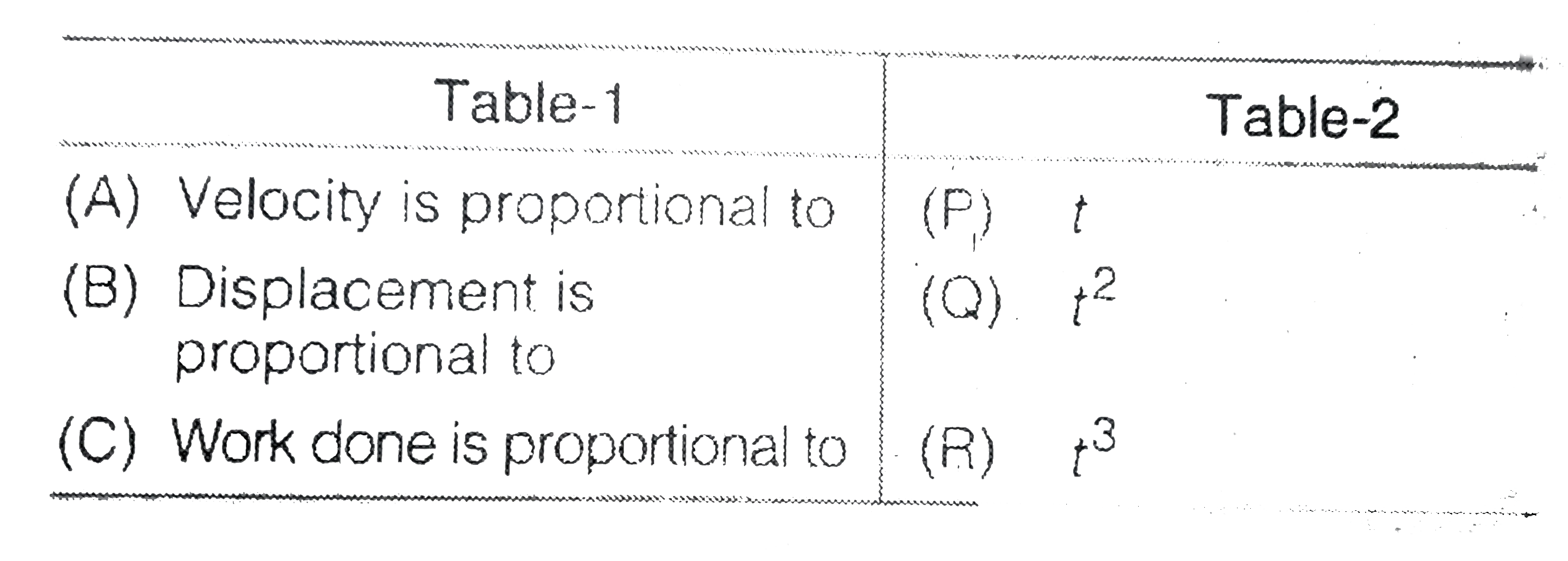
View SolutionA body is moved along a straight line by a machine delivering a power proportional to time (P∝t). Then, match the following.
View SolutionA constant force acts on a mass m at rest. Velocity acquired in travelling a fixed distance is directly proportional to :
View Solution
