A body is moved from rest along a straight line by a machine delivering constant power. The ratio of displacement and velocity (s/v) varies with time t as
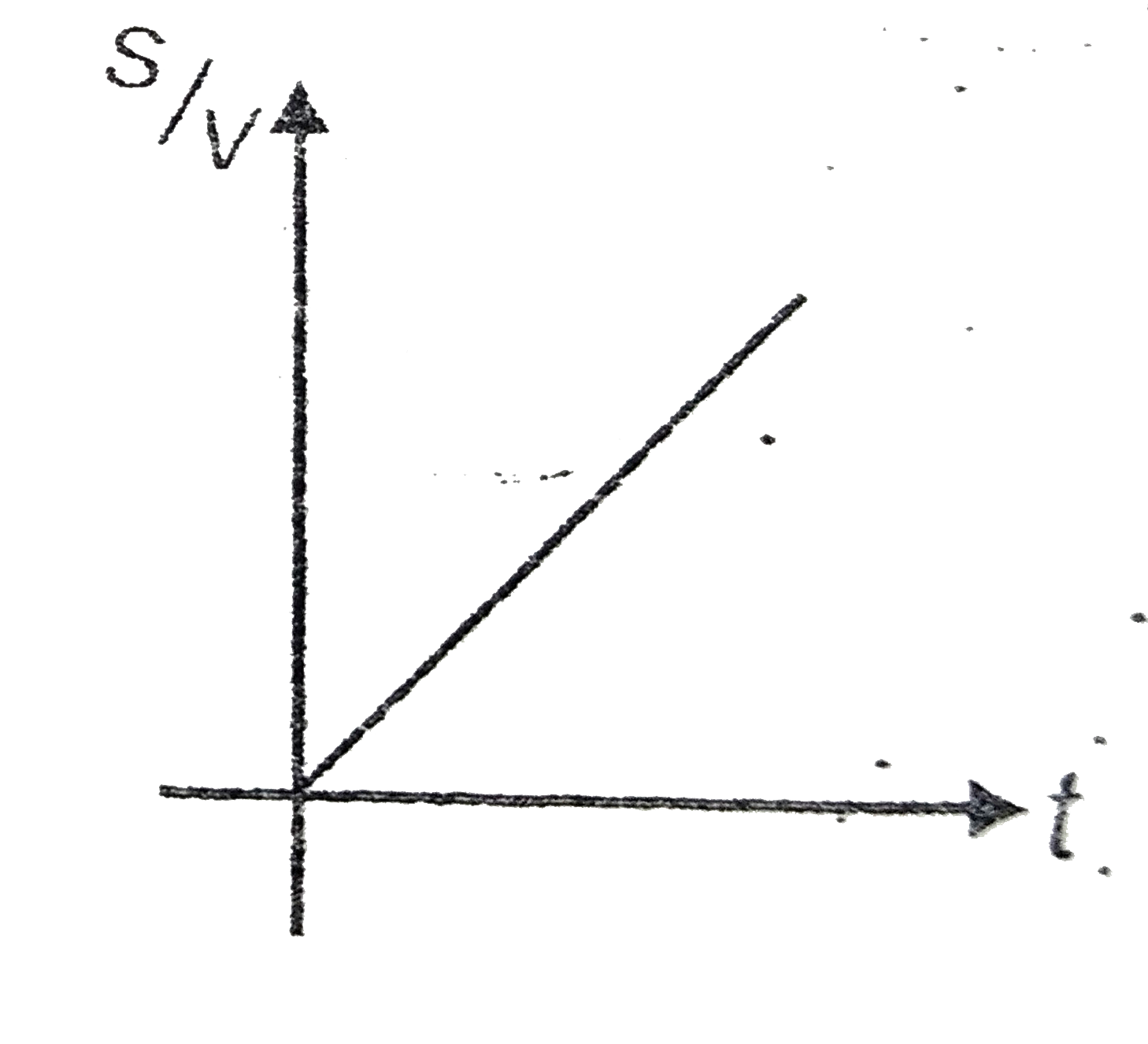
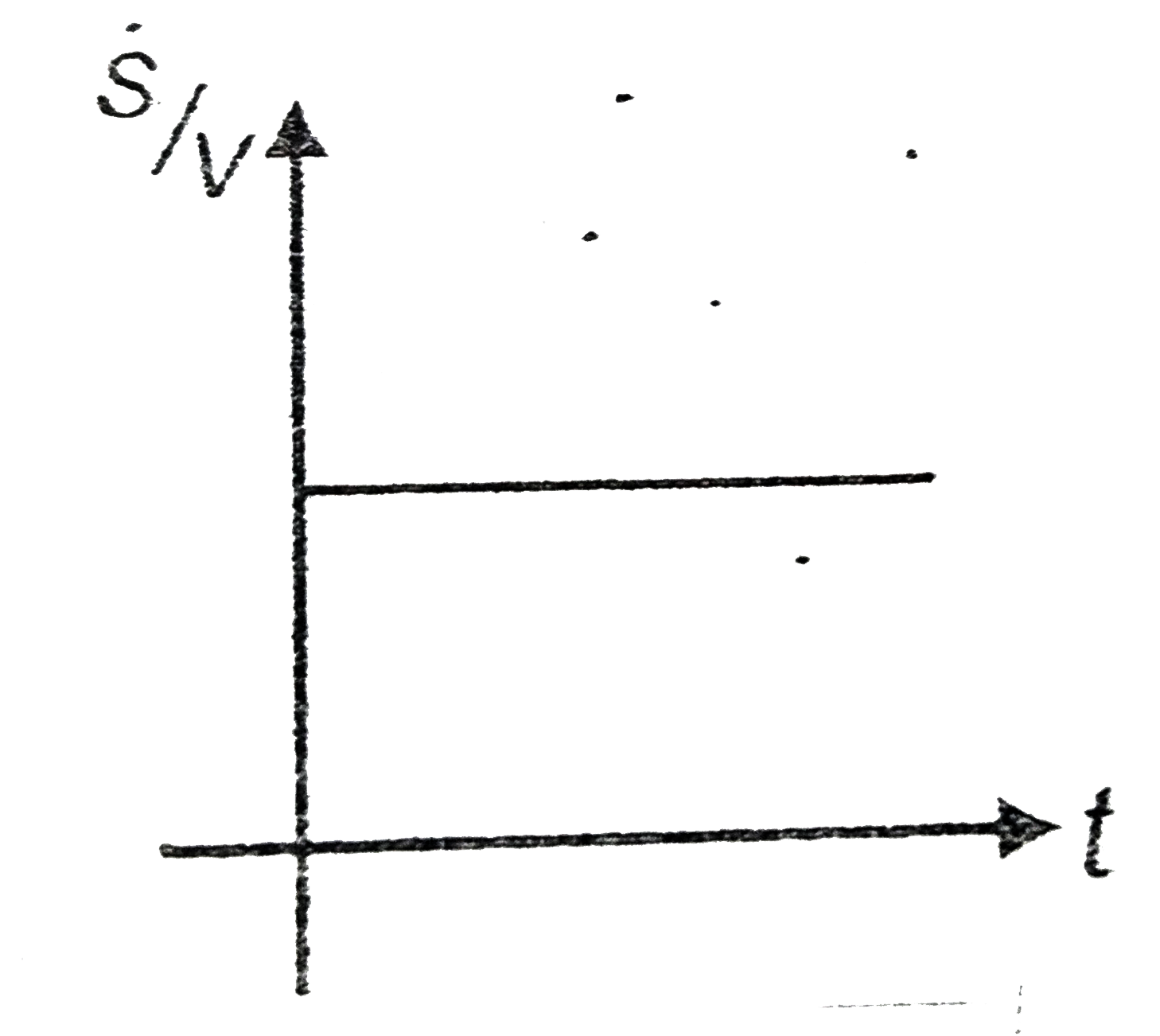
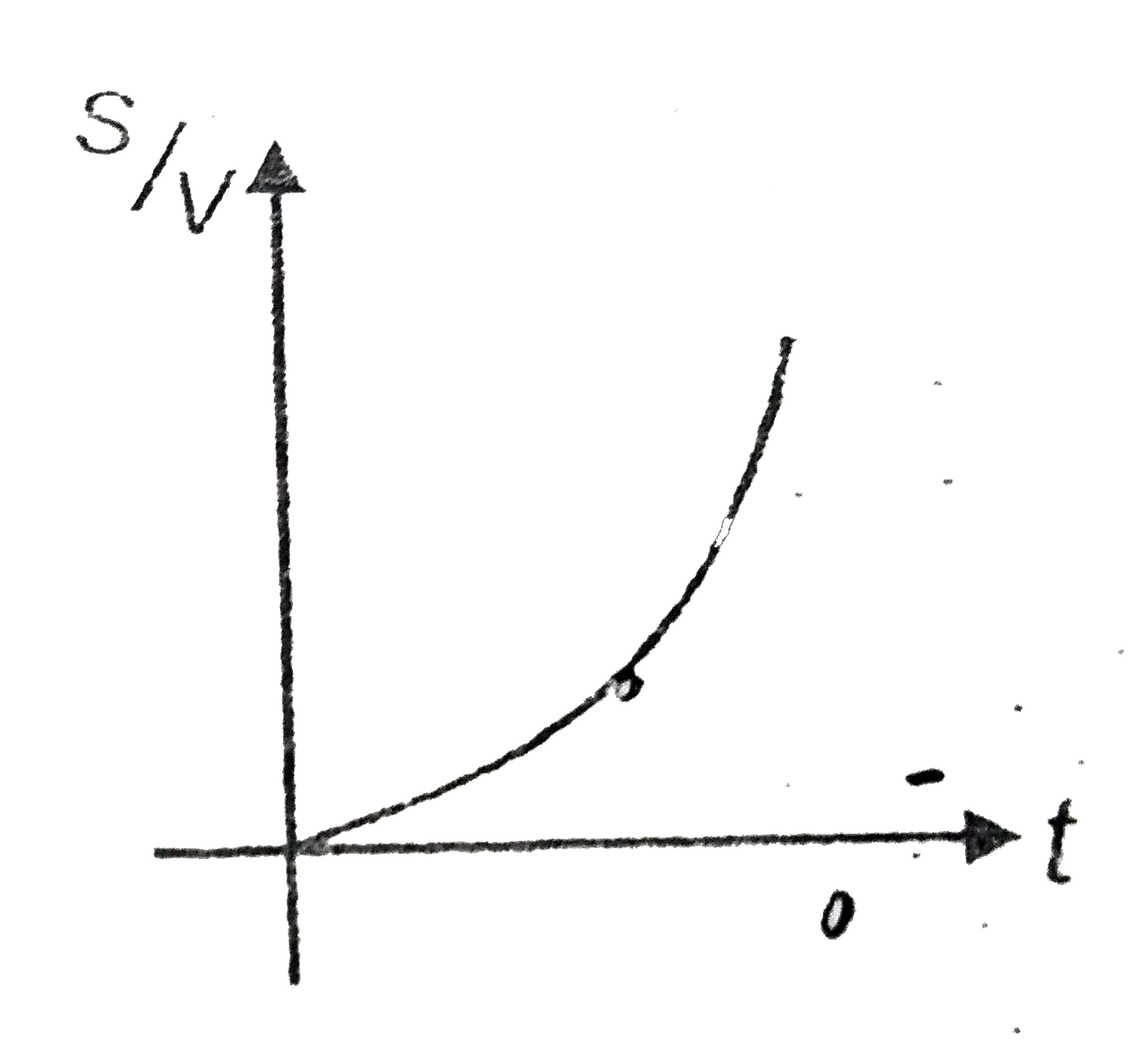
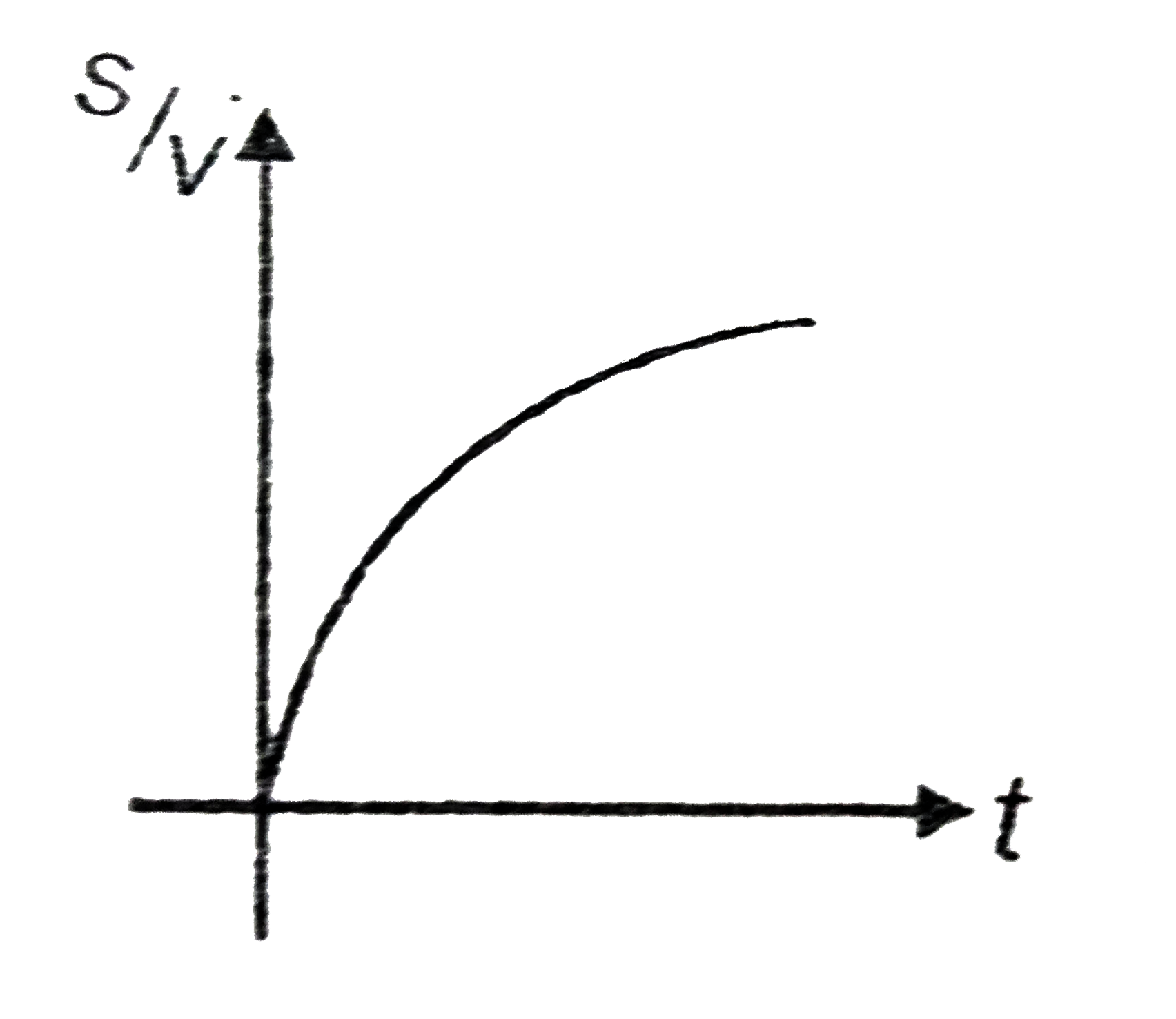
The correct Answer is:A
To solve the problem, we need to analyze the relationship between displacement (s), velocity (v), and time (t) when a body is moved from rest by a machine delivering constant power (P).
1. Understanding Power:
Power (P) is defined as the rate of doing work. Mathematically, it can be expressed as:
P=Wt
where W is the work done and t is time.
2. Work Done:
Since the machine delivers constant power, the work done over time t can be expressed as:
W=Pt
3. Kinetic Energy Relation:
The work done on the body is converted into kinetic energy. Hence, we can write:
W=12mv2
Setting the two expressions for work equal gives:
Pt=12mv2
4. Solving for Velocity:
Rearranging the equation to solve for v2:
v2=2Ptm
Taking the square root gives:
v=√2Pm⋅t1/2
Let k=√2Pm, then:
v=kt1/2
5. Finding Displacement:
The velocity v can also be expressed as the derivative of displacement s with respect to time t:
v=dsdt
Substituting our expression for v:
dsdt=kt1/2
Rearranging gives:
ds=kt1/2dt
6. Integrating to Find Displacement:
Integrating both sides to find s:
s=∫kt1/2dt
The integral of t1/2 is:
s=k⋅23t3/2+C
Since the body starts from rest, we can set the constant C=0:
s=2k3t3/2
7. Finding the Ratio sv:
Now, we need to find the ratio of displacement to velocity:
sv=2k3t3/2kt1/2
Simplifying gives:
sv=23t
8. Conclusion:
The ratio of displacement to velocity sv varies linearly with time t as:
sv=23t
Final Answer:
The ratio of displacement and velocity sv varies with time t as sv=23t.
Power (P) is defined as the rate of doing work. Mathematically, it can be expressed as:
P=Wt
where W is the work done and t is time.
Since the machine delivers constant power, the work done over time t can be expressed as:
W=Pt
The work done on the body is converted into kinetic energy. Hence, we can write:
W=12mv2
Setting the two expressions for work equal gives:
Pt=12mv2
Rearranging the equation to solve for v2:
v2=2Ptm
Taking the square root gives:
v=√2Pm⋅t1/2
Let k=√2Pm, then:
v=kt1/2
The velocity v can also be expressed as the derivative of displacement s with respect to time t:
v=dsdt
Substituting our expression for v:
dsdt=kt1/2
Rearranging gives:
ds=kt1/2dt
Integrating both sides to find s:
s=∫kt1/2dt
The integral of t1/2 is:
s=k⋅23t3/2+C
Since the body starts from rest, we can set the constant C=0:
s=2k3t3/2
Now, we need to find the ratio of displacement to velocity:
sv=2k3t3/2kt1/2
Simplifying gives:
sv=23t
The ratio of displacement to velocity sv varies linearly with time t as:
sv=23t
The ratio of displacement and velocity sv varies with time t as sv=23t.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-C (OBJECTIVE TYPE QUESTIONS) (MORE THAN ONE OPTIONS ARE CORRECT)
16 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-D (LINKED COMPREHENSION TYPE QUESTIONS) (COMPREHENSION-I)
3 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-A (OBJECTIVE TYPE QUESTIONS (ONE OPTIONISCORRECT)
60 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
A body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moved along a straight line by a machine delivering a power proportional to time (P∝t). Then, match the following.
View SolutionA car drives along a straight level frictionless road by an engine delivering constant power. Then velocity is directly proportional to
View SolutionA body starting from rest moves along a straight line with a constant acceleration. The variation of speed (v) with distance (s) is represented by the graph:
View SolutionA particle starting from rest moves along a straight line with constant acceleration for this velocity displacement graph will have the form-
View SolutionA particle of mass m is driven by a machine that delivers a constant power k watts. If the particle starts from rest the force on the particle at time t is
View SolutionA particle moves along a straight line its velocity dipends on time as v=4t−t2. Then for first 5s:
View SolutionA motor drives a body along a straight line with a constant force. The power P developed by the motor must vary with time t as
View Solution
