A body is moved along a straight line by a machine delivering a power proportional to time $(P \propto t)$ . Then, match the following.

Video Solution

Text Solution

Verified by Experts

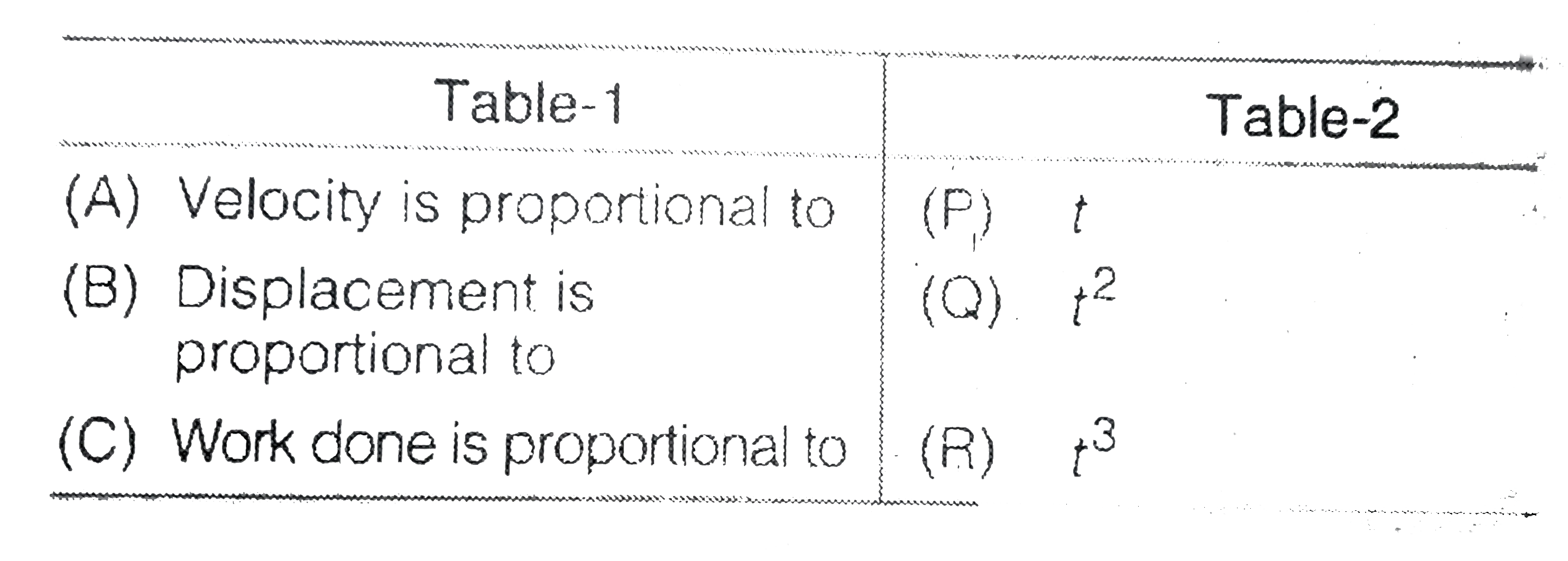
The correct Answer is: $(A \to p, B \to q, C \to q)$

$P \propto t$
$W = \int P d t = \int α t d t or W \propto t^{2}$
Since, work done is equal to change is KE
$Hence, v^{2} \propto t^{2} or v \propto t$ $W = \int P d t = \int α t d t or W \propto t^{2}$
Further, $v = \frac{d s}{d t}$
$∴ \frac{d s}{d t} \propto t or d s \propto t d t or s \propto t^{2} (by integration)$

Updated on:21/07/2023

|

Class 11 PHYSICS WORK, ENERGY AND POWER

Topper's Solved these Questions

Similar Questions

DC PANDEY ENGLISH-WORK, ENERGY AND POWER-MATCH THE COLUMNS

Home
Ask Doubt
Profile