A cylindrical block of length 0.4 m and area of cross-section 0.04m2 is placed coaxially on a thin metal disc of mass 0.4 kg and of the same cross - section. The upper face of the cylinder is maintained at a constant temperature of 400 K and the initial temperature of the disc is 300K. if the thermal conductivity of the material of the cylinder is 10watt/m−K and the specific heat of the material of the disc is 600J/kg−K, how long will it take for the temperature of the disc to increase to 350 K? Assume for purpose of calculation the thermal conductivity of the disc to be very high and the system to be thermally insulated except for the upper face of the cylinder.
The correct Answer is:A
To solve the problem, we need to determine how long it will take for the temperature of the metal disc to increase from 300 K to 350 K when it is in contact with a cylindrical block maintained at 400 K. We will use the principles of heat conduction and specific heat capacity.
1. Identify Given Values:
- Length of the cylinder, L=0.4m
- Cross-sectional area, A=0.04m2
- Mass of the disc, m=0.4kg
- Initial temperature of the disc, Ti=300K
- Final temperature of the disc, Tf=350K
- Constant temperature of the cylinder, Tc=400K
- Thermal conductivity of the cylinder, k=10W/m-K
- Specific heat capacity of the disc, c=600J/kg-K
2. Calculate Heat Transfer Rate (dQ/dt):
The heat transfer rate through conduction can be expressed as:
dQdt=k⋅AL(Tc−T)
Substituting the values:
dQdt=10⋅0.040.4(400−T)=1(400−T)
Thus, we have:
dQdt=400−T(Equation 1)
3. Relate Heat Transfer to Temperature Change of the Disc:
The heat gained by the disc can be expressed as:
dQdt=m⋅c⋅dTdt
Substituting the values:
dQdt=0.4⋅600⋅dTdt=240dTdt(Equation 2)
4. Set Equations Equal:
From Equations 1 and 2, we have:
400−T=240dTdt
5. Rearrange and Separate Variables:
Rearranging gives:
dT400−T=dt240
6. Integrate Both Sides:
Integrating both sides, we have:
∫350300dT400−T=∫T0dt240
The left side integrates to:
−ln(400−T)∣∣350300=−ln(400−350)+ln(400−300)
This simplifies to:
−ln(50)+ln(100)=ln(10050)=ln(2)
The right side integrates to:
T240
7. Equate and Solve for Time (T):
Thus, we have:
ln(2)=T240
Solving for T:
T=240ln(2)
8. Calculate T:
Using ln(2)≈0.693:
T≈240×0.693≈166.32seconds
Final Answer:
It will take approximately 166.32 seconds for the temperature of the disc to increase from 300 K to 350 K.
- Length of the cylinder, L=0.4m
- Cross-sectional area, A=0.04m2
- Mass of the disc, m=0.4kg
- Initial temperature of the disc, Ti=300K
- Final temperature of the disc, Tf=350K
- Constant temperature of the cylinder, Tc=400K
- Thermal conductivity of the cylinder, k=10W/m-K
- Specific heat capacity of the disc, c=600J/kg-K
The heat transfer rate through conduction can be expressed as:
dQdt=k⋅AL(Tc−T)
Substituting the values:
dQdt=10⋅0.040.4(400−T)=1(400−T)
Thus, we have:
dQdt=400−T(Equation 1)
The heat gained by the disc can be expressed as:
dQdt=m⋅c⋅dTdt
Substituting the values:
dQdt=0.4⋅600⋅dTdt=240dTdt(Equation 2)
From Equations 1 and 2, we have:
400−T=240dTdt
Rearranging gives:
dT400−T=dt240
Integrating both sides, we have:
∫350300dT400−T=∫T0dt240
The left side integrates to:
−ln(400−T)∣∣350300=−ln(400−350)+ln(400−300)
This simplifies to:
−ln(50)+ln(100)=ln(10050)=ln(2)
T240
Thus, we have:
ln(2)=T240
Solving for T:
T=240ln(2)
Using ln(2)≈0.693:
T≈240×0.693≈166.32seconds
It will take approximately 166.32 seconds for the temperature of the disc to increase from 300 K to 350 K.
Topper's Solved these Questions
- CALORIMETRY & HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY & HEAT TRANSFEREXERCISE - Level 2 More Than One Correct
5 Videos
- BASIC MATHEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise
13 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery
38 Videos
Similar Questions
A cylindrical conductor of length l and uniform area of cross-section A has resistance R. Another conductor of length 2l and resistance R of the same material has area of cross-section :
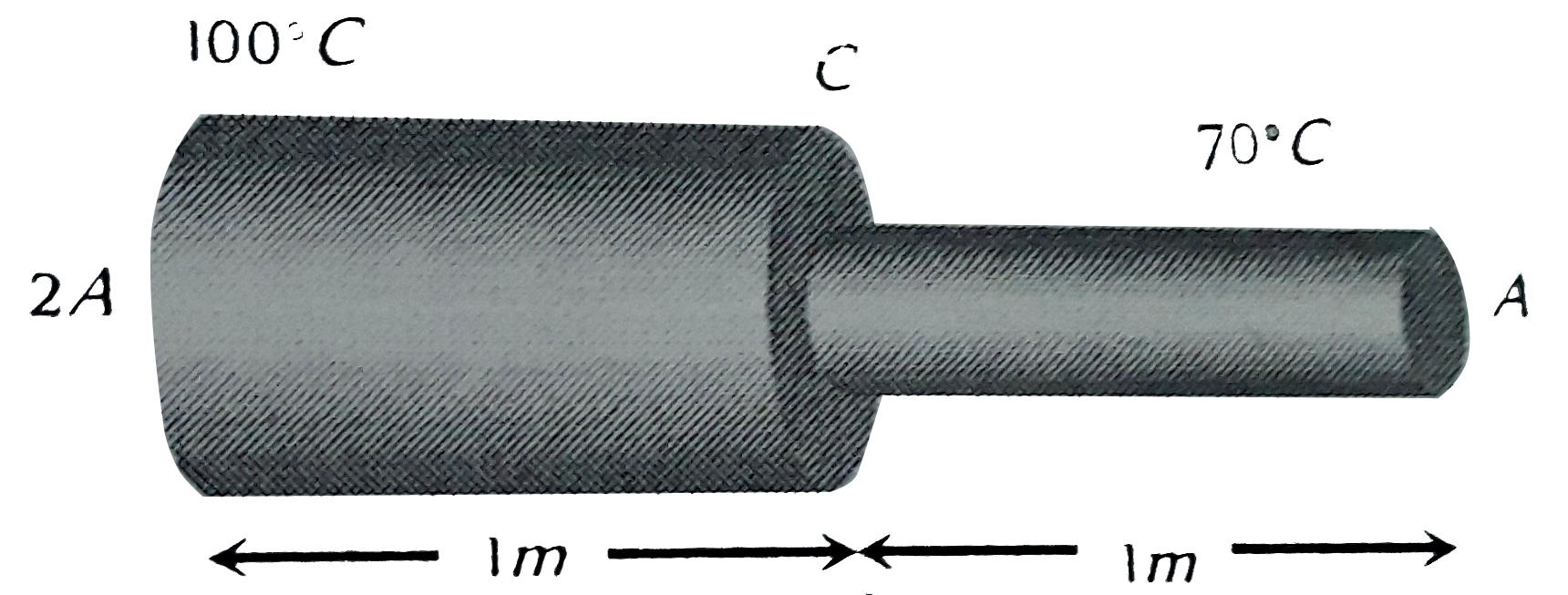
View SolutionA metal rod of length 2 m has cross sectional areas 2 A and A as shown in figure. The ends are maintained at temperatures 100° C and 70° C . The temperature at middle point C is
View SolutionA black body with surface area 0.001 m2 is heated upto a temperature 400 K and is suspended in a room temperature 300K. The intitial rate of loss of heat from the body to room is
View SolutionA uniform cylinder of length L and thermal conductivity k is placed on a metal plate of the same area S of mass m and infinite conductivity. The specific heat of the plate is c. The top of the cylinder is maintained at T0. Find the time required for the temperature of the plate to rise from T1 to T2(T1<T2<T0).
View SolutionA silver cylindrical rod has a length of 0.5 m and radius of 0.4 m, find the density of the rod, if its mass is 2640 kg.
View SolutionA cylinder of radius R is surrounded by a cylindrical shell of inner radius R and oputer radius 2R. The thermal conductivity of the material of the inner cylinder is k1 and that of the outer cylinder is K2 ,Assumming no loss of heat , the effective thermal conductivity of the system for heat flowing along the length of the cylinder is :
View SolutionA copper rod of length 75 cm and an iron rod of length 125cm are joined together end to end . Both are of circular cross section with diameter 2 cm . The free ends of the copper and iron are maintained at 100∘C and0∘C respectively . The surface of the bars are insulated thermally . The temperature of the copper -iron junction is [Thermal conductivity of the copper is 386.4W/m−K and that of iron is 48.46W/m−K].
View SolutionThree rods each of same length and cross - section are joined in series. The thermal conductivity of the materials are K, 2K and 3K respectively. If one end is kept at 200∘C and the other at 100∘C. What would be the temperature of the junctions in the steady state? Assume that no heat is lost due to radiation from the sides of the rods.
View SolutionThe ends of a copper rod of length 1m and area of cross-section 1cm2 are maintained at 0∘C and 100∘C. At the centre of the rod there is a source of heat of power 25 W. Calculate the temperature gradient in the two halves of the rod in steady state. Thermal conductivity of copper is 400Wm−1K−1.
View SolutionThe ends of a copper rod of length 1m and area of cross-section 1cm2 are maintained at 0∘C and 100∘C. At the centre of the rod there is a source of heat of power 25 W. Calculate the temperature gradient in the two halves of the rod in steady state. Thermal conductivity of copper is 400Wm−1K−1.
View Solution
