A horizontal disc rotates freely with angular velocity ω about a vertical axes through its centre. A ring having the same mass and radius as the disc, is now gently placed coaxially on the disc. After some time, the two rotate with a common angular velocity. then.
no friction exists between te disc and the ring
the angular momentum of the system is conserved
the final common angular velocity is 12ω
all of the above
To solve the problem step by step, we will analyze the situation involving a rotating disc and a ring placed on it.
Step 1: Understand the Initial Conditions
- A horizontal disc is rotating with an angular velocity ω about a vertical axis through its center.
- A ring, having the same mass m and radius r as the disc, is gently placed coaxially on the disc.
Hint: Identify the initial angular momentum of the system before the ring is placed.
Step 2: Determine the Initial Angular Momentum
- The moment of inertia of the disc Idisc is given by:
Idisc=12mr2
- The initial angular momentum Linitial of the disc is:
Linitial=Idisc⋅ω=12mr2⋅ω
Hint: Remember that angular momentum is conserved when no external torque acts on the system.
Step 3: Analyze the System After the Ring is Placed
- When the ring is placed on the disc, it will eventually rotate with the disc at a common angular velocity ω′.
- The total moment of inertia of the system after the ring is placed is:
Itotal=Idisc+Iring=12mr2+mr2=32mr2
Hint: Use the principle of conservation of angular momentum to relate the initial and final states.
Step 4: Apply Conservation of Angular Momentum
- According to the conservation of angular momentum:
Linitial=Lfinal
- The final angular momentum Lfinal can be expressed as:
Lfinal=Itotal⋅ω′=32mr2⋅ω′
- Setting the initial and final angular momentum equal gives:
12mr2⋅ω=32mr2⋅ω′
Hint: Simplify the equation by canceling common terms.
Step 5: Solve for the Final Angular Velocity
- Canceling mr2 from both sides:
12ω=32ω′
- Rearranging gives:
ω′=13ω
Hint: Check if the final angular velocity matches any of the options provided in the question.
Step 6: Evaluate the Statements
1. No friction exists between the disc and the ring: This is false because friction is necessary for the ring to gain angular velocity.
2. The angular momentum of the system is conserved: This is true since no external torque acts on the system.
3. The final common angular velocity is half ω: This is false; we found it to be 13ω.
Conclusion
- The only correct statement is that the angular momentum of the system is conserved.
Final Answer: The correct statement is: "The angular momentum of the system is conserved."
- A horizontal disc is rotating with an angular velocity ω about a vertical axis through its center.
- A ring, having the same mass m and radius r as the disc, is gently placed coaxially on the disc.
- The moment of inertia of the disc Idisc is given by:
Idisc=12mr2
- The initial angular momentum Linitial of the disc is:
Linitial=Idisc⋅ω=12mr2⋅ω
- When the ring is placed on the disc, it will eventually rotate with the disc at a common angular velocity ω′.
- The total moment of inertia of the system after the ring is placed is:
Itotal=Idisc+Iring=12mr2+mr2=32mr2
- According to the conservation of angular momentum:
Linitial=Lfinal
- The final angular momentum Lfinal can be expressed as:
Lfinal=Itotal⋅ω′=32mr2⋅ω′
- Setting the initial and final angular momentum equal gives:
12mr2⋅ω=32mr2⋅ω′
- Canceling mr2 from both sides:
12ω=32ω′
- Rearranging gives:
ω′=13ω
1. No friction exists between the disc and the ring: This is false because friction is necessary for the ring to gain angular velocity.
2. The angular momentum of the system is conserved: This is true since no external torque acts on the system.
3. The final common angular velocity is half ω: This is false; we found it to be 13ω.
- The only correct statement is that the angular momentum of the system is conserved.
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 1 Subjective
32 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 2 Single Correct
26 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Assertion And Reason
11 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
Similar Questions
A disc is rotating with angular velocity ω. If a child sits on it, what is conserved?
View SolutionA uniform disc of radius a and mass m, is rotating freely with angular speed ω in a horizontal plane about a smooth fixed vertical axis through its centre. A particle, also of mass m, is suddenly attached to the rim of the disc of the disc and rotates with it. The new angular speed is
View Solution
Knowledge Check
When a disc rotates with uniform angular velocity, which of the following is not true ?
Aa. The sense of rotation remains same.Bb. The orientation of the axis of rotation remains same.Cc. The speed of rotation is non-zero and remains same.Dd. The angular acceleration is non-zero and remains same.
A horizontal disc is rotating about a vertical axis passing through its centre. If an insect moves from centre to rim then the angular momentum of the system
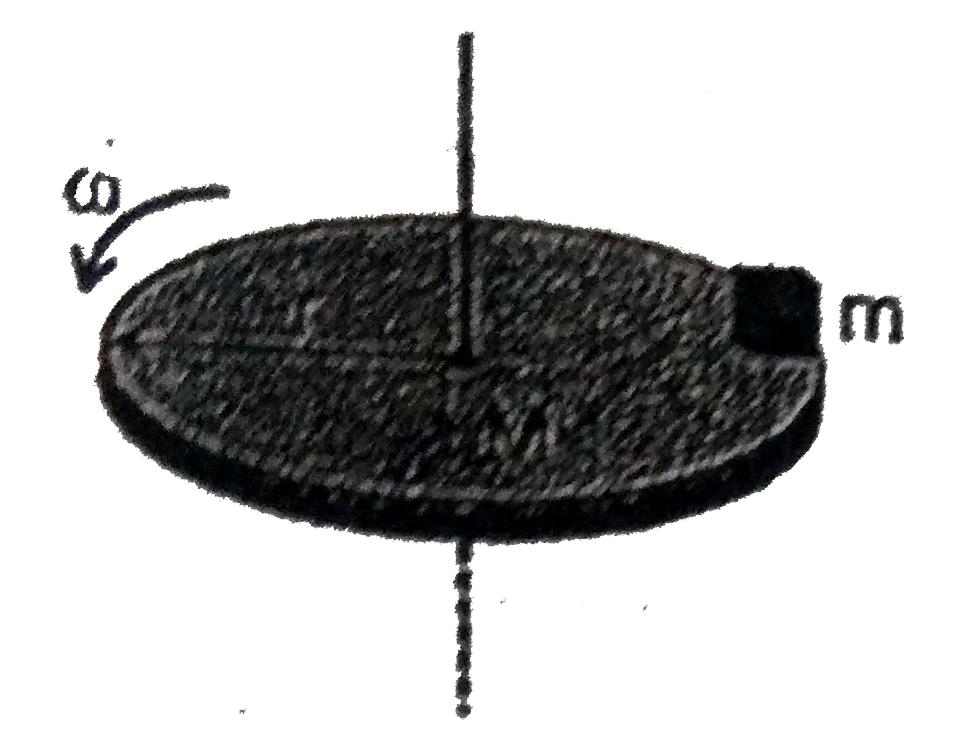
View SolutionA smoothh disc is rotating with uniform angular speed ω about a fixed vertical axis passing through its centre and normal to its plane as shown. A small block of mass m is gently placed at the periphery of the disc. Then (pickup the correct alternative or alternatives)
View SolutionA disc has mass 'M" and radius 'R'. How much tangential force should be applied to the rim of the disc so as to rotate with angular velocity ω in time 't'?
View SolutionWhen a disc rotates with uniform angular velocity, which of the following is not true ?
View SolutionTwo discs A and B are mounted coaxiallay on a vertical axle. The discs have moments of inertia I and 2 I respectively about the common axis. Disc A is imparted an initial angular velocity 2ω using the entire potential energy of a spring compressed by a distance x1 Disc B is imparted an angular velocity ω by a spring having the same spring constant and compressed by a distance x2 Both the discs rotate in the clockwise direction.
When disc B is brought in contact with disc A, they acquire a common angular velocity in time t. The average frictional torque on one disc by the other during this period is
View SolutionA metal disc of radius a rotates with a constant angular velocity ω about its axis. The potential difference between the center and the rim of the disc is (m = mass of electron, e = charge on electro)
View SolutionA uniform circular disc of mass 50kg and radius 0.4 m is rotating with an angular velocity of 10rads−1 about its own axis, which is vertical. Two uniform circular rings, each of mass 6.25 kg and radius 0.2 m, are gently placed symmetrically on the disc in such a manner that they are touching each other along the axis of the disc and are horizontal. Assume that the friction is large enough such that the rings are at rest relative to the disc and the system rotates about the original axis. The new angular velocity (∈reds−1) of the system is
View Solution
