A line charge λ per unit length is lodged uniformly onto the rim of a wheel of mass M and radius R. The wheel has light non-conducting spokes and is free to rotate without friction about its axis (Fig. 6.22). A uniform magnetic field extends over a circular region within the rim. It is given by,
B=B0k (ra≤a,a<R)
=0 (otherwise)
What is the angular velocity of the wheel after the field is suddenly switched off?

Line charge per unit length =λ=Total charg eLeng th=Q2πr
Where,
r, Distance of the point within the wheel
Mass of the wheel = M
Radius of the wheel = R
Magnetic field, ¯¯¯B=−B0ˆk
At distance r,themagnetic force is balanced by the centripetal force i.e.,
BQv=Mv2r
Where,
v= linear velocity of the wheel
∴B2πrλ=Mvr
v=B2πλr2M
∴ Angular velocity , ω=vR=B2πλr2MR
For r≤ and a<R, we get
ω=−2B0a2λMRˆk
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise
17 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Exercise
34 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise
15 Videos
Similar Questions
A line charge λ per unit length is pasted uniformly on to the wire of a wheel of mass m and radius R. The wheel has light non-conducting spokes and is free to rotate about a vertical axis as shown in Fig.3.166. A uniform magnetic field extends over a radial rigion of radius r given by B=−B0ˆk(r≤a,a<R)=0 (otherwise). What is the angular velocity of the wheel this field is suddenly switched off?
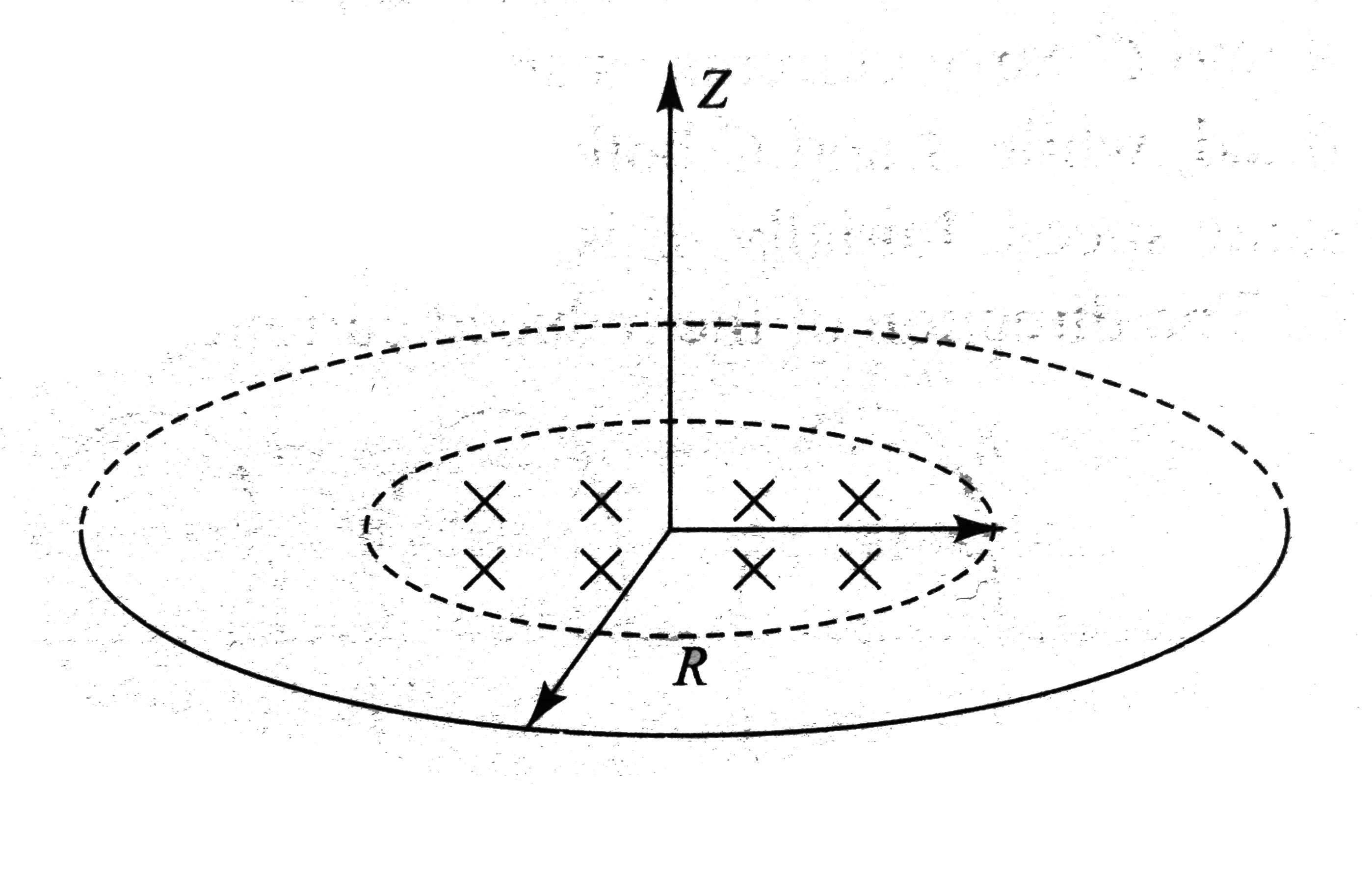
View SolutionThe moment of inertia of a disc of mass M and radius R about a tangent to its rim in its plane is
View SolutionA wheel comprises a ring of radius R and mass M and three spokes of mass m each. The moment of inertia of the wheel about its axis is

View SolutionA dust particle lying at the rim of a wheel has an angular speed of 22 rad/s. Find the time period.
View SolutionA conducting disc of radius r rotaes with a small but constant angular velocity ω about its axis. A uniform magnetic field B exists parallel to the axis of rotation. Find the motional emf between the centre and the periphery of the disc.
View SolutionThe spokes of a wheel are made of metal and their lengths are of one metre. On rotating the wheel about its own axis in a uniform magnetic field of 5×10−5 tesla normal to the plane of the wheel, a potential difference of 3.14mV is generated between the rim and the axis. The rotational velocity of the wheel is-
View SolutionA string is wrapped around the rim of a wheel of moment of inertia 0.20 kg-m^2 and radius 20 cm. The wheel is free to rotate about it axis. Initially, the wheel is at rest. The string is now pulled by a force of 20 N. Find the angular velocity of the wheel after 5.0 seconds.
View SolutionA plastic disc of radius 'R' has a charge 'q ' uniformly distributed over its surface. If the disc is rotated with a frequency 'f' about its axis, then the magnetic induction at the centre of the disc is given by
View SolutionA point on the rim of a wheel 3 m in diameter has linear velocity of 18 ms−1 . The angular velocity of the wheel is
View SolutionA uniformly charged solid sphere a mass M, radius R having charge Q is rotated about its diameter with frequency f0. The magnetic moment of the sphere is
View Solution
