A dust particle lying at the rim of a wheel has an angular speed of 22 rad/s. Find the time period.
The correct Answer is:27s
To solve the problem of finding the time period of a dust particle lying at the rim of a wheel with an angular speed of 22 rad/s, we can follow these steps:
1. Understand the Relationship: The time period T of an object in circular motion is related to its angular speed ω by the formula:
T=2πω
2. Identify Given Values: From the problem, we know:
- Angular speed ω=22rad/s
3. Substitute the Value into the Formula: Plug the value of angular speed into the formula for time period:
T=2π22
4. Calculate 2π: We can use the approximation π≈227:
2π≈2×227=447
5. Calculate the Time Period: Now substitute this back into the equation for T:
T=44722
Simplifying this gives:
T=447×22=44154=27seconds
6. Final Answer: Therefore, the time period T is:
T=27seconds
Summary:
The time period of the dust particle is 27 seconds.
---
T=2πω
- Angular speed ω=22rad/s
T=2π22
2π≈2×227=447
T=44722
Simplifying this gives:
T=447×22=44154=27seconds
T=27seconds
The time period of the dust particle is 27 seconds.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -A Objective (one option is correct)
50 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Assignement section -B Objective (one option is correct)
29 Videos
- MOTION IN A PLANEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - llustration 1 :
1 Videos
- MOCK_TEST_17BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOCK_TEST_17EXERCISE - Example
15 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - D)
15 Videos
Similar Questions
A disc of radius 10 cm is rotating about its axis at an angular speed of 20 rad/s. Find the linear speed of
a. a point on the rim,
b. the middle point of the radius.
View SolutionA particle is revolving in a circle of radius 1 m with an angular speed of 12 rad/s. At t = 0, it was subjected to a constant angular acceleration α and its angular speed increased to (480π) rotation per minute (rpm) in 2 sec. Particle then continues to move with attained speed. Calculate
(i) angular acceleration of the particle,
(ii) tangential velocity of the particle as a function of time.
(iii) acceleration of the particle at t = 0.5 second and at t = 3 second
(iv) angular displacement at t = 3 second.
View SolutionA simple pendulum of length 40 cm oscillates with an angular amplitude of 0.04 rad. Find a. the time period b. the linear amplitude of the bob, c. The speed of the bob when the string makes 0.02 rad with the vertical and d. the angular acceleration when the bob is in momentarily rest. Take g=10ms−2.
View SolutionA particle is moving in a circle of radius 4cm with constant speed of 1cm/s. Find
(a) time period of the particle.
(b) average speed, average velocity and average acceleration in a time interval from t=0 to t=T4. Here, T is the time period of the particle. Give only their magnitudes.
View SolutionTwo particles AandB move on a circle Initially, particles AandB are diagonally opposite to each other. Particle A moves with angular velocity πrads−1 and angular acceleration π/2rads−2 and particle B moves with constant angular velocity 2πrads−2. Find the time after which both the particlsd AnadB will collide.
View SolutionIn figure wheel A of radius rA=10cm is coupled by belt B to wheel C of radius rC=25cm. The angular speed of wheel A is increased from rest at a constant rate of 1.6rad/s2. Find the time needed for wheel C to reach an angular speed of 12.8rad/s, assuming the belt does not slip.
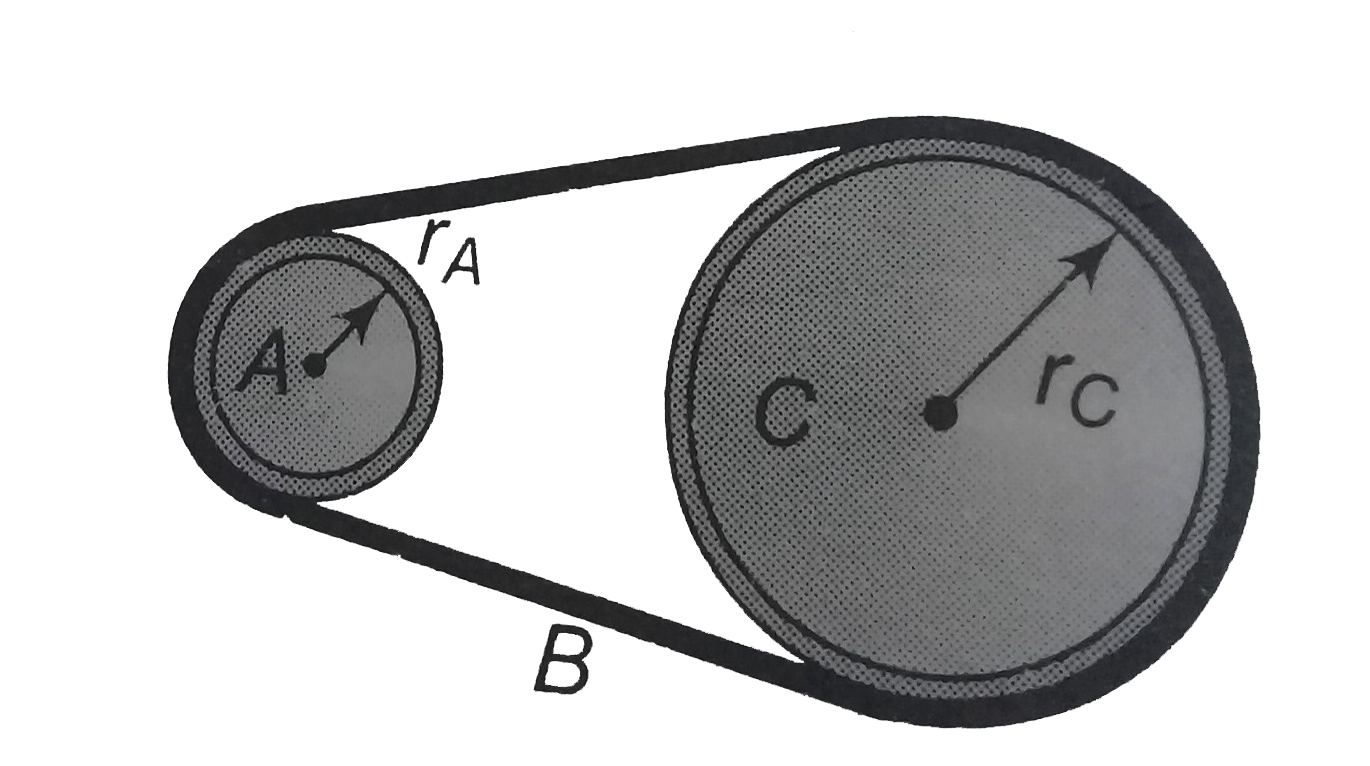 .
.
View SolutionA particle is revolving in a circular path of radius 2 m with constant angular speed 4 rad/s. The angular acceleration of particle is
View SolutionAngular speed of a uniformly circulating body with time period T is
View SolutionA wheel has angular acceleration of 3.0rad/s2 and an initial angular speed of 2.00rad/s. In a time of 2s it has rotated through an angle (in radian) of
View SolutionA wheel has angular acceleration of 3.0rad/s2 and an initial angular speed of 2.00rad/s. In a time of 2s it has rotated through an angle (in radian) of
View Solution
