A loop of mass M with two identical rings of mass m at its top hangs from a ceiling by an inextensible string. If the rings gently pushed horizontally in opposite directions, find the angular distance covered by each ring when the tension in the string vanishes for once during their motion.
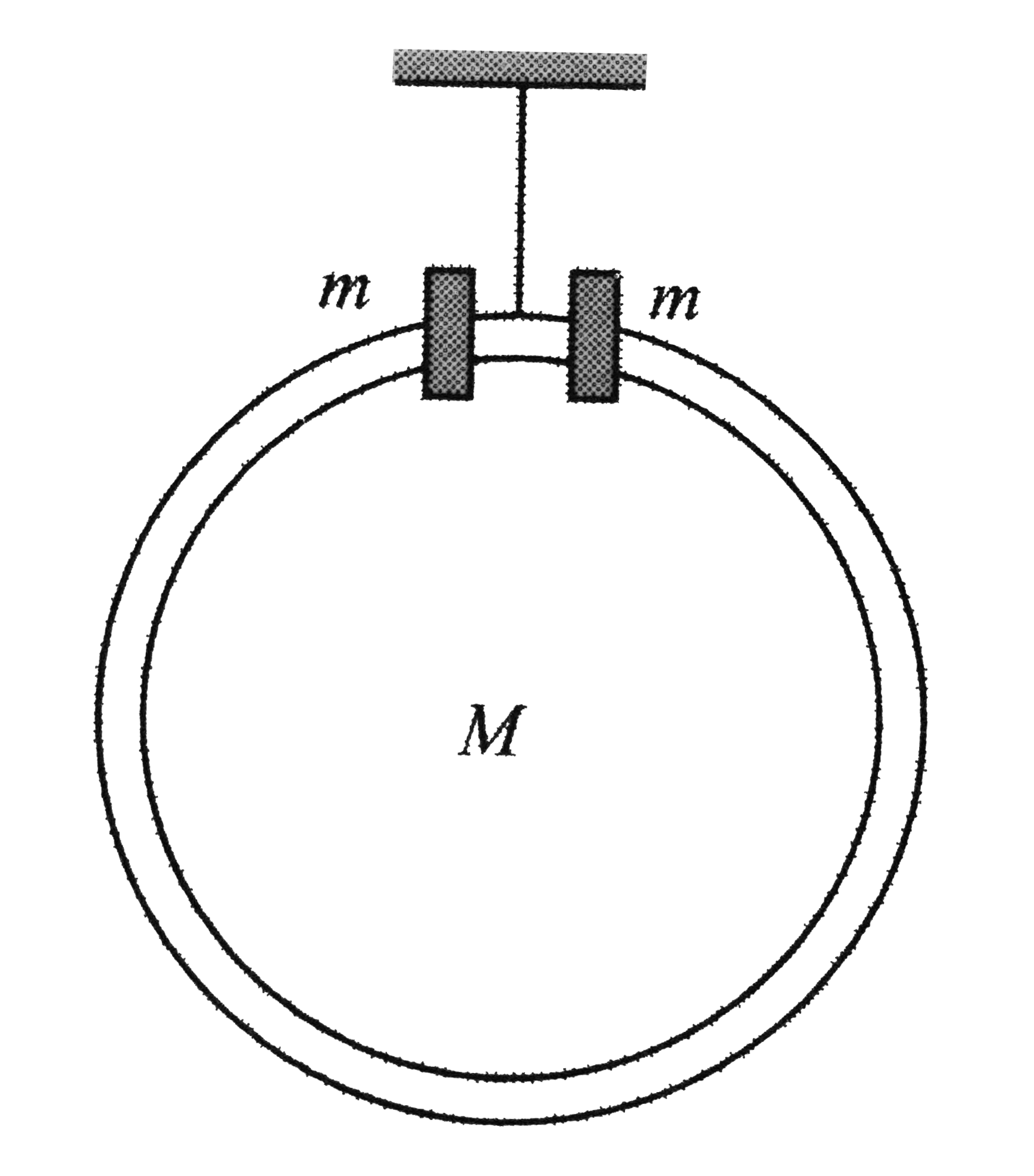
Suppose the string slakens that means its tension T becomes zero when the rings slide through and angle θ with vertical as shown in figure. The speeds of the rings at the angular position θ can be given by conserving its energy between the position 1 and 2

⇒ΔPE=ΔKE
⇒mgh=12mv2
⇒mg[r−rcosθ]=12mv2
⇒v=√2gr(1−cosθ) (i)
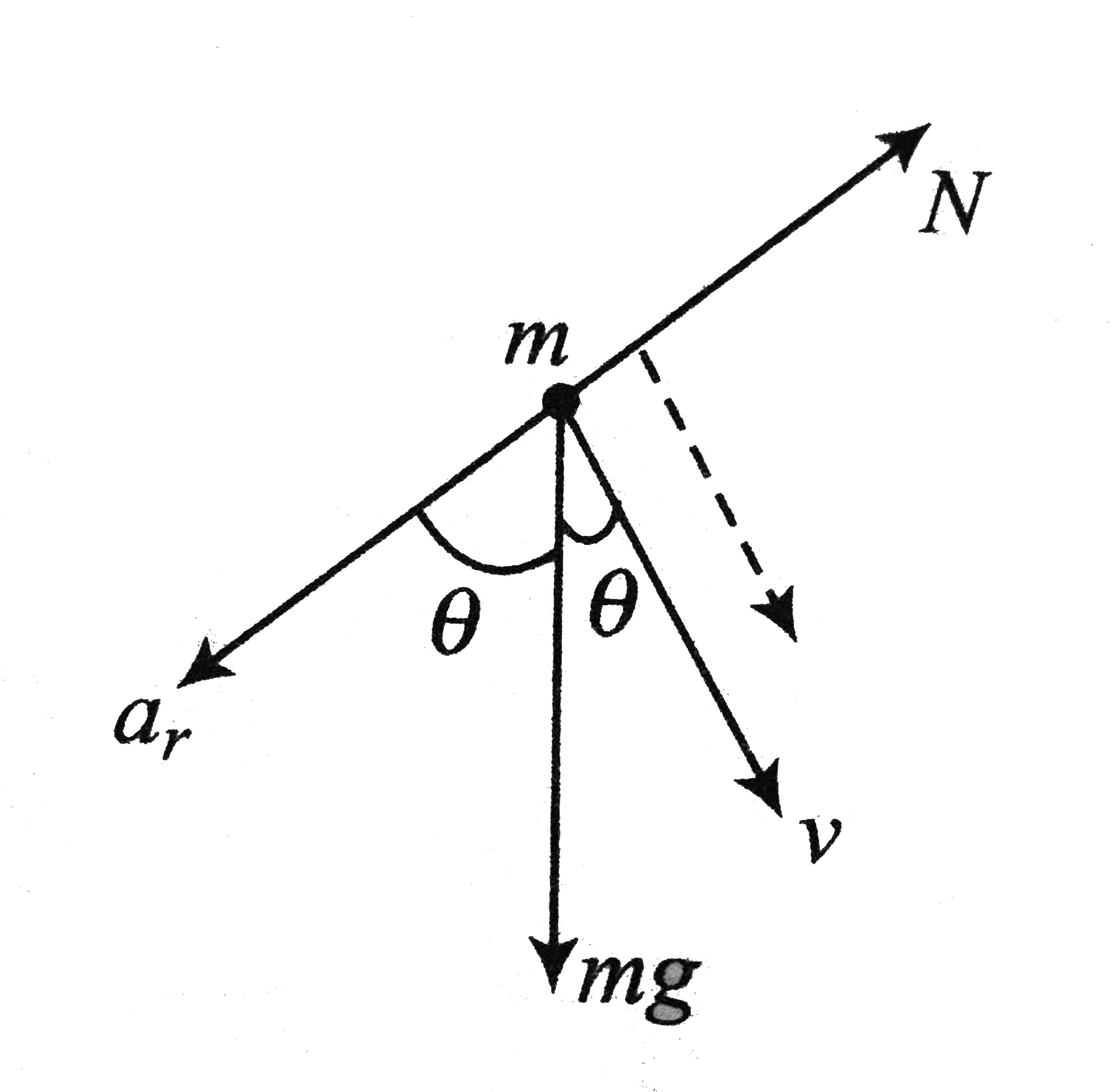
Referring figure, resolving the force acting on the ring radially we obtain
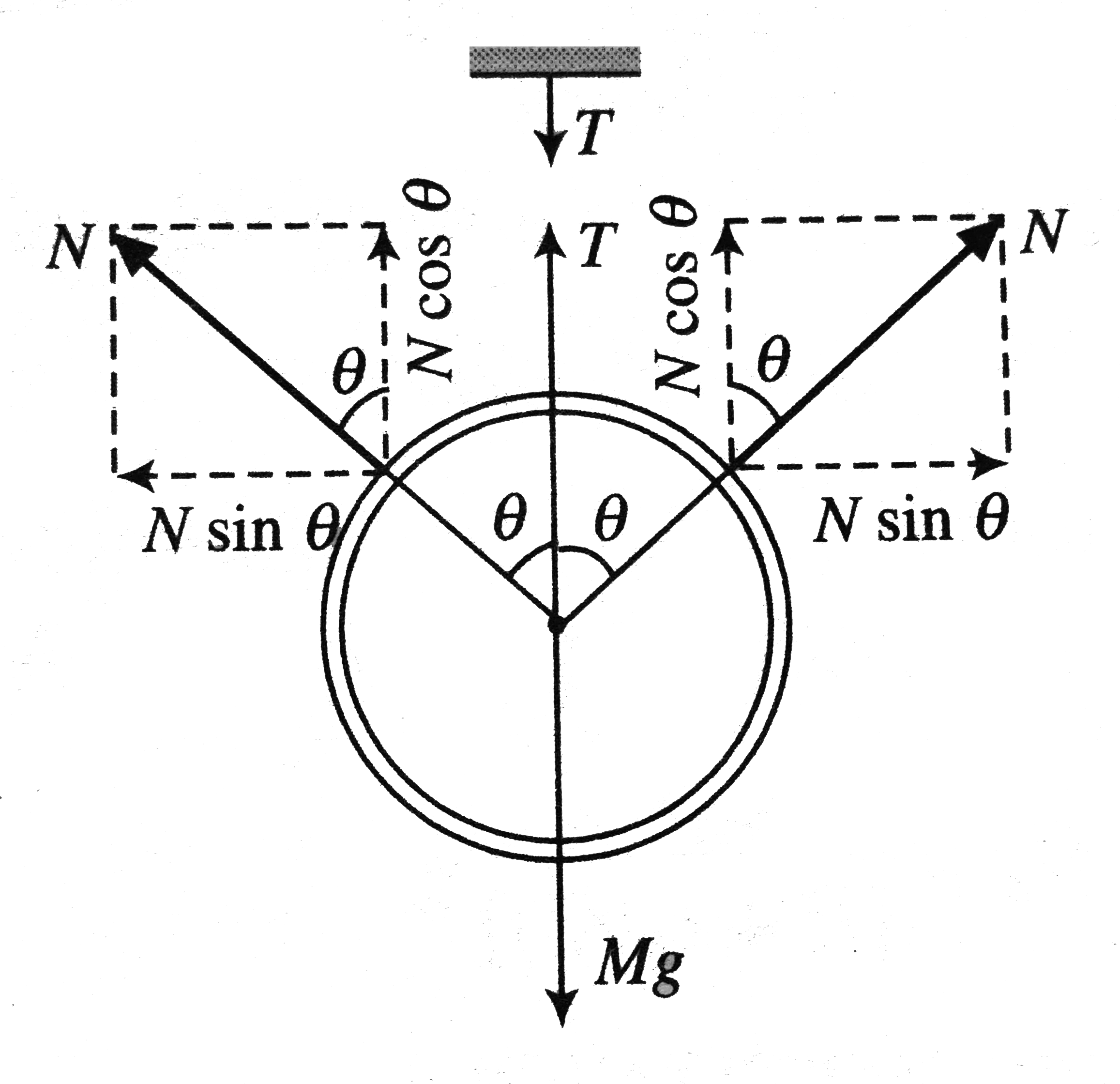
Fcp=mgcosθ+N
where N is the contacting force between the hoop and the rings.
mar=mgcosθ+N
⇒mv2r=mgcosθ+N
⇒N=mv2r−mgcosθ (ii)
Resolving the forces acting on the hoop for its equilibrium (refer figure we obtain)
Fx=Nsinθ−Nsinθ=0
and Fy=2Ncosθ+T−Mg=0
⇒2Ncosθ+T−Mg=0,
Since the string slackens,
T=0⇒N=Mg2cosθ (iii)
Using Eqs (ii) and (iii),
Mg2cosθ=mv2r−mgcosθ (iv)
Using Eqs (i) and (iv),
Mg2cosθ=mr{2gr(1−cosθ)}−mgcosθ
⇒3cos2θ−2cosθ+M2m=0 (v)
⇒cosθ=13[1±√(1−(3/2)(M/m))
If Mm≤23, if Mm>23
cosθ in imaginary, which means the string cannot be slakened.
If Mm=23, the quadratic equation (v) has one root, which means the string is slacken at θ=cos−1(1/3).
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.1
25 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.2
25 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Archives (integer)
4 Videos
- VECTORSBOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A loop of mass M with two identical rings of mass 32M each at its top hangs from a ceiling by an inextensible string. If the rings gently pushed horizontally in opposite directions, find the angular distance covered by each ring when the tension in the string vanishes for once during their motion.
View SolutionA mass M is hung with a light inextensible string as shown in Find the tension in the horizontal part of the string .
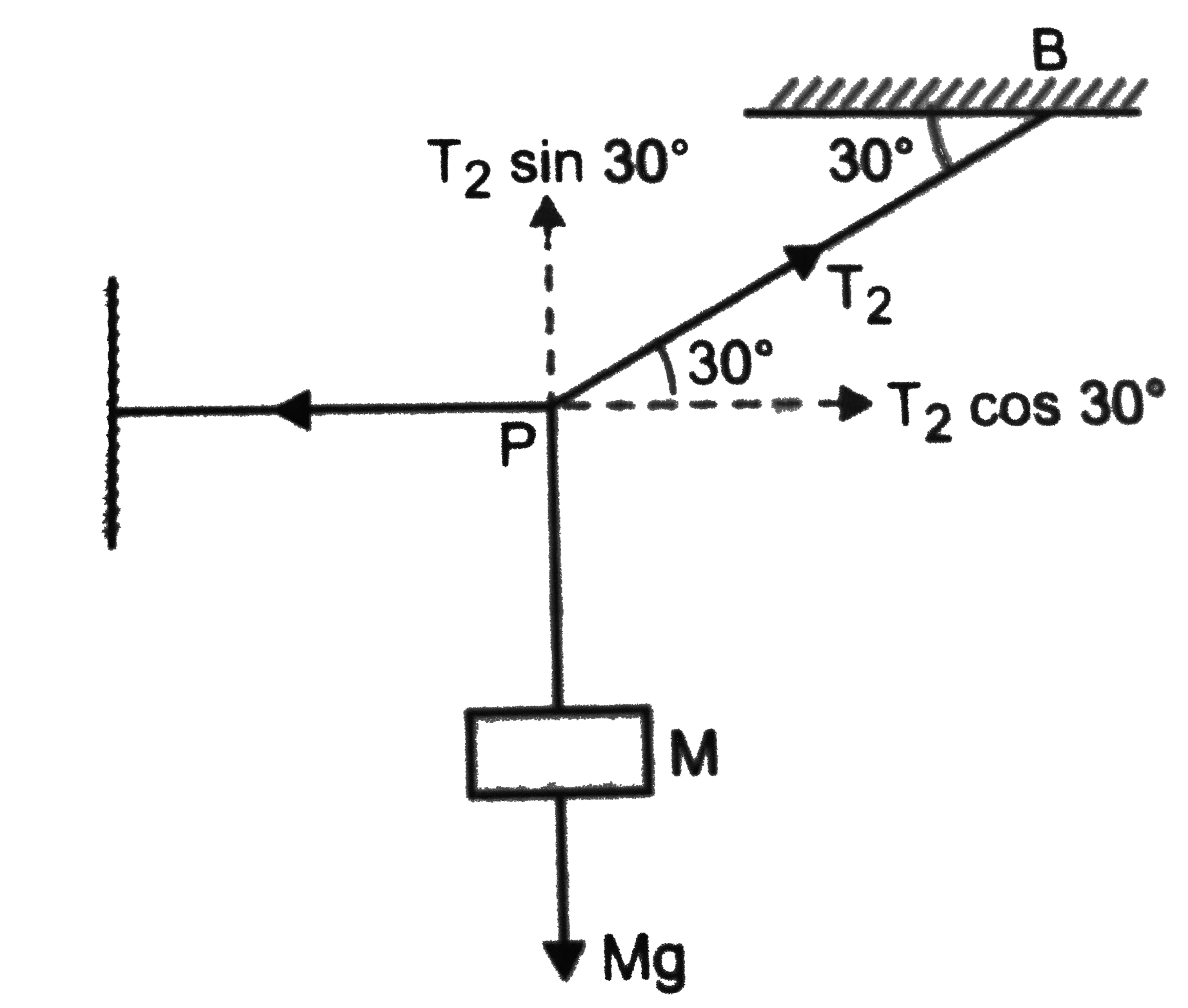
View Solution
Knowledge Check
A smooth circular track of mass M is vertically hung by a string down the ceiling. Two small rings, each of mass m, are initially at rest at the top of the track. They then slide down simultaneously along the track in opposite directions. Find the position of the rings when the tension in the string is zero.
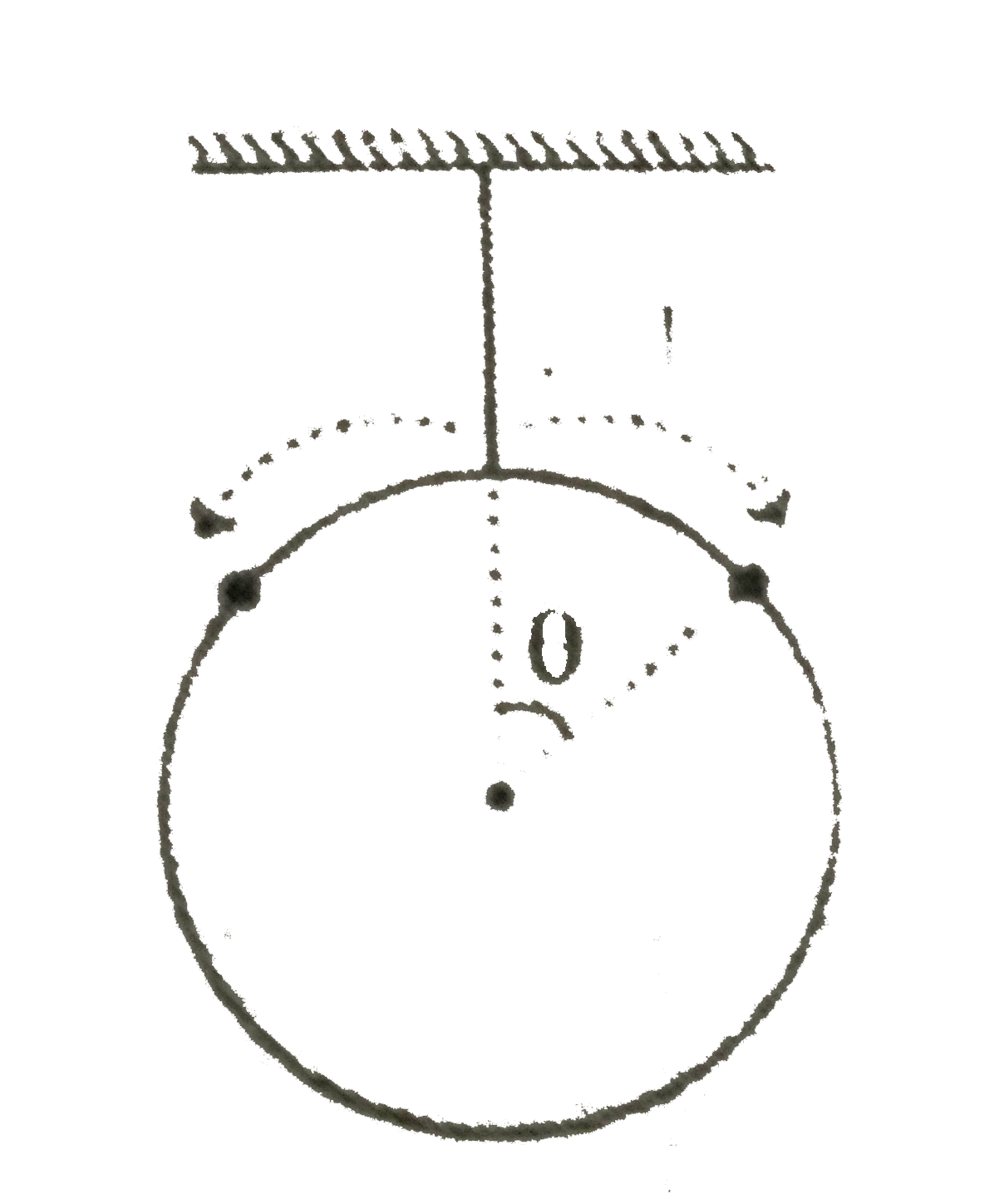 Aθ=sin−1[13(1+√1−GM3m)]Bθ=cos−1[53(1+√1−3M2m)]Cθ=cos−1[13(1+√1−3M2m)]Dθ=cos−1[13(1+√1−5M3m)]
Aθ=sin−1[13(1+√1−GM3m)]Bθ=cos−1[53(1+√1−3M2m)]Cθ=cos−1[13(1+√1−3M2m)]Dθ=cos−1[13(1+√1−5M3m)]A body is hanging from a rigid support. by an inextensible string of length '1' . It is struck inelastically by an identical body of mass in with horizontal velocity v=√2gl the tension in the string increases just after the striking by
AmgB3mgC2mgDnone of these
A mass M is hung with a light inextensible string as shown in Find the tension in the horizontal part of the string .

View SolutionA smooth of mass m1 is lying on a rigid horizontal string A bob of mass m2 hangs from, the ring by an inextensible light string of length l. Find angular frequency of oscillation of the system.
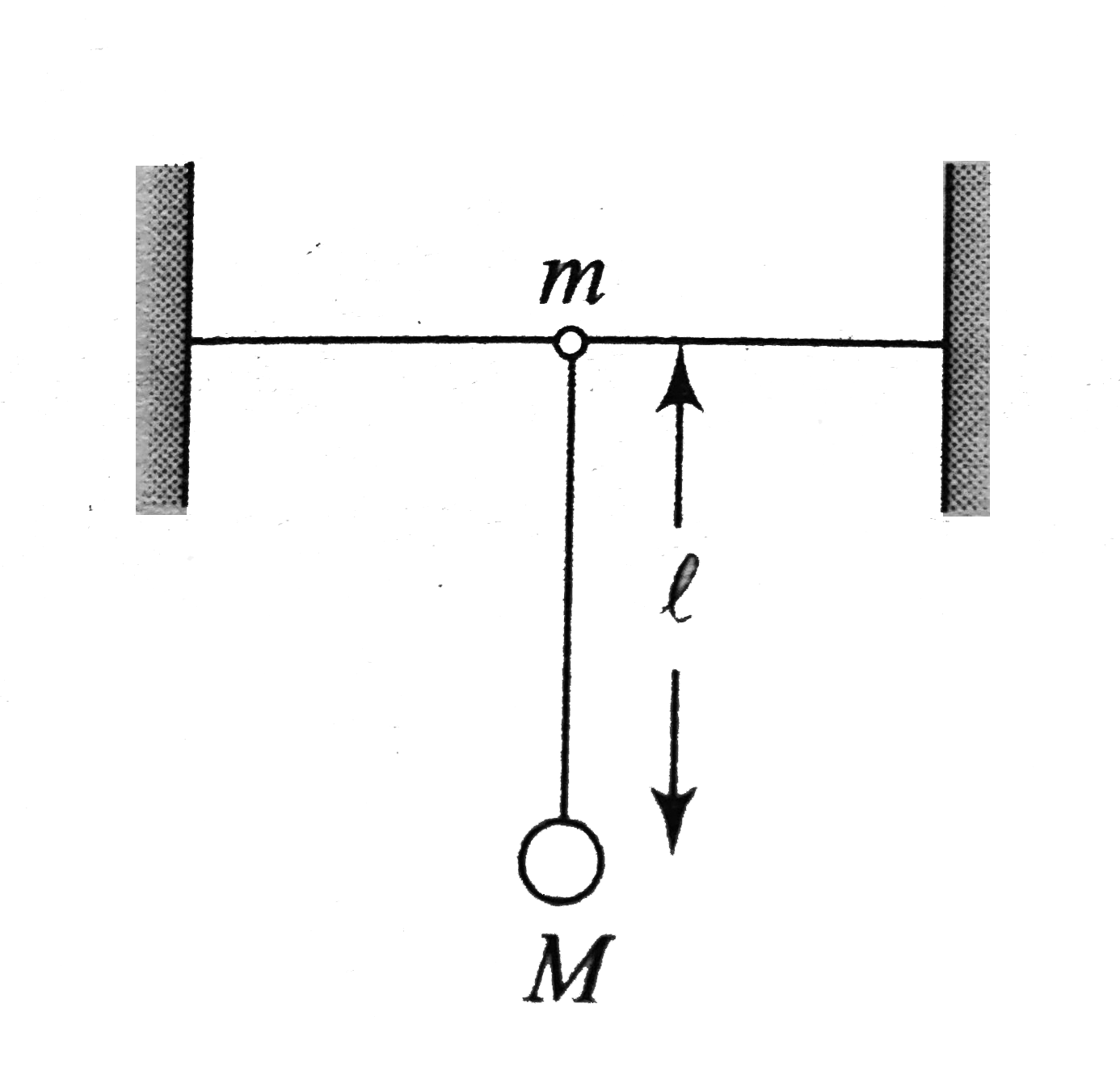
View SolutionA smooth of mass m1 is lying on a rigid horizontal string A bob of mass m2 hangs from, the ring by an inextensible light string of length l. Find angular frequency of oscillation of the system.

View SolutionA smooth circular track of mass M is vertically hung by a string down the ceiling. Two small rings, each of mass m, are initially at rest at the top of the track. They then slide down simultaneously along the track in opposite directions. Find the position of the rings when the tension in the string is zero.

View SolutionA body of mass M is hanging by an inextensible string of mass m. If the free end of the string accelerates up with constant acceleration a. find the variation of tension in the string a function of the distance measured from the mass M (bottom of the string).

View SolutionA body of mass M is hanging by an inextensible string of mass m. If the free end of the string accelerates up with constant acceleration a. find the variation of tension in the string a fuction of the distance measured from the mass M (bottom of the string).

View Solution
