A loop of wire enclosing an area S is placed in a region where the magnetic field is perpendicular to the plane. The magnetic field B varies with time according to the expression B=B0e−at where a is some constant. That is, at t=0. The field is B0 and for t>0, the field decreases exponentially. Find the induced emf in the loop as a function of time.
The correct Answer is:A, B
To find the induced emf in the loop as a function of time, we will follow these steps:
Step 1: Understand the Magnetic Flux
The magnetic flux (Φ) through the loop is given by the formula:
Φ=B⋅A
where B is the magnetic field and A is the area of the loop. Since the magnetic field is perpendicular to the plane of the loop, we can simplify this to:
Φ=B⋅S
where S is the area of the loop.
Step 2: Substitute the Expression for Magnetic Field
The magnetic field varies with time according to the expression:
B=B0e−at
Substituting this into the magnetic flux equation gives:
Φ=B0e−at⋅S
Step 3: Apply Faraday's Law of Electromagnetic Induction
According to Faraday's law, the induced emf (ε) in the loop is given by the negative rate of change of magnetic flux:
ε=−dΦdt
Substituting the expression for magnetic flux:
ε=−ddt(B0Se−at)
Step 4: Differentiate the Magnetic Flux
Now, we differentiate the expression:
ε=−B0Sddt(e−at)
Using the chain rule, the derivative of e−at is:
ddt(e−at)=−ae−at
Thus, substituting this back into the equation gives:
ε=−B0S(−ae−at)=aB0Se−at
Step 5: Final Expression for Induced EMF
Therefore, the induced emf as a function of time is:
ε(t)=aB0Se−at
Summary
The induced emf in the loop as a function of time is:
ε(t)=aB0Se−at
---
The magnetic flux (Φ) through the loop is given by the formula:
Φ=B⋅A
where B is the magnetic field and A is the area of the loop. Since the magnetic field is perpendicular to the plane of the loop, we can simplify this to:
Φ=B⋅S
where S is the area of the loop.
The magnetic field varies with time according to the expression:
B=B0e−at
Substituting this into the magnetic flux equation gives:
Φ=B0e−at⋅S
According to Faraday's law, the induced emf (ε) in the loop is given by the negative rate of change of magnetic flux:
ε=−dΦdt
Substituting the expression for magnetic flux:
ε=−ddt(B0Se−at)
Now, we differentiate the expression:
ε=−B0Sddt(e−at)
Using the chain rule, the derivative of e−at is:
ddt(e−at)=−ae−at
Thus, substituting this back into the equation gives:
ε=−B0S(−ae−at)=aB0Se−at
Therefore, the induced emf as a function of time is:
ε(t)=aB0Se−at
The induced emf in the loop as a function of time is:
ε(t)=aB0Se−at
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 2 Single Correct
37 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 2 More Than One Correct
10 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective Questions
3 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery
97 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
Similar Questions
A rectangular coil is placed in a region having a uniform magnetic field B perpendicular to the plane of the coil. An emf will not be induced ion the coil if the

View SolutionA closed loop with the geometry shown in Fig. 3.9 is placed in a uniform magnetic field direction into the plane of the paper. If the magnetic field decreases with time, determine the direction of the induced emf in this loop.
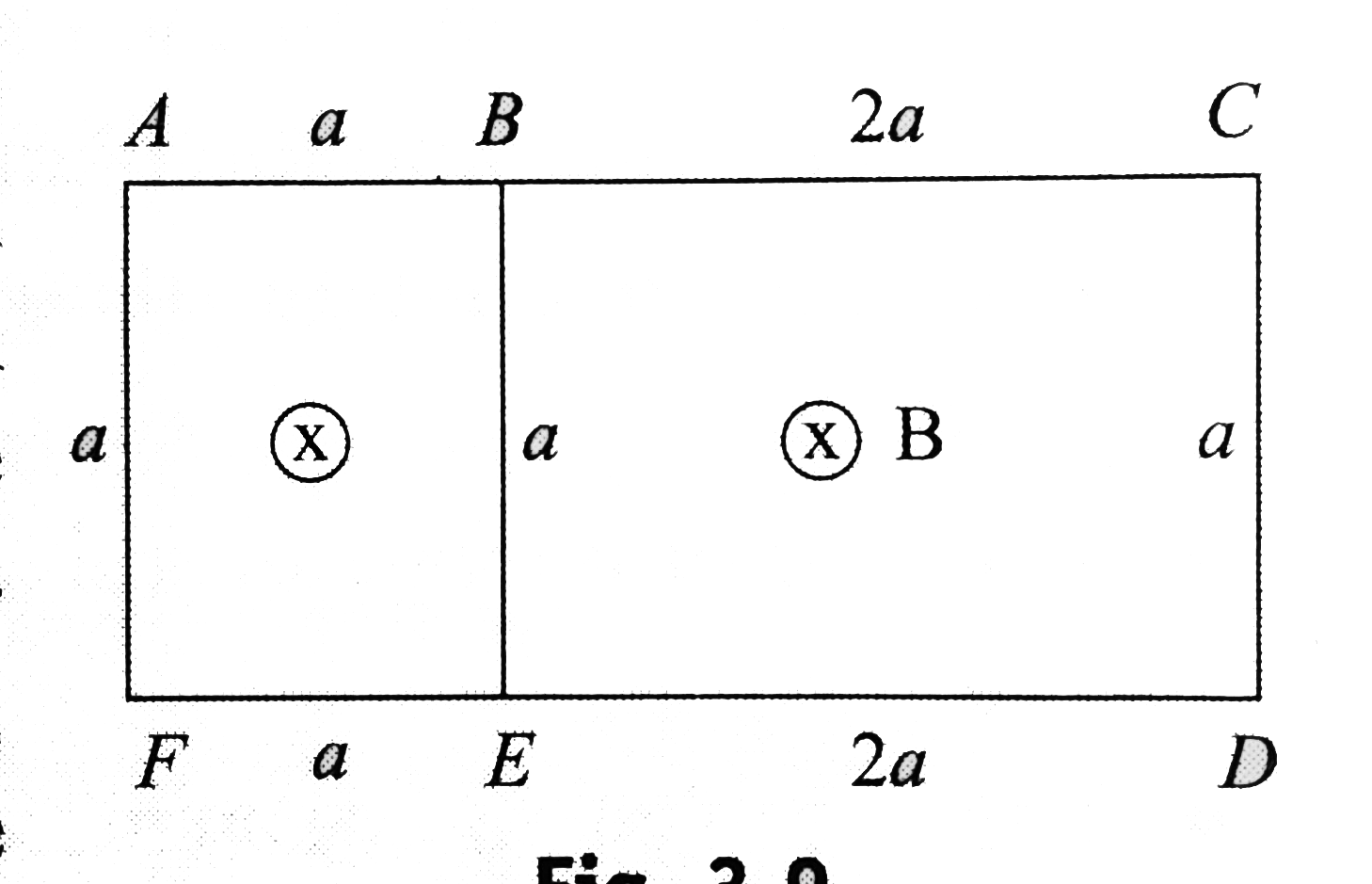
View Solution
Knowledge Check
A conducting metal circular-wire-loop of radius r is placed perpendicular to a magnetic field which varies with time as B=B0e−t/τ, where B0andτ are constants, at time = 0. If the resistance of the loop is R then the heat generated in the loop after a long time (t→∞) is :
Aπ2r4B402τRBπ2r4B202τRCπ2r4B20RτDπ2r4B20τRA conducting circular loop of radius r carries a constant current i. It is placed in a uniform magnetic field B such that B is perpendicular to the plane of loop. What is the magnetic force acting on the loop?
AI r BB2πrIBCzeroDπrIB
The magnetic field perpendicular to the plane of a loop of area 0.1m2 is 0.2 T. Calculate the magnetic flux through the loop (in weber)
View SolutionA circular loop of area 1cm2 , carrying a current of 10 A , is placed in a magnetic field of 0.1 T perpendicular to the plane of the loop. The torque on the loop due to the magnetic field is
View SolutionA semicircular loop of radius R is rotated with an angular velocity ω perpendicular to the plane of a magnetic field B as shown in the figure. Emf Induced in the loop is

View SolutionA conducting ring of radius r and resistance R is placed in region of uniform time varying magnetic field B which is perpendicular to the plane of the ring. It the magnetic field is changing at a rate α, then the current induced in the ring is
View SolutionA conducting metal circular-wire-loop of radius r is placed perpendicular to a magnetic field which varies with time as B=B0e−t/τ, where B0andτ are constants, at time = 0. If the resistance of the loop is R then the heat generated in the loop after a long time (t→∞) is :
View SolutionA conducting loop of radius R is present in a uniform magnetic field B perpendicular to the plane of the ring. If radius R varies as a function of time t, as R=R0+t. The emf induced in the loop is

View Solution
