A parallel plate capacitor (C=50μF.d=4mm) is charged to 200V and then charging battery is removed. Now a dielectric slab (K=4) of thickness 2mm is placed between the plates. Find new potential difference across capacitor.
To solve the problem step-by-step, we will follow the outlined procedure to find the new potential difference across the capacitor after inserting the dielectric slab.
Step 1: Calculate the initial charge on the capacitor
The charge Q on the capacitor can be calculated using the formula:
Q=C×V
where:
- C=50μF=50×10−6F
- V=200V
Calculating Q:
Q=50×10−6F×200V=10×10−3C=10mC
Hint for Step 1: Remember that the charge on the capacitor remains constant when the battery is removed.
Step 2: Analyze the capacitor with the dielectric slab
The dielectric slab has a dielectric constant K=4 and a thickness of 2mm. The total distance between the plates is d=4mm. Therefore, the remaining distance without the dielectric is:
d′=d−thickness of dielectric=4mm−2mm=2mm
Step 3: Calculate the capacitance with the dielectric
The capacitor can be viewed as two capacitors in series:
1. Capacitor C1 with the dielectric slab:
C1=Kϵ0Ad1=4ϵ0A2mm
2. Capacitor C2 without the dielectric:
C2=ϵ0Ad2=ϵ0A2mm
Where:
- d1=2mm (thickness of the dielectric)
- d2=2mm (remaining distance)
Step 4: Find the equivalent capacitance
The equivalent capacitance C′ for capacitors in series is given by:
1C′=1C1+1C2
Substituting the values:
1C′=14ϵ0A2mm+1ϵ0A2mm
This simplifies to:
1C′=2mm4ϵ0A+2mmϵ0A=2mm+8mm4ϵ0A=10mm4ϵ0A
Thus:
C′=4ϵ0A10mm=2ϵ0A5mm
Step 5: Relate charge and new potential difference
Using the relationship Q=C′V′, we can solve for the new potential difference V′:
Q=C×V=C′×V′
Substituting the known values:
10mC=(8C5)V′
Since C=50μF, we can cancel C:
10×10−3=(8×50×10−65)V′
This gives:
10×10−3=(80×10−6)V′
Solving for V′:
V′=10×10−380×10−6=1080×103=18×1000=125V
Final Answer
The new potential difference across the capacitor is V′=125V.
---
The charge Q on the capacitor can be calculated using the formula:
Q=C×V
where:
- C=50μF=50×10−6F
- V=200V
Q=50×10−6F×200V=10×10−3C=10mC
The dielectric slab has a dielectric constant K=4 and a thickness of 2mm. The total distance between the plates is d=4mm. Therefore, the remaining distance without the dielectric is:
d′=d−thickness of dielectric=4mm−2mm=2mm
The capacitor can be viewed as two capacitors in series:
1. Capacitor C1 with the dielectric slab:
C1=Kϵ0Ad1=4ϵ0A2mm
2. Capacitor C2 without the dielectric:
C2=ϵ0Ad2=ϵ0A2mm
- d1=2mm (thickness of the dielectric)
- d2=2mm (remaining distance)
The equivalent capacitance C′ for capacitors in series is given by:
1C′=1C1+1C2
Substituting the values:
1C′=14ϵ0A2mm+1ϵ0A2mm
This simplifies to:
1C′=2mm4ϵ0A+2mmϵ0A=2mm+8mm4ϵ0A=10mm4ϵ0A
Thus:
C′=4ϵ0A10mm=2ϵ0A5mm
Using the relationship Q=C′V′, we can solve for the new potential difference V′:
Q=C×V=C′×V′
Substituting the known values:
10mC=(8C5)V′
Since C=50μF, we can cancel C:
10×10−3=(8×50×10−65)V′
This gives:
10×10−3=(80×10−6)V′
Solving for V′:
V′=10×10−380×10−6=1080×103=18×1000=125V
The new potential difference across the capacitor is V′=125V.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.1
15 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.2
15 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
After charging a capacitor the battery is removed. Now by placing a dielectric slab between the plates
View SolutionA capacitor with plate separation d is charged to V volts. The battry is disconnected and a dielectric slab of thickness d2 and dlelectric constant '2' is inserted between the plates. The potential difference across its terminals becomes
View SolutionTwo identical parallel plate capacitors are connected in series to a battery of 100V. A dielectric slab of dielectric constant 4.0 is inserted between the plates of second capacitor. The potential difference across the capacitors will now be respectively
View SolutionThe space between plates of a parallel plate capacitor is filled by a dielectric and it is charged and then battery is removed. Now dielectric slab is slowly drawn out of the capacitor parallel to the plates. The variation of the potential of capacitor with respect to the length of the dielectric plate drawn out is
View SolutionA capacitor is charged by a cell of emf E the charging battery is then removed. If an identical capacitor is now inserted in the circuit in parallel with the previous capacitor, the potential difference across the new capacitor is
View SolutionThe plates of a parallel plate capacitor are charged up to 200 V. A dielectric slab of thickness 4 mm is inserted between its plates. Then, to maintain the same potential difference between the plates of the capacitor, the distance between the plates increased by 3.2 mm. The dielectric constant of the dielectric slab is
View SolutionSeparation between the plates of parallel plate capacitor is 5 mm. this capacitor, having air as the dielectric medium between the plates, is charged to a potential difference 25 V using a battery. The battery is then disconnected and a dielectric slab of thickness 3mm and dielectric constant K=10 is placed between the plates as shown. potential difference between the plates after the dielectric slab has been introduced is-
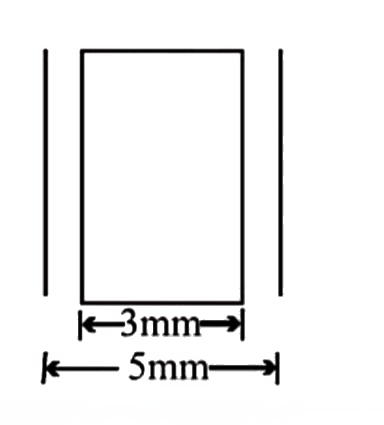
View SolutionThe parallel combination of two air filled parallel plate capacitor of capacitance C and nC is connected to a battery of voltage, V. When the capacitors are fulley charged, the battery is removed and after that a dielectric material of dielectric constant K is placed between the two plates of the first capacitors. the new potential difference of the combined system is
View SolutionTwo identical parallel plate capacitors are connected in series and then joined in series with a battery of 100V. A slab of dielectric constant K=3 is inserted between the plates of the first capacitor. Then, the potential difference across the capacitor will be, respectively.
View SolutionA parallel plate capacitor is charged by a battery. After sometime the battery is disconnected and a dielectric slab with its thickness equal to the plate separation is inserted between the plates. How will (i) the capacitance of the capacitor, (ii) potential difference between the plates and (iii) the energy stored in the capacitor be affected ?
Justify your answer in each case.
View Solution
