A particle is placed at the origin and a force F=Kx is acting on it (where k is a positive constant). If U(0)=0, the graph of U(x) verses x will be (where U is the potential energy function.)
The correct Answer is:A, B, C
To solve the problem, we need to find the potential energy function U(x) given the force F=kx and the condition that U(0)=0.
1. Understand the relationship between force and potential energy:
The relationship between force F and potential energy U is given by:
F=−dUdx
This means that the force is equal to the negative gradient (derivative) of the potential energy function.
2. Substitute the given force into the equation:
We are given that F=kx. Substituting this into the equation gives:
kx=−dUdx
3. Rearranging the equation:
We can rearrange the equation to express dU:
dU=−kxdx
4. Integrate both sides:
To find U, we need to integrate:
U=−∫kxdx
The integral of kx is:
U=−(kx22)+C
where C is the constant of integration.
5. Apply the condition U(0)=0:
We know that U(0)=0. Substituting x=0 into the equation gives:
U(0)=−k(0)22+C=0⟹C=0
Therefore, the potential energy function simplifies to:
U(x)=−kx22
6. Identify the nature of the function:
The function U(x)=−kx22 is a quadratic function that opens downwards (since the coefficient of x2 is negative). This indicates that the graph of U(x) versus x will be a downward-opening parabola.
7. Sketch the graph:
The graph will intersect the x-axis at x=0 and will decrease as |x| increases. The vertex of the parabola will be at the origin (0,0).
Final Answer:
The graph of U(x) versus x will be a downward-opening parabola with its vertex at the origin (0,0).
---
The relationship between force F and potential energy U is given by:
F=−dUdx
This means that the force is equal to the negative gradient (derivative) of the potential energy function.
We are given that F=kx. Substituting this into the equation gives:
kx=−dUdx
We can rearrange the equation to express dU:
dU=−kxdx
To find U, we need to integrate:
U=−∫kxdx
The integral of kx is:
U=−(kx22)+C
where C is the constant of integration.
We know that U(0)=0. Substituting x=0 into the equation gives:
U(0)=−k(0)22+C=0⟹C=0
Therefore, the potential energy function simplifies to:
U(x)=−kx22
The function U(x)=−kx22 is a quadratic function that opens downwards (since the coefficient of x2 is negative). This indicates that the graph of U(x) versus x will be a downward-opening parabola.
The graph will intersect the x-axis at x=0 and will decrease as |x| increases. The vertex of the parabola will be at the origin (0,0).
The graph of U(x) versus x will be a downward-opening parabola with its vertex at the origin (0,0).
Topper's Solved these Questions
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - TYPE2
1 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Miscellaneous Example
6 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question
11 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY
33 Videos
Similar Questions
On a particle placed at origin a variable force F=−ax(where a is a positive constant) is applied. If U(0)=0, the graph between potential energy of particle U(x) and x is best represented by:-
View SolutionA particle of mass m is executing osciallations about the origin on the x-axis with amplitude A. its potential energy is given as U(x)=αx4, where α is a positive constant. The x-coordinate of mass where potential energy is one-third the kinetic energy of particle is
View Solution
Knowledge Check
The potential energy function for a particle executing linear SHM is given by V(x)=12kx2 where k is the force constant of the oscillator. For k=0.5Nm−1, the graph of V(x) versus x is shown in the figure A particle of total energy E turns back when it reaches x=±xm.if V and K indicate the potential energy and kinetic energy respectively of the particle at x=+xm,then which of the following is correct?
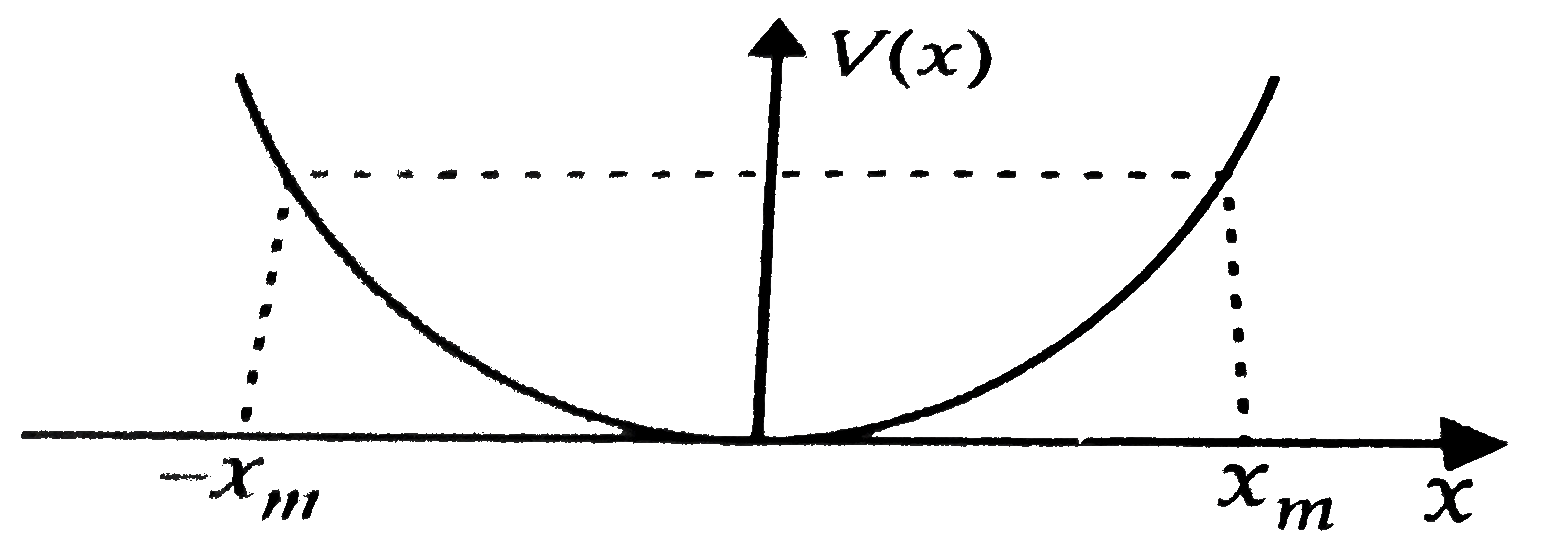 AV=0, K=EBV=E, K=0CV lt K, K=0DV=0, K lt E
AV=0, K=EBV=E, K=0CV lt K, K=0DV=0, K lt E
A particle, which is constrained to move along x-axis, is subjected to a force in the some direction which varies with the distance x of the particle from the origin an F(x)=−kx+ax3. Here, k and a are positive constants. For x(≥0, the functional form of the potential energy (u) U of the U (x) the particle is.
(a)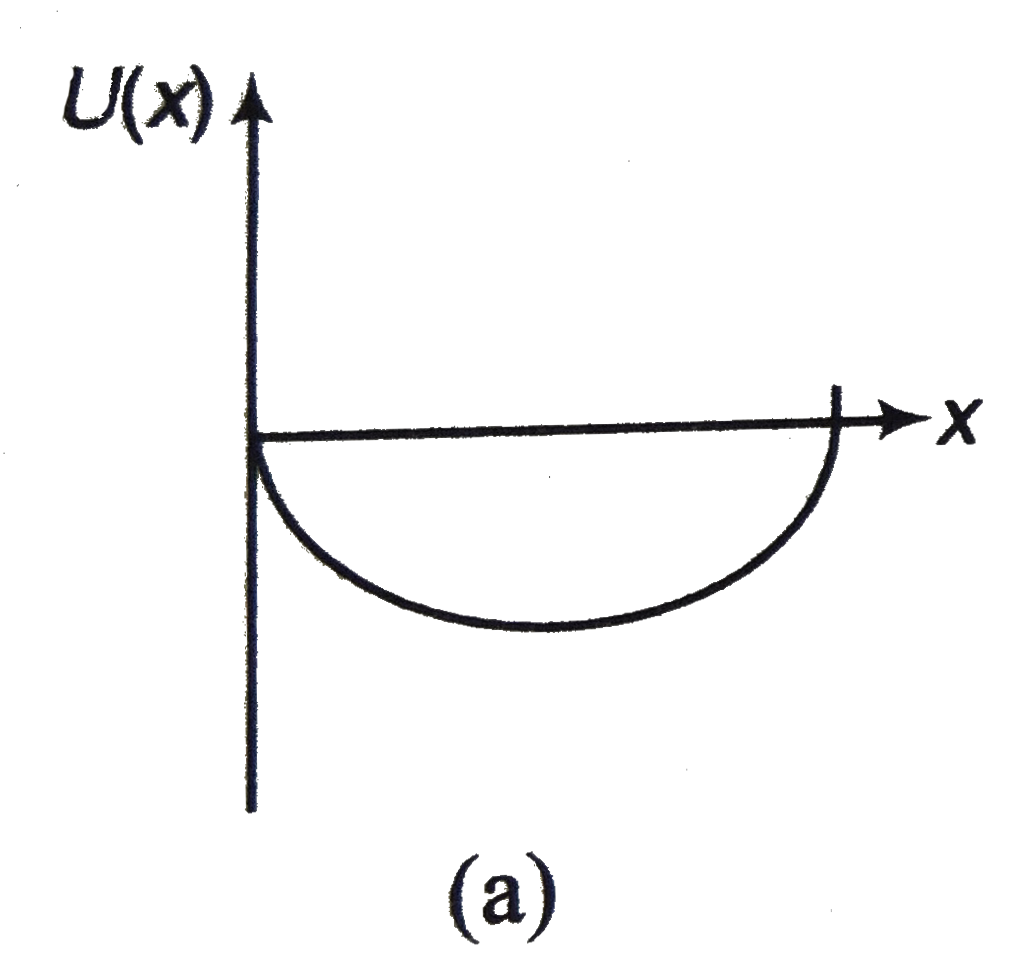 , (b)
, (b) 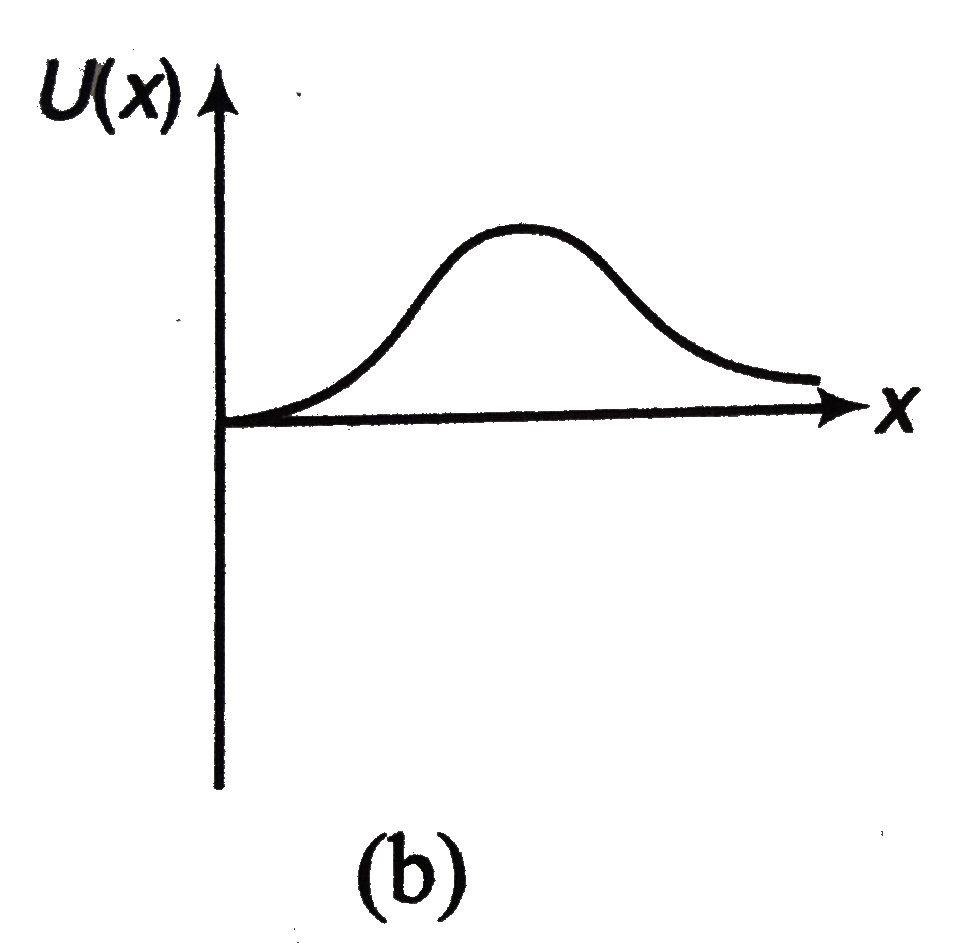 , (c)
, (c) 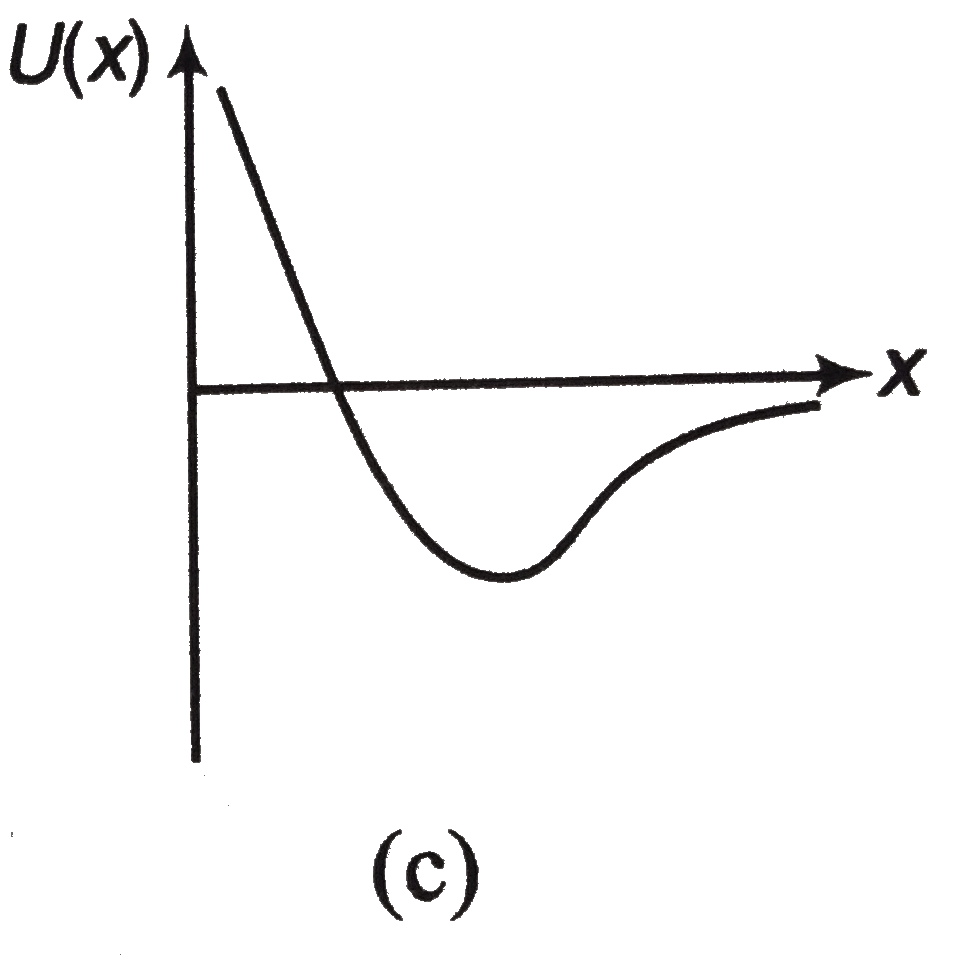 , (d)
, (d) 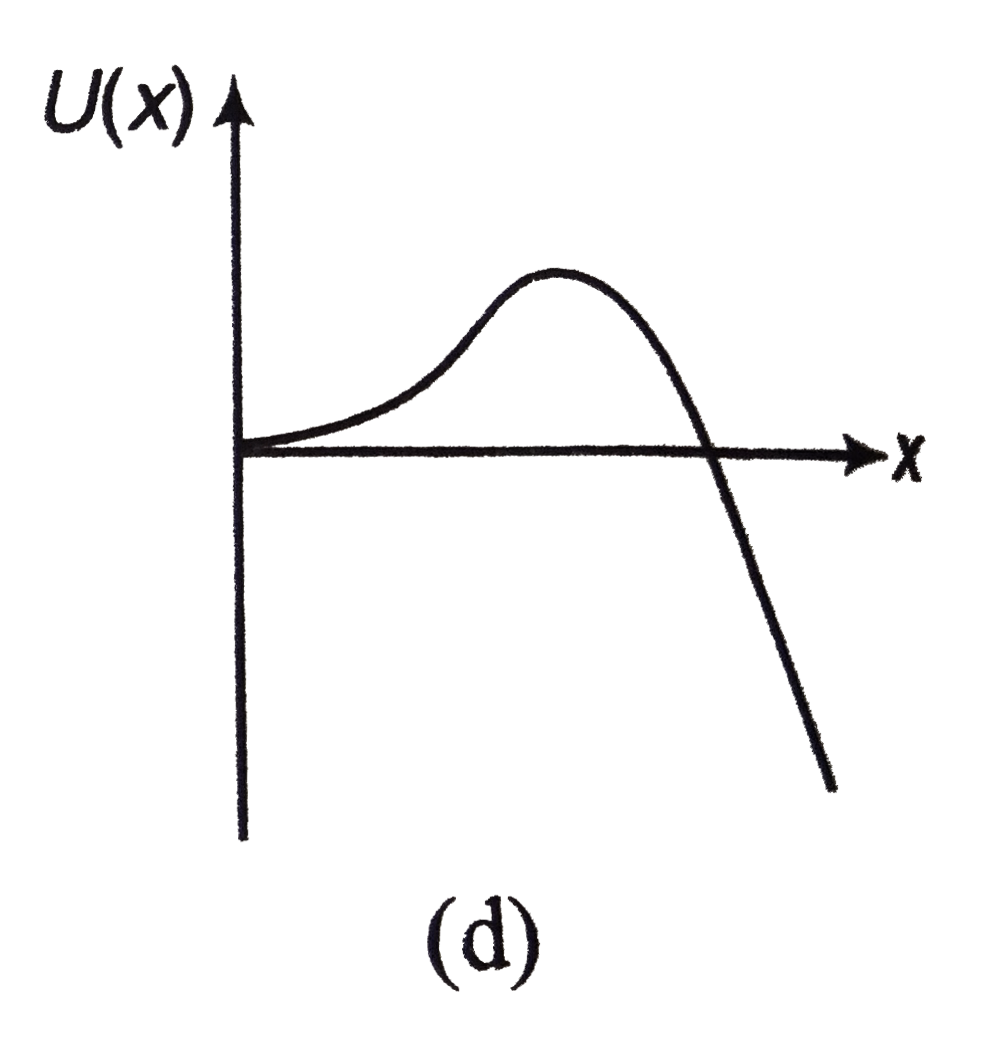 .
.
View SolutionThe potential energy U for a force field →F is such that U=−kxy where K is a constant . Then
View SolutionThe potential energy of configuration changes in x and y directions as U=kxy, where k is a positive constant. Find the force acting on the particle of the system as the function of x and y.
View SolutionA particle is acted by x force F=Kx where K is a(+ve) constant its potential energy at x=0 is zero . Which curve correctly represent the variation of potential energy of the block with respect to x
View SolutionA particle free to move along x-axis is acted upon by a force F=−ax+bx2whrteaandbarepositiveconstants., the correct variation of potential energy function U(x) is best represented by.
View SolutionA particle moving along the X-axis executes simple harmonic motion, then the force acting on it is given by
where, A and K are positive constants.
View SolutionA particle of mass m moves in a one dimensional potential energy U(x)=−ax2+bx4, where a and b are positive constant. The angular frequency of small oscillation about the minima of the potential energy is equal to
View Solution
