A particle moves according to the law, x=acos(πt/2). . What is the distance covered by it in time interval t=0 to t=3 second.
2a
3a
4a
a
The correct Answer is:B
To find the distance covered by the particle in the time interval from t=0 to t=3 seconds, we can follow these steps:
Step 1: Understand the motion
The motion of the particle is described by the equation:
x=acos(πt2)
This indicates that the particle is undergoing simple harmonic motion (SHM).
Step 2: Determine the time period
The angular frequency ω can be identified from the equation:
ω=π2
The time period T of the motion can be calculated using the formula:
T=2πω
Substituting the value of ω:
T=2ππ2=4 seconds
Step 3: Analyze the motion over one complete cycle
In one complete cycle (4 seconds), the particle moves from:
- Maximum displacement +a (at t=0)
- To the mean position 0 (at t=2 seconds)
- To the minimum displacement −a (at t=4 seconds)
Step 4: Calculate the distance covered in the first 2 seconds
From t=0 to t=2 seconds, the particle moves from +a to −a:
- Distance from +a to 0 is a
- Distance from 0 to −a is a
Thus, the total distance covered in the first 2 seconds is:
a+a=2a
Step 5: Calculate the distance covered from t=2 to t=3 seconds
At t=2 seconds, the particle is at the mean position 0. Now we need to find its position at t=3 seconds:
x(3)=acos(π⋅32)=acos(3π2)=a⋅0=0
The particle moves from 0 to 0 in this interval, but it actually moves to the maximum position +a and back to 0 in the next second.
The distance covered from t=2 to t=3 seconds is:
- From 0 to +a is a
- From +a back to 0 is a
So, the total distance covered in this interval is:
a
Step 6: Total distance covered
Now, we sum the distances covered in both intervals:
- Distance from t=0 to t=2 seconds: 2a
- Distance from t=2 to t=3 seconds: a
Thus, the total distance covered from t=0 to t=3 seconds is:
2a+a=3a
Final Answer
The distance covered by the particle in the time interval from t=0 to t=3 seconds is 3a.
---
The motion of the particle is described by the equation:
x=acos(πt2)
This indicates that the particle is undergoing simple harmonic motion (SHM).
The angular frequency ω can be identified from the equation:
ω=π2
The time period T of the motion can be calculated using the formula:
T=2πω
Substituting the value of ω:
T=2ππ2=4 seconds
In one complete cycle (4 seconds), the particle moves from:
- Maximum displacement +a (at t=0)
- To the mean position 0 (at t=2 seconds)
- To the minimum displacement −a (at t=4 seconds)
From t=0 to t=2 seconds, the particle moves from +a to −a:
- Distance from +a to 0 is a
- Distance from 0 to −a is a
a+a=2a
At t=2 seconds, the particle is at the mean position 0. Now we need to find its position at t=3 seconds:
x(3)=acos(π⋅32)=acos(3π2)=a⋅0=0
The particle moves from 0 to 0 in this interval, but it actually moves to the maximum position +a and back to 0 in the next second.
- From 0 to +a is a
- From +a back to 0 is a
a
Now, we sum the distances covered in both intervals:
- Distance from t=0 to t=2 seconds: 2a
- Distance from t=2 to t=3 seconds: a
2a+a=3a
The distance covered by the particle in the time interval from t=0 to t=3 seconds is 3a.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - JEE Advanced
34 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - More than one option is correct
50 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise 14.4
4 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
A particle moves according to the law x=rcos(pi)t/(2) The distance covered by it in the time interval between t=0 to t=3s is
View SolutionA particle moves according to the equation x=acosπt. The distance covered by it in 2.5 s is
View SolutionA particle moves in x−y plane according to the law x=4sin6tandy=4(1−cos6t). The distance traversed by the particle in 4 second is (x&y are in meters)
View SolutionThe diagram shows the displacement - time graph for a particle moving in a straight line. The distance (in m) coverted by the particle in the time interval t=0 to t=5s is
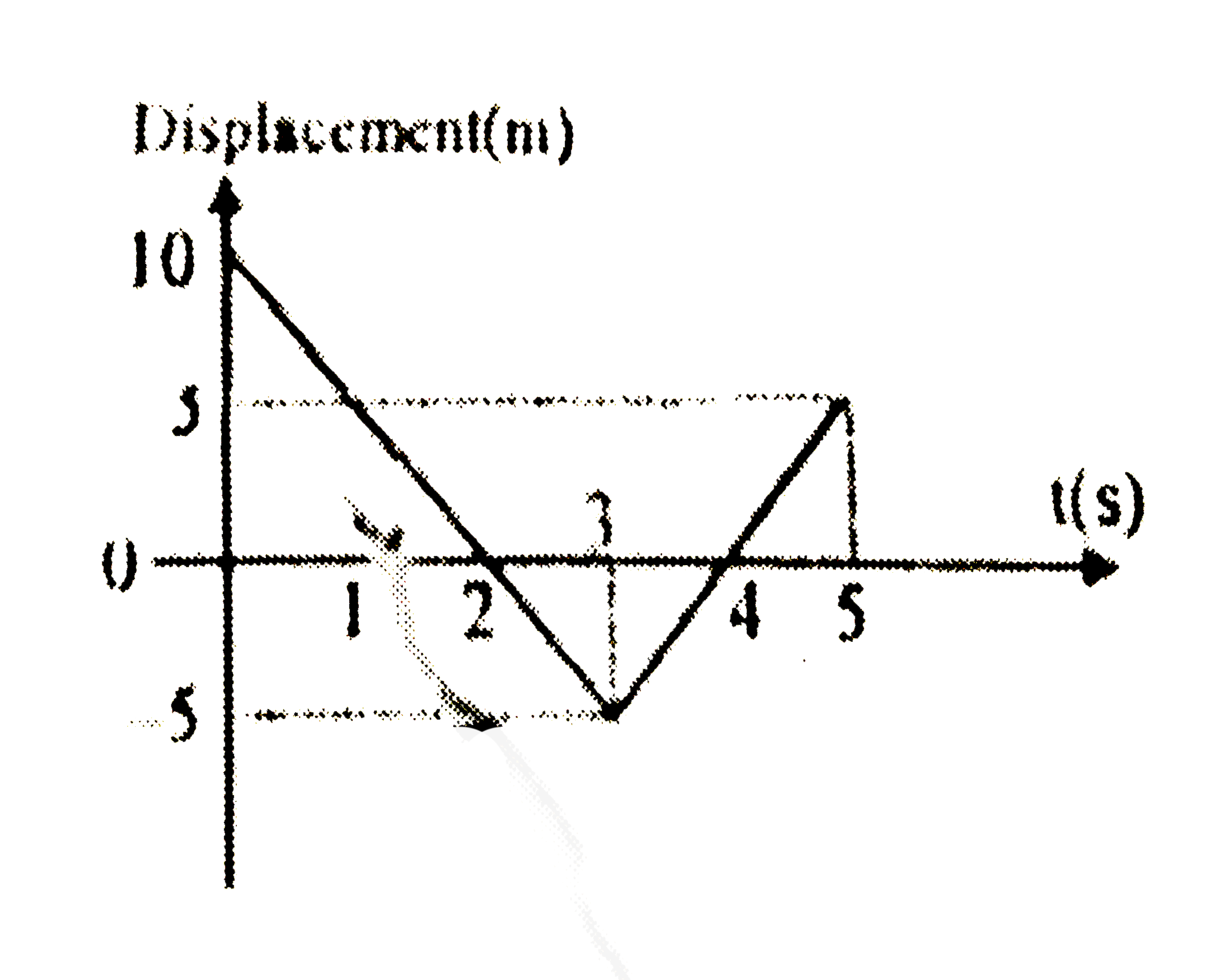
View SolutionA particle moves along the x - axis according to x=A[1+sinωt]. What distance does is travel in time interval from t=0 to t=2.5π/ω ?
View SolutionIf a particle moves according to the law s=6t2−t32, then the time at which it is momentarily at rest is
View SolutionA particle is moving in a straight line such that the distance covered by it in t seconds from a point is (t33−t) cm. find its speed at t=3 seconds.
View SolutionA particle is moving on a straight line. Its velocity at time t is (8−2t)m/s. What is the total distance covered from t=0 to t=6s?
View SolutionThe position of a particle along X-axis at time t is given by x=2+t−3t2. The displacement and the distance travelled in the interval, t = 0 to t = 1 are respectively
View SolutionA particle moves along a horizontal path, such that its velocity is given by v=(3t2−6t)m/s, where t is the time in seconds. If it is initially located at the origin O, determine the distance travelled by the particle in time interval from t=0 to t=3.5s and the particle's average velocity and average speed during the same time interval.
View Solution
