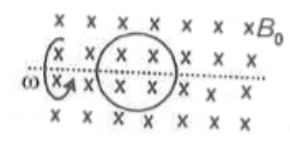
A rectangular loop and a circular loop are moving out of a uniform magnetic field to a field-free region with a constant velocity 'v' as shown in the figure . Explain the which loop do you expect the induced emf to the constant during the passage out of the field region . The magnetic field is normal to the loops .
To solve the question, we need to analyze the behavior of the induced electromotive force (emf) in both the rectangular and circular loops as they move out of a uniform magnetic field into a field-free region.
1. Understanding the Scenario:
- We have two loops: a rectangular loop and a circular loop.
- Both loops are moving with a constant velocity v out of a uniform magnetic field into a region where there is no magnetic field.
- The magnetic field is perpendicular (normal) to the plane of the loops.
2. Induced EMF and Faraday's Law:
- According to Faraday's Law of Electromagnetic Induction, the induced emf (E) in a loop is given by the negative rate of change of magnetic flux (ΦB) through the loop:
E=−dΦBdt
- The magnetic flux (ΦB) through a loop is defined as:
ΦB=B⋅A
where B is the magnetic field strength and A is the area of the loop within the magnetic field.
3. Analyzing the Rectangular Loop:
- As the rectangular loop moves out of the magnetic field, the area of the loop that is still within the magnetic field decreases at a constant rate.
- Since the width of the rectangular loop remains constant and it moves out at a constant velocity v, the rate of change of area (and thus the rate of change of magnetic flux) is constant.
- Therefore, the induced emf in the rectangular loop will also be constant during its passage out of the magnetic field.
4. Analyzing the Circular Loop:
- For the circular loop, as it moves out of the magnetic field, the area that is within the magnetic field does not decrease at a constant rate.
- The circular loop has a varying radius, and as it exits the magnetic field, the effective area that is still within the field changes non-linearly.
- This results in a non-constant rate of change of magnetic flux, leading to a varying induced emf during its passage out of the magnetic field.
5. Conclusion:
- The induced emf in the rectangular loop is constant as it exits the magnetic field because the rate of change of area is constant.
- In contrast, the induced emf in the circular loop is not constant due to the non-linear change in area as it exits the magnetic field.
Final Answer:
The induced emf in the rectangular loop is expected to be constant during its passage out of the field region, while the induced emf in the circular loop is not constant.
- We have two loops: a rectangular loop and a circular loop.
- Both loops are moving with a constant velocity v out of a uniform magnetic field into a region where there is no magnetic field.
- The magnetic field is perpendicular (normal) to the plane of the loops.
- According to Faraday's Law of Electromagnetic Induction, the induced emf (E) in a loop is given by the negative rate of change of magnetic flux (ΦB) through the loop:
E=−dΦBdt
- The magnetic flux (ΦB) through a loop is defined as:
ΦB=B⋅A
where B is the magnetic field strength and A is the area of the loop within the magnetic field.
- As the rectangular loop moves out of the magnetic field, the area of the loop that is still within the magnetic field decreases at a constant rate.
- Since the width of the rectangular loop remains constant and it moves out at a constant velocity v, the rate of change of area (and thus the rate of change of magnetic flux) is constant.
- Therefore, the induced emf in the rectangular loop will also be constant during its passage out of the magnetic field.
- For the circular loop, as it moves out of the magnetic field, the area that is within the magnetic field does not decrease at a constant rate.
- The circular loop has a varying radius, and as it exits the magnetic field, the effective area that is still within the field changes non-linearly.
- This results in a non-constant rate of change of magnetic flux, leading to a varying induced emf during its passage out of the magnetic field.
- The induced emf in the rectangular loop is constant as it exits the magnetic field because the rate of change of area is constant.
- In contrast, the induced emf in the circular loop is not constant due to the non-linear change in area as it exits the magnetic field.
The induced emf in the rectangular loop is expected to be constant during its passage out of the field region, while the induced emf in the circular loop is not constant.
Topper's Solved these Questions
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET -III
4 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - PHYSICS (Theory) [SET -I]
25 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET -I
29 Videos
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D
6 Videos
Similar Questions
(a) A closed loop is held stationary in the magnetic field between the north and south poles of two permanent magnets held fixed. Can we hope to generate current in the loop by using very strong magnets?
(b) A closed loop moves normal to the constant electric field between the plates of a large capacitor. Is a current induced in the loop (i) when it is wholly inside the region between the capacitor plates (ii) when it is partially outside the plates of the capacitor? The electric field is normal to the plane of the loop.
(c) A rectangular loop and a circular loop are moving out of a uniform magnetic field region (Fig. 6.8) to a field-free region with a constant velocity v. In which loop do you expect the induced emf to be constant during the passage out of the field region? The field is normal to the loops.

(d) Predict the polarity of the capacitor in the situation described by Fig. 6.9.

View SolutionA current loop in a magnetic field
View SolutionA semi-circular loop of radius R is placed in a uniform magnetic field as shown. It is pulled with a constant velocity. The induced emf in the loop is
View SolutionA current carrying loop in a uniform magnetic field will experience
View SolutionA circular loop of radius 'a' is rotated in a uniform magnetic field B, with constant angular velocity o about diameter as shown in the figure. Choose the correct graph of induced emf & versus time t. (consider at t = 0 area vector of loop is in the direction of magnetic field)
View SolutionA rectangular, a square , a circular and an elliptical loop, all in the (x−y) plane, are moving out of a uniform magnetic field with a constant velocity →v=vˆi . The magnetic field is directed along the negative z-axis direction. The induced emf, during the passage of these loops , out of the field region, will not remain constant for :
View SolutionA metallic loop is placed in a nonuniform magnetic field. Will an emf be induced in the loop?
View SolutionA square loop of side a carris a current I. The magnetic field at the centre of the loop is
View SolutionAssertion A conducting loop is rotated in a uniform magnetic field with constant angular velocity ω as shown in figure. At time t = 0, plane of the loop is perpendicular to the magnetic field. Induced emf produced in the loop is maximum when plane of loop is parallel to magnetic field.
Reason When plane of loop is parallel to magnetic field, then magnetic flux passing through the loop is zero.
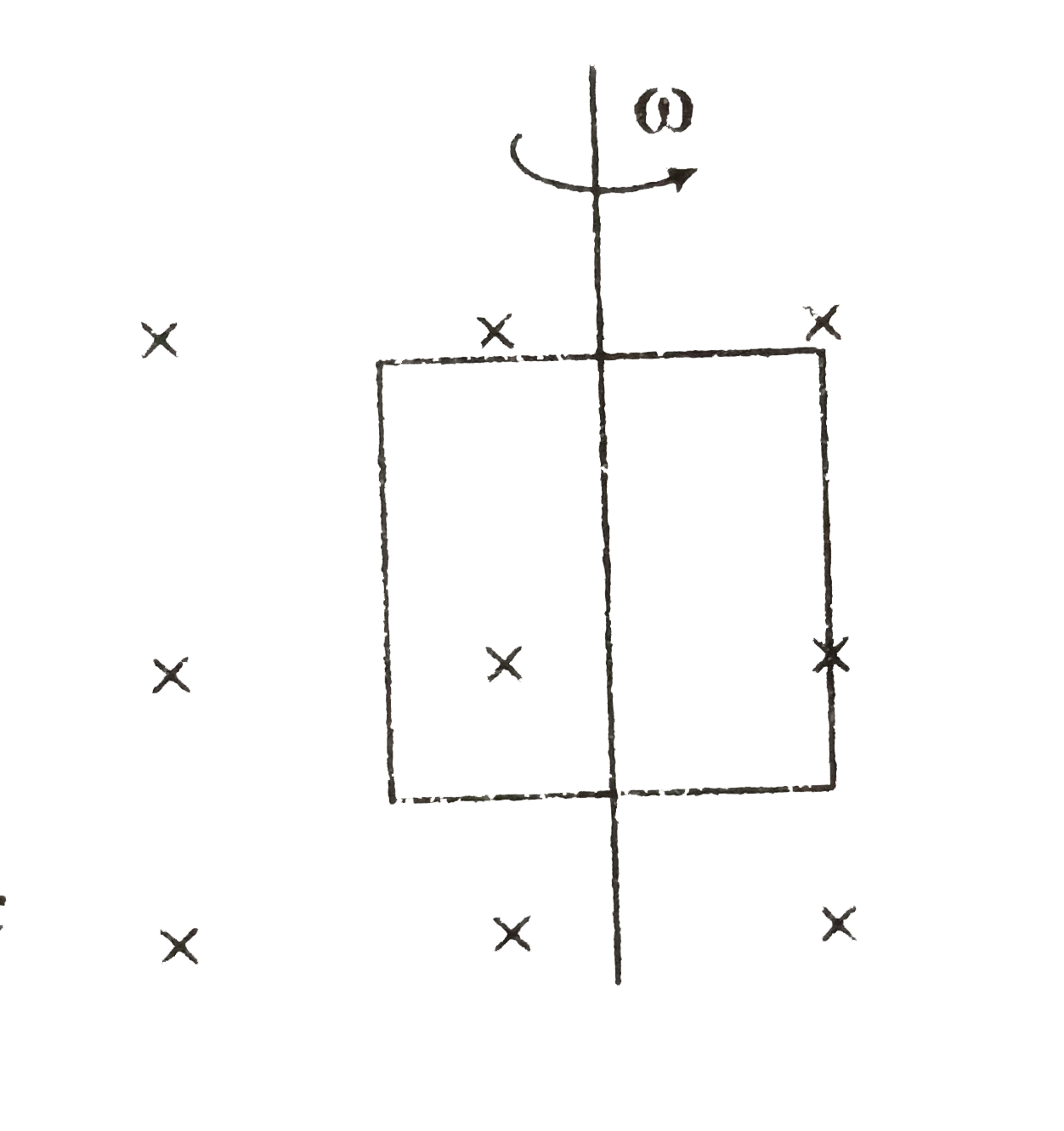
View SolutionRadius ol a circular luop placed in a perpendicular uniform magnetic field is increasing at a constant rate of roms1. If at any instant radius of the loop is r, then emf induced in the loop at that instant will be
View Solution
