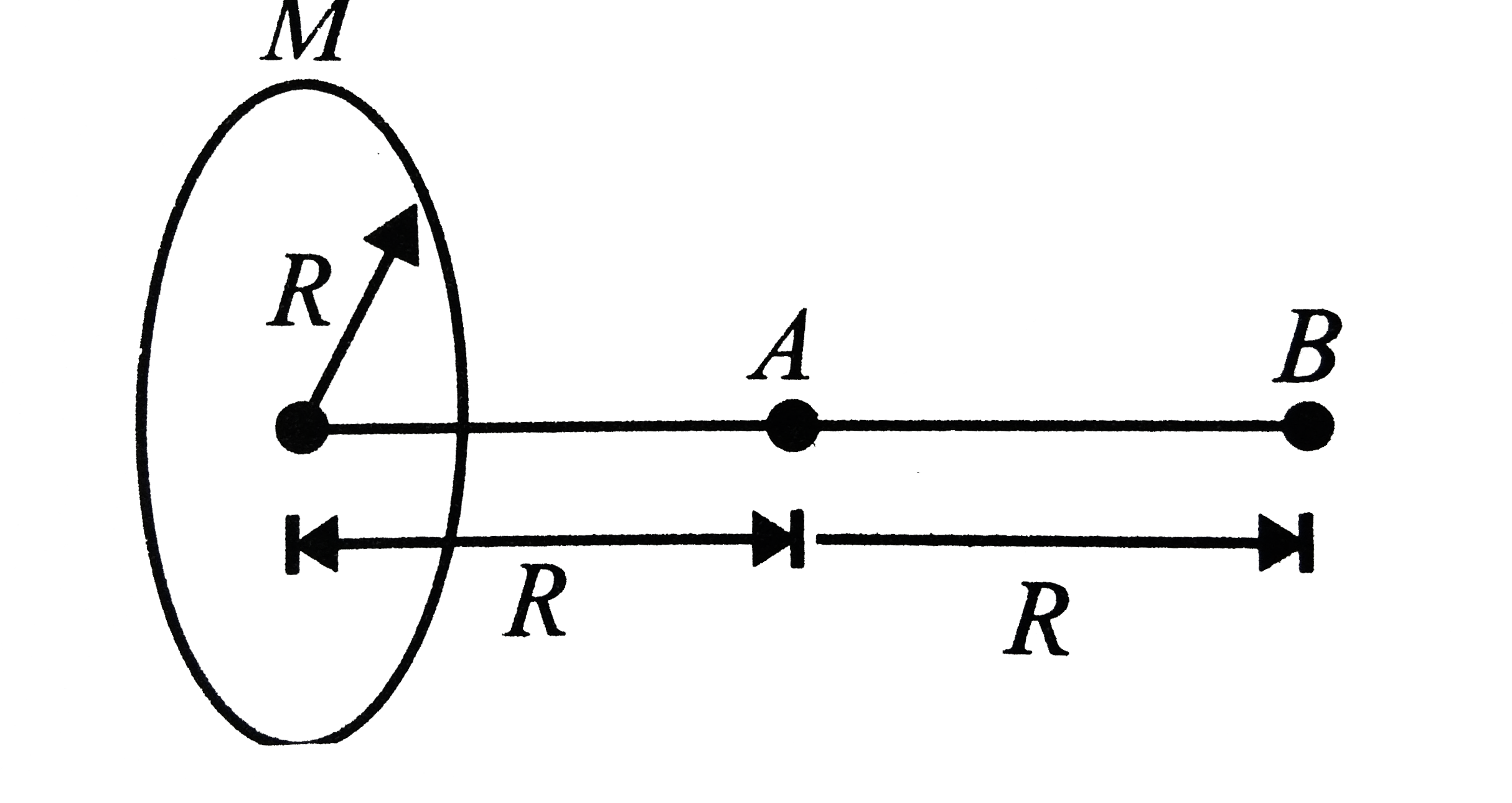
A ring having non-uniform distribution of mass M and radius R is being considered. A point mass m0 is taken slowly towards the ring. In doing so, work done by the external force against the gravitational force exerted by ring is
GMm0√2R
GMm0R[1√2−1√5]
GMm0R[1√5−1√2]
It is not possible to find the required work as the nature of distribution of mass is not known.
The correct Answer is:B
Even though the distribution of the mass is unknown, we can find the potential due to the ring on any axial point because from any axial point the entire mass is at the same distance (whatever woud be the nature of distribution).
Potential at A due to the ring is VA=GM√(2R)
Potential at B due to the ring is VB=GM√5R
dU=Uf−Ui−Ui=UB−UA=m0(VB−VA)
GMm0R[−1√5+1√2]
Wgr=−Wext
Wgr=dU=−Wext
Wext=dU=GMm0R[1√2−1√5]
Topper's Solved these Questions
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Multiple Correct
24 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Assertion- Reasoning
13 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Subjective
15 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE
1 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
Similar Questions
Knowledge Check
A uniform ring of mass m and radius a is placed directly above a uniform sphere of mass M and of equal radius. The centre of the ring is at distance √3a from the centre of the sphere. Find the gravitational force exerted by the sphere on the ring.
AGMm8r2BGMm4r2C√3GMm8r2DGMm8r3√3A point mass m is placed inside a spherical shell of radius R and mass M at a distance R2 form the centre of the shell. The gravitational force exerted by the shell on the point mass is
AGMmR2B2GMmR2CzeroD4MmR2
A uniform ring of mas m and radius a is placed directly above a uniform sphere of mass M and of equal radius. The center of the ring is at a distance √3a from the center of the sphere. Find the gravitational force exerted by the sphere on the ring.
View SolutionA uniform ring of mas m and radius a is placed directly above a uniform sphere of mass M and of equal radius. The centre of the ring is at a distance √3 a from the centre of the sphere. Find the gravitational force exerted by the sphere on the ring.
View SolutionA ring of mass m1 and radius R is fixed in space at some location. An external agent brings a point mass m2 from infinity to centre of the ring. Work done by the external agent will be
View SolutionA ring of mass m and radius R is being rotated about its axis with angular velocity o. If o increases then tension in ring
View SolutionThe moment of inertia of a ring of mass M and radius R about PQ axis will be

View SolutionFind the out the moment of inertia of a ring having uniform mass distribution of mass M and radius R about an axis which is tangent ot the ring and a in the plane of the ring b. perpendicular to the plane of the ring.
View Solution
