A spherical tank of radius is half filled with oil of relative density . If the tank is given a horizontal acceleration of , the maximum pressure on the tank is pascal. Find the value of .
8600
9600
5800
6500
The correct Answer is:B
To solve the problem step by step, we will analyze the situation involving the spherical tank filled with oil and subjected to horizontal acceleration.
Step 1: Identify the parameters
- Radius of the spherical tank, R=1.2m
- Relative density of oil, ρr=0.8
- Acceleration of the tank, a=10m/s2
- Density of water, ρw=1000kg/m3
Step 2: Calculate the density of oil
The density of oil can be calculated using the relative density:
ρ=ρr×ρw=0.8×1000kg/m3=800kg/m3
Step 3: Determine the angle of tilt (θ) due to acceleration
The angle of tilt can be determined using the formula:
tanθ=ag
where g=10m/s2 (acceleration due to gravity).
tanθ=1010=1⟹θ=45∘
Step 4: Calculate the maximum height difference (h_max)
When the tank is accelerated, the oil will tilt, causing a height difference across the tank. The maximum height difference can be calculated as follows:
hmax=Rsinθ
Since θ=45∘, we have:
sin45∘=1√2
Thus,
hmax=R⋅1√2=1.2⋅1√2=1.2√2=1.2√22=0.6√2m
Step 5: Calculate the maximum pressure (P_max)
The pressure at the bottom of the oil column can be calculated using the formula:
P=h⋅ρ⋅g
Substituting hmax:
Pmax=hmax⋅ρ⋅g=(0.6√2)⋅(800)⋅(10)
Calculating this gives:
Pmax=0.6√2⋅8000=4800√2Pa
Step 6: Relate P_max to the given condition
According to the problem, the maximum pressure is given as:
Pmax=√2P
Setting the two expressions for Pmax equal:
4800√2=√2P
Dividing both sides by √2:
P=4800Pa
Final Answer
Thus, the value of P is:
4800
- Radius of the spherical tank, R=1.2m
- Relative density of oil, ρr=0.8
- Acceleration of the tank, a=10m/s2
- Density of water, ρw=1000kg/m3
The density of oil can be calculated using the relative density:
ρ=ρr×ρw=0.8×1000kg/m3=800kg/m3
The angle of tilt can be determined using the formula:
tanθ=ag
where g=10m/s2 (acceleration due to gravity).
tanθ=1010=1⟹θ=45∘
When the tank is accelerated, the oil will tilt, causing a height difference across the tank. The maximum height difference can be calculated as follows:
hmax=Rsinθ
Since θ=45∘, we have:
sin45∘=1√2
Thus,
hmax=R⋅1√2=1.2⋅1√2=1.2√2=1.2√22=0.6√2m
The pressure at the bottom of the oil column can be calculated using the formula:
P=h⋅ρ⋅g
Substituting hmax:
Pmax=hmax⋅ρ⋅g=(0.6√2)⋅(800)⋅(10)
Calculating this gives:
Pmax=0.6√2⋅8000=4800√2Pa
According to the problem, the maximum pressure is given as:
Pmax=√2P
Setting the two expressions for Pmax equal:
4800√2=√2P
Dividing both sides by √2:
P=4800Pa
Thus, the value of P is:
4800
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Single Correct
95 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Multipe Correct
15 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Exercise 4.4
10 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
(a) A spherical tank of 1.2m radius is half filled with oil of relaive density 0.8. If the tank is given a horizontal acceleration of 10m/s2. Calculate the inclination of the oil surface to horizontal and maximum pressure on the tank.
(b) The volume of an air bubble is doubled as it rises from the bottom of a lake to its surface. If the atmospheric pressure is Hm of mercury & the density of mercury is n times that of lake water. Find the depth of the lake.
View SolutionA spherical tank of radius 0.35m is half filled with oil of relative density 0.8. if the tank is moving with a horizontal acceleration of 10ms2. The surface of oil is at rest with respect to sphere. The maximum gauge pressure of oil in the tank is n×103Pa, the value of n is approximately equal to (take g=10ms2
View Solution
Knowledge Check
An open cylindrical tank is of radius 2.8 m and height 3.5 m. What is the capacity of the tank?
A96.24 m3B84.26 m3C86.24 m3D82.64 m3A tank 5 m high is half filled with water and then is filled to the top with oil of density 0.85 g The pressure at the bottom of the tank, due to these liquids is
A1.85g/cm2B89.25g/cm2C462.5g/cm2D500g/cm2A tank 5 m high is half filled with water and then is filled to top with oil of density 0.85g/cm3 The pressure at the bottom of the tank, due to these liquids is
A1.85g/cm2B89.25g/cm2C462.5g/cm2D500g/cm2
A hemispherical tank has inner radius of 2.8m. Find its capacity in litres.
View SolutionAn open cubical tank completely filled with water is kept on a horizontal surface. Its acceleration is then slowly increasesd to 2m/s2 as shown in the figure. The side of the tank is 1m. Find the mass of water that would spill out of the tank.
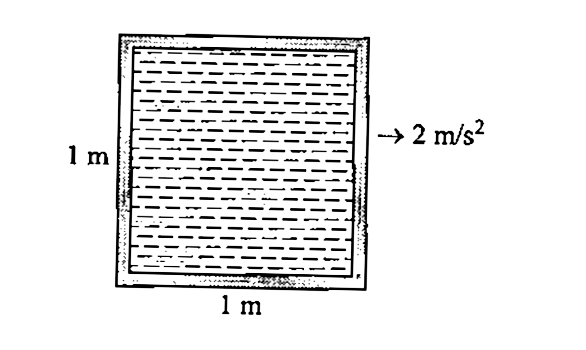
View SolutionAn open cubical tank completely filled with water is kept on a horizontal surface. Its acceleration is then slowly increasesd to 2m/s2 as shown in the figure. The side of the tank is 1m. Find the mass of water that would spill out of the tank.

View Solution_E01_226_Q01.png)
An open retangular tank 5m×4m×3m high containing water upto a height of 2m is accelerated horizontally along the longer side.
(i). Determine the maximum acceleration that can be given without spilling the water.
(ii). Calculate the percentage of water split over if this acceleration of increased by 20%
(iii). If initially the tank is closed at the top and its accelerated horizontally by 9m/s2 find the gauge pressure at the bottom of the front and rear walls of the tank.
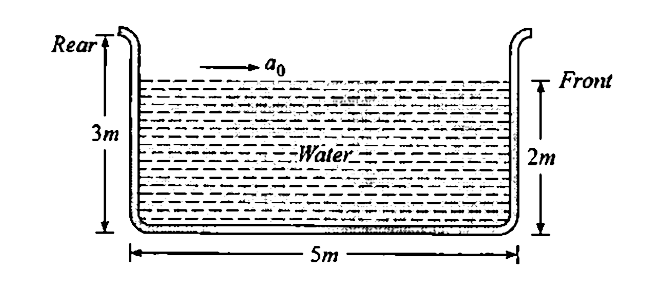
View SolutionAn open reactangular tank with tank with dimensions 5×4m×3m contains water upto a height of 2m is accelerated horizontally along the longer life.
(a) Determine the maximum acceleration that can be given without spilling the water.
(b) If this acceleration is inscreased by 20%. Calculate the percentage of water spilt over.
(c) If initially,the tank is closed at the top and is accelerated horizontally by 9m/s2,find the gauge pressure at the bottom of the front and rear walls of the tank. (g=10m/s2)
View Solution
