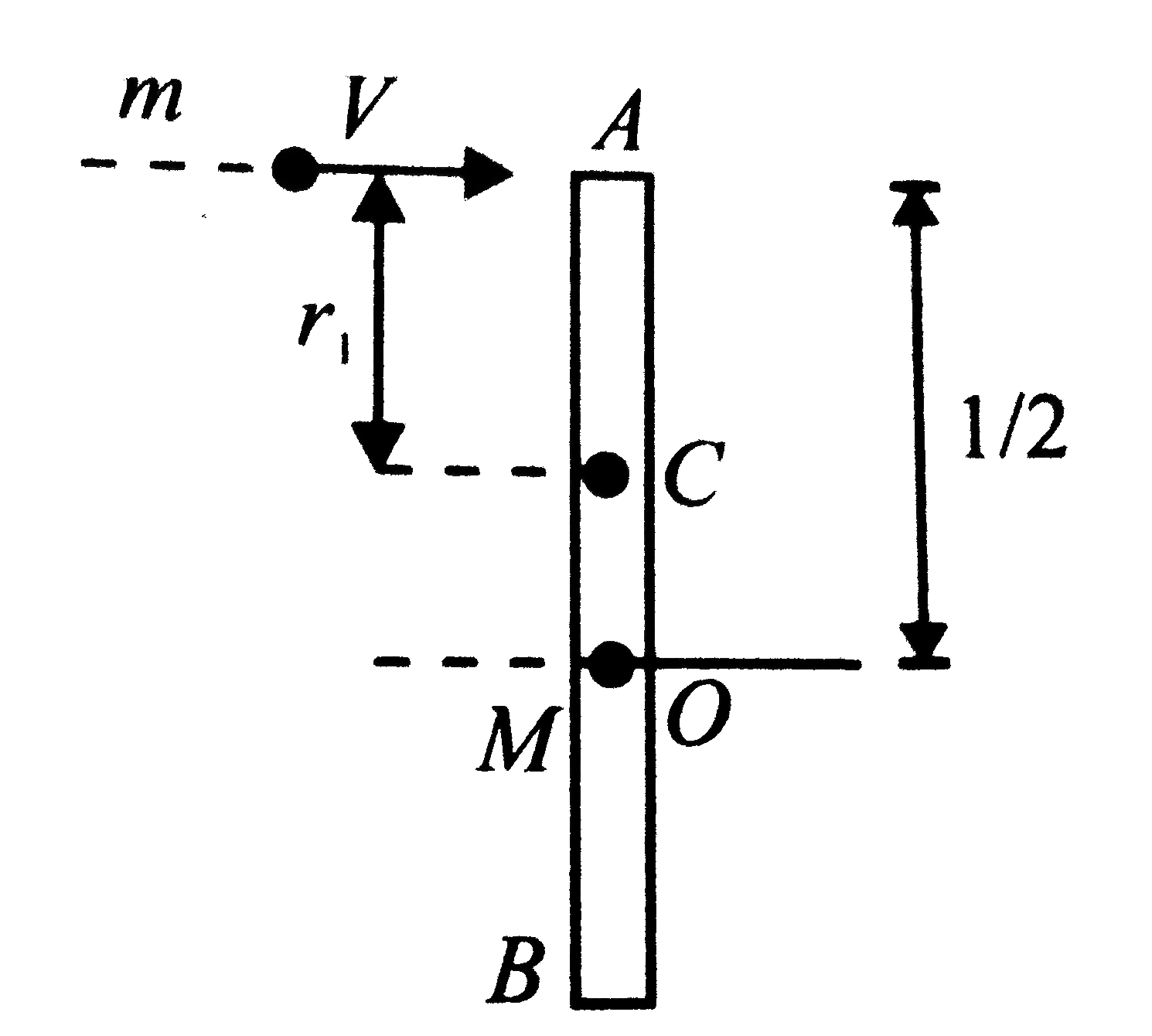
A uniform rod of mass M and length a lies on a smooth horizontal plane. A particle of mass m moving at a speed v perpendicular to the length of the rod strikes it at a distance a4 from the centre and stops after the collision. Find (a). the velocity of the centre of the rod and (b). the angular velocity of the rod abut its centre just after the collision.
(2mv),(M+m),3Mv(M+2m)L
(Mv),(M+m),6Mv(M+4m)L
(2mv),(M+m),3Mv(M+4m)L
(mv),(M+m),6Mv(M+4m)L
The correct Answer is:D
Velocity of the centre of mass of the rod +particle system
VCM=mv(M+m)
→vp,CM=→vp−→vc
vc=mvM+m and vp=v
→vp,CM=MvM+m
→vrod,CM=→vrod−→vCM=0−mvM+m
vrod,CM=−mvM+m
Location of the centre of mass of the system rod + particle from A
r1=m×0+Ml/2(m+M)
=Ml2(m+M)
Angular momentum of the particle just before collision about C,
Lp,c=mvp,cr1
=M2mv2(M+m)2
Angular momentum of the rod about the centre of mass of system C,
Lrod,CM=Mvrod,CM(l2−r1)
=M(mvM+m)(ml2(M+m))
=Mm2vl2(M+m)2
Moment of inertia about the vertcal axis passing through C,
Ic,rod=I0=M(l2−r1)2
Ic,rod=Ml212+m(ml2(M+m))2
Ic,particle=mr21=m(Ml2(M+m))2
Ic=Ml212+M(ml2(M+m))2+m(Ml2(M+m))2
Ic=M(M+4m)12(M+m)L2
As no external forces are acting on the system rod + particle, hence the velocity of the centre of mass of the system will remain constant.
vCM=mvM+m
For angular velocity about C
mvr1=Icω
ω=mvr1Ic=mv(ml2(M+m))(M(M+4m)L212(m+m))
=6mv(M+3m)L
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Integer7videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Fill In The Blanks7videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Multiple Correct26videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer11videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
Similar Questions
A uniform rod of mass 2kg and length 1m lies on a smooth horizontal plane. A particle of mass 1 kg moving at a speed of 2ms−1 perpendicular to the length of the rod strikes it at a distance 14 m from the centre and stops . Find the angular velocity of the rod about its centre just after the collision (in rad/s)
View SolutionA uniform rod of length L lies on a smooth horizontal table. The rod has a mass M. A particle of mass m moving with speed v strikes the rod perpendicularly at one of the ends of the rod sticks to it after collision.
Find the velocity of the particle with respect to C before the collisionView SolutionA uniform rod of mass m and length L is at rest on a smooth horizontal surface. A ball of mass m, moving with velocity v0, hits the rod perpendicularly at its one end and sticks to it. The angular velocity of rod after collision is
 View Solution
View SolutionA uniform rod of mass m, length l rests on a smooth horizontal surface. Rod is given a sharp horizontal impulse p perpendicular to the rod at a distance l/4 from the centre. The angular velocity of the rod will be
View SolutionA rod of mass 4m and length L is hinged at the mid point. A ball of mass 'm' moving with speed V in the plane of rod, strikes at the end at an angle of 45∘ and sticks to it. The angular velocity of system after collision is-
View SolutionA uniform rod of length 8 a and mass 6 m lies on a smooth horizontal surface. Two point masses m and 2 m moving in the same plane with speed 2 v and v respectively strike the rod perpendicular at distances a and 2a from the mid point of the rod in the opposite directions and stick to the rod. The angular velocity of the system immediately after the collision is
View SolutionA uniform rod AB of mass m and length l is at rest on a smooth horizontal surface. An impulse J is applied to the end B, perpendicular to the rod in the horizontal direction. Speed of particlem P at a distance l6 from the centre towards A of the rod after time t=πml12J is.
View Solution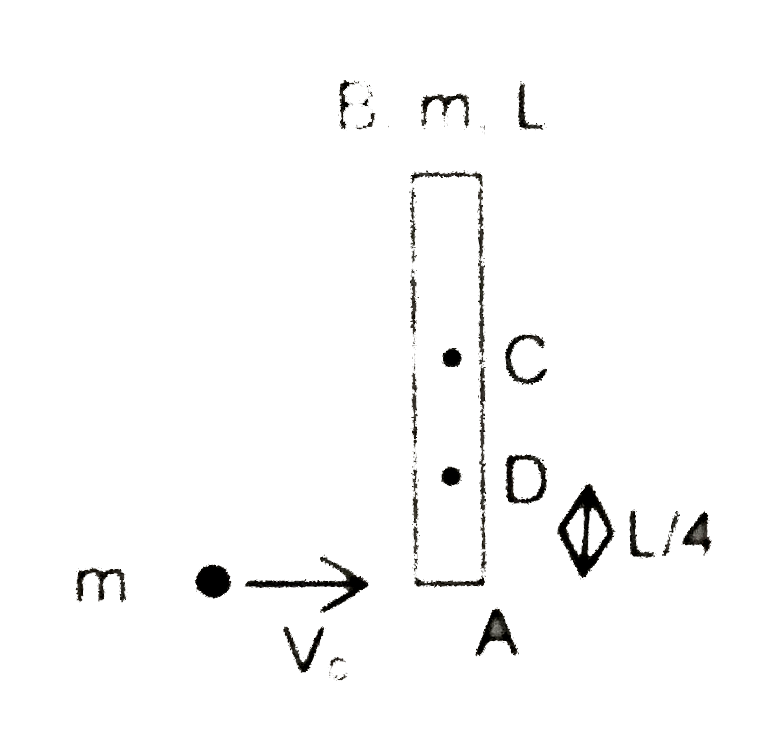
A thin, uniform rod of mass M and length L is at rest on a smooth horizontal surface. A particle of same mass M collides with the rod at one end perpendicular to its length with velocity V∘ and sticks to it. C is the middle point of the rod and D is a point at a distance of L/4 from end A. the time taken by the rod turn through 90∘ after collisions.View SolutionA uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
View Solution
A uniform rod of mass 3 m and length l is lying on a smooth horizontal surface. Two particle of mass m and 2 m moving with speed 2v0 and v0 in the opposite directly hit the rod perpendicularly at a distance l3 and l6 from the midpoint O of the rod ad stick to it after collision. if the loss in kinetic energy of the system due to collision is n5mv20 find the value of n.View Solution
