Find the electric field at the centre of a uniformly charged semicircular ring of radius R. Linear charge density is λ
The correct Answer is:A, B, D
To find the electric field at the center of a uniformly charged semicircular ring of radius R with linear charge density λ, we can follow these steps:
Step 1: Define the Charge Element
Consider a small charge element dq on the semicircular ring. The linear charge density λ is defined as charge per unit length. Therefore, for an infinitesimal arc length dl, we have:
dq=λdl
For a semicircular ring, the arc length dl can be expressed in terms of the angle θ as:
dl=Rdθ
Thus, we can write:
dq=λRdθ
Step 2: Determine the Electric Field Contribution
The electric field dE due to the charge element dq at the center of the semicircle can be expressed using Coulomb's law:
dE=kdqR2
Substituting dq:
dE=kλRdθR2=kλdθR
where k is Coulomb's constant.
Step 3: Resolve the Electric Field Components
The electric field dE has both x and y components. Due to symmetry, the x-components from opposite sides will cancel out. Therefore, we only need to consider the y-component:
dEy=dEcos(θ)=kλdθRcos(θ)
Step 4: Integrate to Find the Total Electric Field
To find the total electric field Ey at the center, we integrate dEy from θ=0 to θ=π:
Ey=∫π0dEy=∫π0kλRcos(θ)dθ
Calculating the integral:
Ey=kλR∫π0cos(θ)dθ
The integral of cos(θ) from 0 to π is:
∫π0cos(θ)dθ=(sin(θ))π0=sin(π)−sin(0)=0−0=0
However, we need to consider the contributions from both halves of the semicircle, which gives us:
Ey=2⋅kλR∫π20cos(θ)dθ
Calculating this integral:
∫π20cos(θ)dθ=(sin(θ))π20=sin(π2)−sin(0)=1−0=1
Thus:
Ey=2⋅kλR⋅1=2kλR
Final Result
The electric field at the center of the uniformly charged semicircular ring is:
E=2kλR
Consider a small charge element dq on the semicircular ring. The linear charge density λ is defined as charge per unit length. Therefore, for an infinitesimal arc length dl, we have:
dq=λdl
For a semicircular ring, the arc length dl can be expressed in terms of the angle θ as:
dl=Rdθ
Thus, we can write:
dq=λRdθ
The electric field dE due to the charge element dq at the center of the semicircle can be expressed using Coulomb's law:
dE=kdqR2
Substituting dq:
dE=kλRdθR2=kλdθR
where k is Coulomb's constant.
The electric field dE has both x and y components. Due to symmetry, the x-components from opposite sides will cancel out. Therefore, we only need to consider the y-component:
dEy=dEcos(θ)=kλdθRcos(θ)
To find the total electric field Ey at the center, we integrate dEy from θ=0 to θ=π:
Ey=∫π0dEy=∫π0kλRcos(θ)dθ
Calculating the integral:
Ey=kλR∫π0cos(θ)dθ
The integral of cos(θ) from 0 to π is:
∫π0cos(θ)dθ=(sin(θ))π0=sin(π)−sin(0)=0−0=0
However, we need to consider the contributions from both halves of the semicircle, which gives us:
Ey=2⋅kλR∫π20cos(θ)dθ
Calculating this integral:
∫π20cos(θ)dθ=(sin(θ))π20=sin(π2)−sin(0)=1−0=1
Thus:
Ey=2⋅kλR⋅1=2kλR
The electric field at the center of the uniformly charged semicircular ring is:
E=2kλR
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Subjective
15 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - SUBJECTIVE_TYPE
6 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Assertion And Reason
19 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
Electric field at centre of a uniforly charged semicirlce of radius a is
View SolutionFind the centre of mass of a uniform semicircular ring of radius R and mass M.
View SolutionFind the electric field at centre of semicircular ring shown in figure .
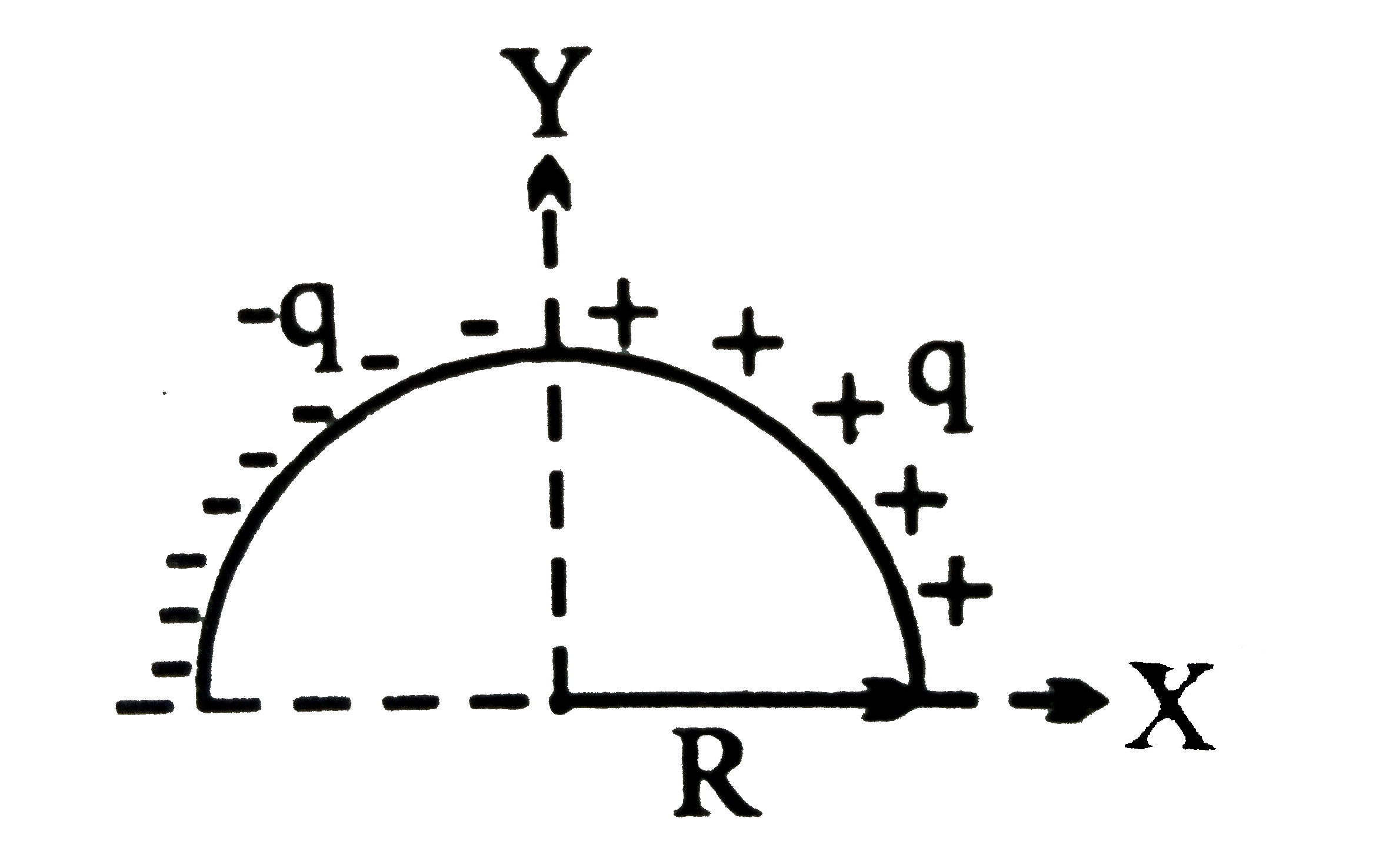 .
.
View SolutionElectric field at centre O of semicircule of radius 'a' having linear charge density λ given is given by

View SolutionElectric field at the centre of uniformly charge hemispherical shell of surface charge density σ is σnε0 then find the value of n.
View SolutionThe electric field at 2R from the centre of a uniformly charged non - conducting sphere of rarius R is E. The electric field at a distance R2 from the centre will be
View Solution(a) Using Gauss's law, derive an expression for the electric field intensity at any point outside a uniformly charged thin spherical shell of radius R and charge density σC/m2. Draw the field lines when the charge density of the sphere is (i) positive, (ii) negative.
(b) A uniformly charged conducting sphere of 2.5 m in diameter has a surface charge density of 100 μC/m2. Calculate the
(i) charge on the sphere
(ii) total electric flux passing through the sphere.
View SolutionElectric field at a point of distance r from a uniformly charged wire of infinite length having linear charge density λ is directly proportional to
View SolutionFind the electric field due to an infinitely long cylindrical charge distribution of radius R and having linear charge density λ at a distance half of the radius from its axis.
View SolutionA point charge q0 is placed at the centre of uniformly charges ring of total charge Q and radius R. If the point charge is slightly displaced with negligible force along axis of the ring then find out its speed when it reaches a large distance.
View Solution
