Ideal gas undergoes an adiabatic change in its state from (P1V1,T1) to(,P2,V2T2). The work done (W) in the process is (n = number of moles, Cp and Cv are molar specific heats of gas)
W=n(T1−T2)CP
W=n(T1−T2)Cv
W=n(T1+T2)Cp
W=n(T1+T2)CV
The correct Answer is:B
To find the work done (W) during an adiabatic process for an ideal gas transitioning from state (P1,V1,T1) to (P2,V2,T2), we can follow these steps:
1. Understand the Adiabatic Process: In an adiabatic process, there is no heat exchange with the surroundings. This means that the change in internal energy (ΔU) is equal to the work done on or by the system.
ΔU=W
2. Use the First Law of Thermodynamics: The first law of thermodynamics states that:
ΔU=Q−W
Since Q=0 for an adiabatic process, we have:
ΔU=−W⇒W=−ΔU
3. Calculate the Change in Internal Energy: The change in internal energy for an ideal gas can be expressed as:
ΔU=nCv(T2−T1)
where n is the number of moles, and Cv is the molar specific heat at constant volume.
4. Substituting into the Work Done Equation: Substitute the expression for ΔU into the work done equation:
W=−nCv(T2−T1)
5. Rearranging the Equation: This can be rearranged to show that:
W=nCv(T1−T2)
6. Final Expression: The work done by the gas during the adiabatic process can thus be expressed as:
W=nCv(T1−T2)
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - THE FIRST LAW OF THERMODYNAMICSEXERCISE - PRACTICE QUESTIONS ( MORE THAN ONE CORRECT CHOICE TYPE )9videos
THE FIRST LAW OF THERMODYNAMICS
- BOOK - RESNICK AND HALLIDAYCHAPTER - THE FIRST LAW OF THERMODYNAMICSEXERCISE - PRACTICE QUESTIONS ( LINKED COMPREHENSION )15videos
THE FIRST LAW OF THERMODYNAMICS
- BOOK - RESNICK AND HALLIDAYCHAPTER - THE FIRST LAW OF THERMODYNAMICSEXERCISE - PROBLEMS41videos
THE FIRST LAW OF THERMODYNAMICS
- BOOK - RESNICK AND HALLIDAYCHAPTER - TEMPERATURE, ZEROTH LAW OF THERMODYNAMICS AND THERMAL EXPANSION EXERCISE - PRACTICE QUETIONS (Integer Type)4videos
TEMPERATURE, ZEROTH LAW OF THERMODYNAMICS AND THERMAL EXPANSION
- BOOK - RESNICK AND HALLIDAYCHAPTER - THE KINETIC THEORY OF GASESEXERCISE - PRACTICE QUESTIONS72videos
THE KINETIC THEORY OF GASES
Similar Questions
Assertion: When ideal gas expand from P1,V1,T1, to P2,V2,T2 two steps, and work done is high in which number of steps are high
Reason: Work is path functionView SolutionOne mole of a gas in the state A(P1,V1,T1) is subjected to adiabatic expansion to attain state B(P2,V2,T2). The work done by the gas is:
View Solution
Knowledge Check
- Question 1 - Select One
The work done in changing the state from (P0,V0) to (P1,V1) in the following system is :
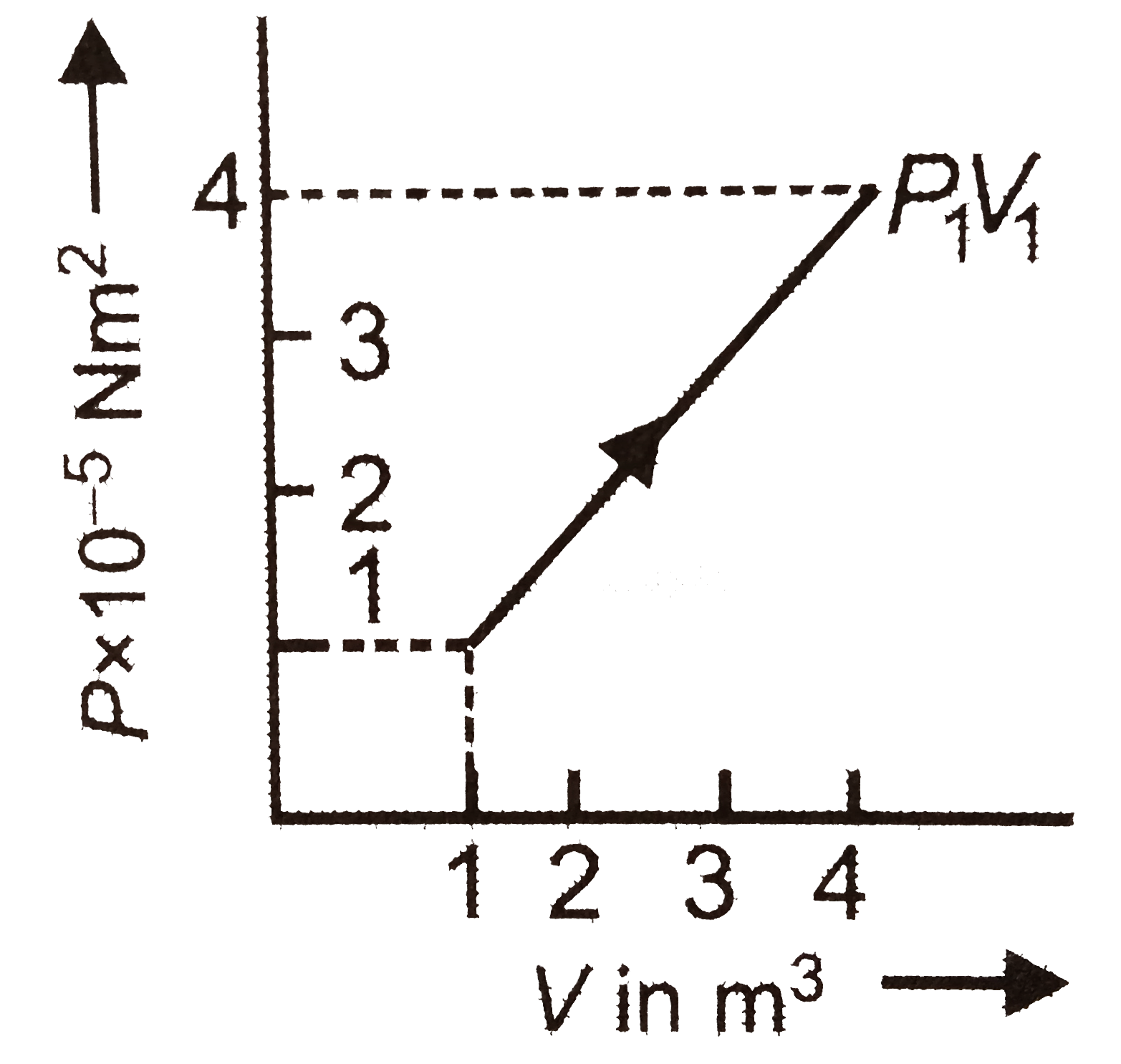 A−7.5×10−5JB−7.5×105JC−7.5×105ergD−7.5×10−5 erg
A−7.5×10−5JB−7.5×105JC−7.5×105ergD−7.5×10−5 erg - Question 2 - Select One
A gas expands under constant pressure P from volume V1 toV2The work done by the gas is
AP(V2−V1)BP(V1−V2)CP(Vλ1−Vλ2)DPV1V2V2−V1 - Question 3 - Select One
In an adiabatic process, the state of a gas is changed from P1,V1,T1,toP2,V2,T2. Which of the following relation is correct
AT1Vλ−11=T2Tλ−12BP1Vλ−11=P2Vλ−12CT1Pλ1=T2Pλ2DT1Vλ1=T2Vλ2
One mole of hydrogen, assumed to be ideal, is adiabatically expanded from its initial state (P1,V1,T1) to the final state (P2,V2,T2). The decrease in the internal energy of the gas during this process will be given by
View SolutionAn ideal gas follows a process described by PV2=C from (P1,V1,T1) to (P2,V2,T2) (C is a constant). Then
View SolutionA gaseous system changes from state A(P1,V1,T1) to B(P2,V2,T2), B to C (P3,V3T3) and finally from C to A. The whole process may be called
View SolutionA gaseous system changes from state A(P1,V1,T1) to B(P2,V2,T2) to C(P3,V3,T3) and finally from C to A. The whole process may be called :
View SolutionAn ideal gas is transformed from state A(P1,V1) to the state B(P2,V2) through path AB. In this process the work done by the gas is
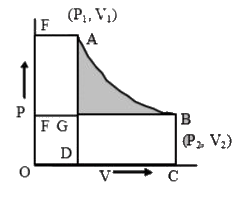 View Solution
View Solution

