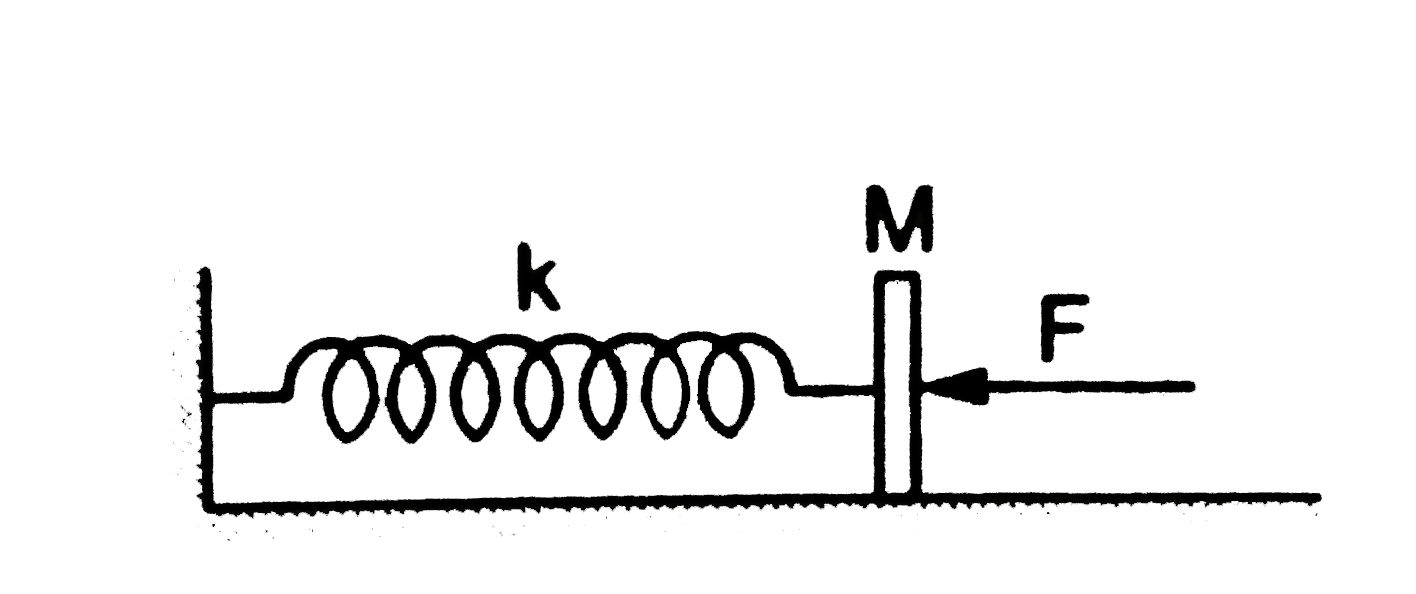
In figue k=100Nm−1M=1kgandF=10N. a. Find the compression of the spring in the equilibrium position. b. A sharp blow by some xternal agent imparts a speed of 2ms−1 to the block towards left. Find the sum of the potential energy of the spring and the kinetic energy of the block at ths instant. c. find the time period of the resulting simple harmonic motion. d. Find the ampitude. e. Write the potential energy of the spring when the block is at the left extreme. f. Write teh potential energy of the spring when teh block is at the right extreme.
The anwer of b, e, and f are different. Explain why this does not violate the principle of conservation of energy.
The correct Answer is:A, B, C, D
Given k=100BNm,m=1kgandF=10N<br>a.Intheequilibriumposition<br>Compression =δ=Fk=10100
=0.1m=10cm
b. The below imparts a speed of 2 m/s to the block towards left.
∴+P.E.+K.E.=12kδ2+12Mv2
=12x100×(0.1)2+12x1×4
=0.5+2=2.5J
c.Timeperiod2pisqrt(M/k)<br>=2pi sqrt(M/100)=pi/5 sec<br>d.Lettheamplitudebexwhighmeansthedistasncebetwenthemeanpositionandtheextremeposition.<br>So∈theextremeposition,compressionofthespr∈gis(x-K)<br>Since∈SHMthe→tle≠rgyrema∈sconstant,<br>1/2k(x+delta)^2=1/2kdelta^2+1/2mv^2+Fx<br>=2.5+10x
[because 1/2kdelta^2+1/2mv^2=2.5]<br>so,50(x+0.1)^2=2.5+10x<br>:. 50x^2+0.5+10x=2.5+10x<br>=:. 50x^2=2<br>rarr x^2=2/50=4/100<br>rarr x=2/50x=20m<br>e.potentiale≠rgyatthe≤ftextremeisgivenby<br>P.E. =1/2k(x+delta)^2<br>=1/2x100xx(0.1+0.2)^2<br>=50xx(0.09)=4.5J<br>f.Potentiale≠rgyattherightextremeisgivenby<br>P.E. 1/2k(x+delta)^2-F(2x)<br>[2x=distancebetweentwoextreme)
=4.5−10(0.4)=0.5J
The different values is b, e, and f do not violate law of conservastion of energy as the work is done by the exterN/Al force 10 N.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Objective-2
2 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises
86 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMACHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Exercises
32 Videos
Similar Questions
In figure, k=100N/m,M=1kg and F=10N
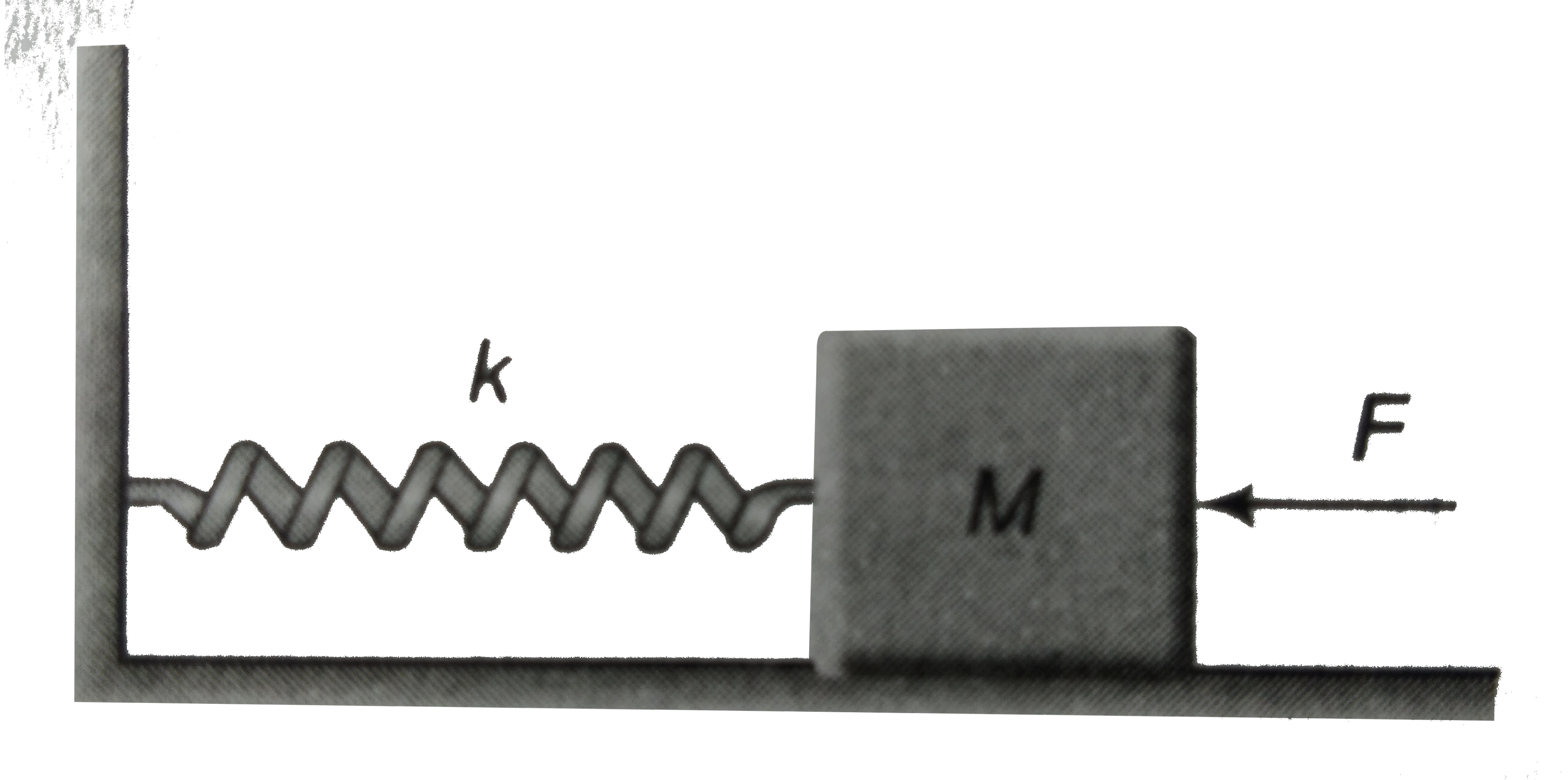
(a) Find the compression of the spring in the equilibrium position
(b) A sharp blow by some external agent imparts a speed of 2m/s to the block towards left. Find the sum of the potential energy of the spring and the kinetic energy of the block at this instant.
(c) Find the time period of the resulting simple harmonic motion.
(d) Find the amplitude.
(e) Write the potential energy of the spring when the block is at the left estreme.
(f) Write the potential energy of the spring when the block is at the right extreme.
The answers of (b), (e) and (f) are different. Explain why this does not violate the principle of conservation of energy ?
View SolutionIn figure, k=100N/m,M=1kg and F=10N

(a) Find the compression of the spring in the equilibrium position
(b) A sharp blow by some external agent imparts a speed of 2m/s to the block towards left. Find the sum of the potential energy of the spring and the kinetic energy of the block at this instant.
(c) Find the time period of the resulting simple harmonic motion.
(d) Find the amplitude.
(e) Write the potential energy of the spring when the block is at the left estreme.
(f) Write the potential energy of the spring when the block is at the right extreme.
The answers of (b), (e) and (f) are different. Explain why this does not violate the principle of conservation of energy ?
View SolutionFind the elastic potential energy stored in each spring shown in figure, when the block is in equilibrium. Also find the time period of vertical oscillation of the block.

View SolutionFind the elastic potential energy stored in each spring shown in figure, when the block is in equilibrium. Also find the time period of vertical oscillation of the block.

View SolutionA block of mass 0.5 kg hanging from a vertical spring executes simple harmonic motion of amplitude 0.1 m and time period 0.314s. Find the maximum fore exerted by the spring on the blockl.
View SolutionA block of mass 0.5 kg hanging from a vertical spring executes simple harmonic motion of amplitude 0.1 m and time period 0.314s. Find the maximum fore exerted by the spring on the blockl.
View SolutionThe spring shown in figure is unstretched when a man starts pulling on the cord. The mass of the block is M. If the man exerts a constant force F, find
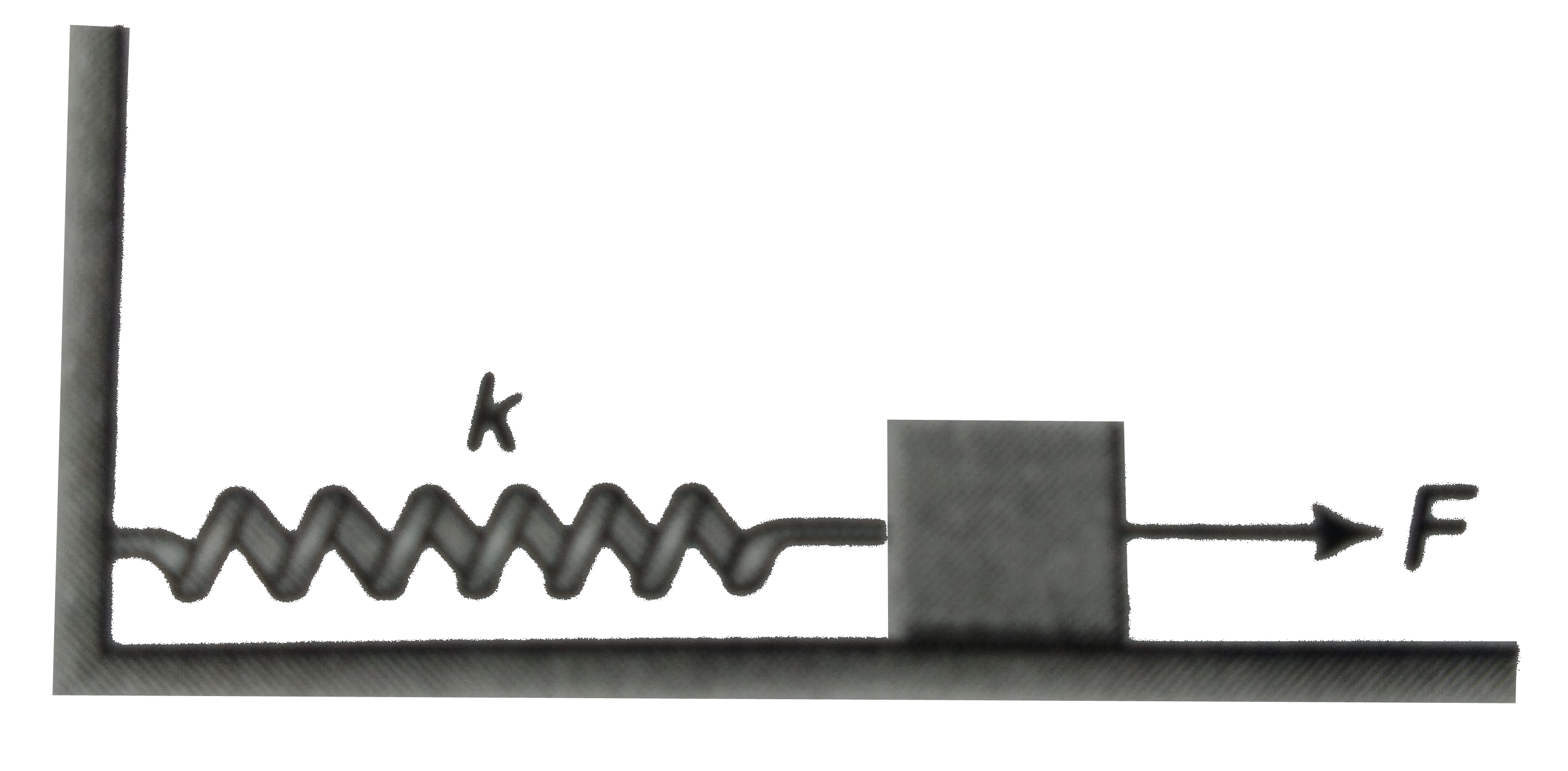
(a) the amplitude and the time period of the motion of the block,
(b) the energy stored in the spring when the block passes through the equilibrium position and
(c) the kinetic energy of the block at this position.
View SolutionThe spring shown in figure is unstretched when a man starts pulling on the cord. The mass of the block is M. If the man exerts a constant force F, find

(a) the amplitude and the time period of the motion of the block,
(b) the energy stored in the spring when the block passes through the equilibrium position and
(c) the kinetic energy of the block at this position.
View SolutionThe spring shown in figure is unstretched when a man starts pulling on the cord. The mass of the block is M. If the man exerts a constant force F, find

(a) the amplitude and the time period of the motion of the block,
(b) the energy stored in the spring when the block passes through the equilibrium position and
(c) the kinetic energy of the block at this position.
View SolutionA oscillator of mass 10kg has a velocity of 5 ms−1 after 1 second of its crossing the mean position. If the time period is 5s. Find the potential energy and kinetic energy at that instant.
View Solution
