Initially, the capacitor is charged to a potential of 5V and then connected to position 1 with the shown polarity for 1s. After 1s it is connected across the inductor at position 2
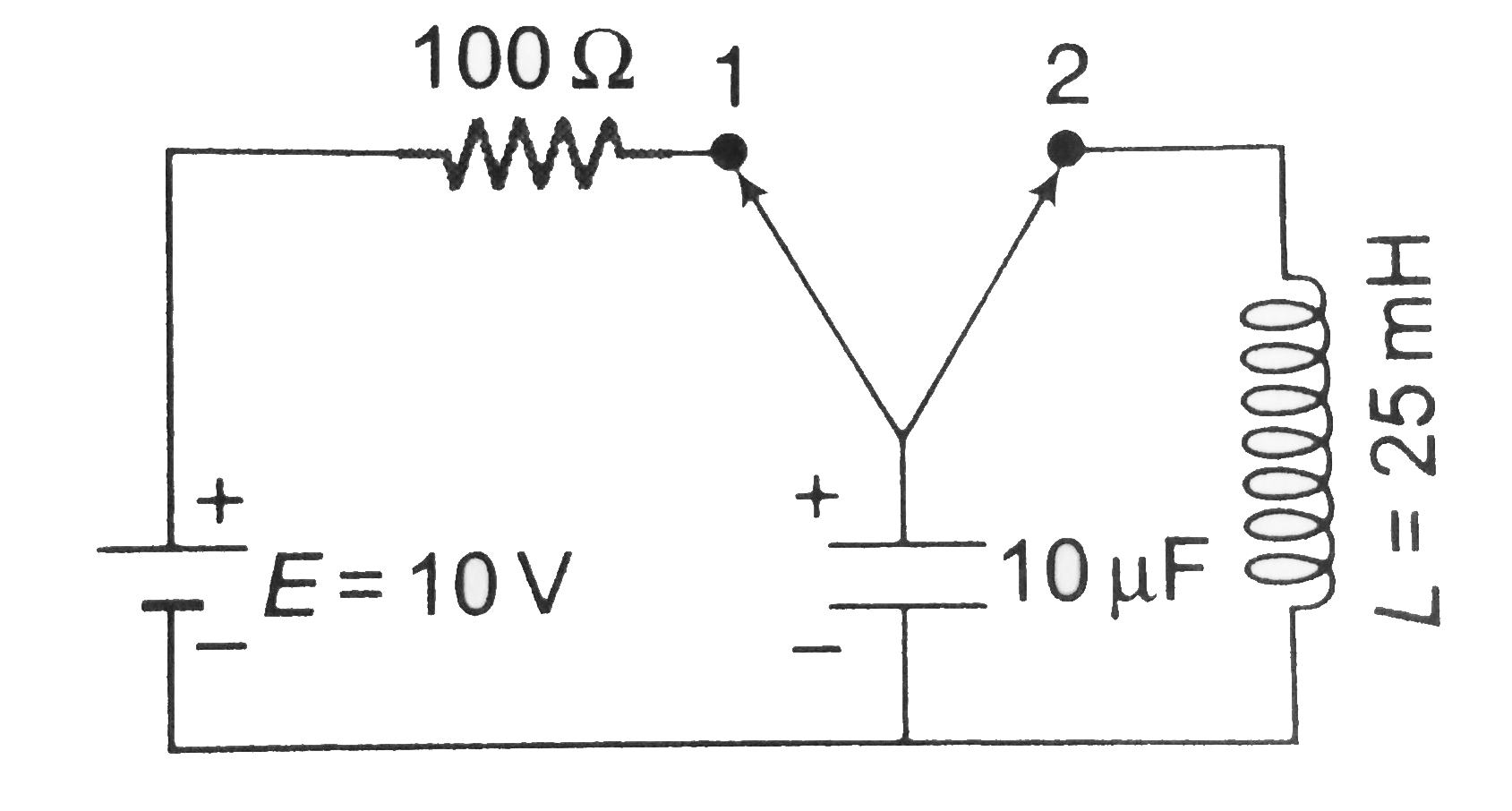
(a) Find the potential across the capacitor after 1s of its connection to position 1.
(b) Find the maximum current flowing in the L−C circuit when capacitor is connected across the inductor. Also, find the frequency of LC oscillations.
The correct Answer is:A
In the capacitor,
qi=CVi=(10×10−3)(5)=0.05C
qf=CVf=(10×10−3)(10)=0.1C
∴ Charge in capacitor will increase from 0.05C to 0.1C exponentialy.
Time constant for this increase would be
τL=CR=1s
∴ Charge at time t will be
q=0.05+(0.1−0.05)(1−e−t/τC)
=0.1−0.05e−t/τC
b. At t=1s,q=0.1−0.05e−1=0.0816C
V=qC=0.081610×10−3=8.16V
b. This charge 0.0816C is also the maximum charge q0 of L−C oscillations.
From energy conservation equation,
12q20C=12Li20 we have,
i0=q0√LC=0.0816√25×10−3×10×10−3
=5.16A
Further, ω=1√LCor f=ω2π=12π√LC
=12π√25×10−3×10×10−3
=10Hz
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Check point
60 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Taking it together
119 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - SUBJECTIVE TYPE
1 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEYCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery
97 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEYCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
Similar Questions
A capacitor is charged to a potential difference of 100V and then it is connected across a resistor.After one second,the potential drop across the plates of the capacitor becomes 80V .Then after 2sec the potential difference
View SolutionA capacitor of capacity 2μF is changed to a potential different of 12V . It is then connected across an inductor of inductance 0.6mH What is the current in the circuit at a time when the potential difference across the capacitor is 6.0V ?
View Solution
Knowledge Check
How many 1μF capacitors must be connected in parallel to store a charge of 1 C with a potential of 110 V across the capacitors?
A990B900C9090D909A capacitor is charged to a potential difference of 100 V and is then connected across a resistor. The potential difference across the capacitor decays exponentially with respect to time. After 1 sec, the P.D. between the plates of the capacitor is 80 V. what will be the potential difference between the plates after 2 sec ?
AAfter 2ms from start, the potential difference across the resistor is 64 VBAfter 2ms from start, the potential difference across the capacitor is 64 VCAfter 2ms from start , the potential difference across the resistor is 60VDAfter 2ms from start , the potential difference across the capacitor is 60 VA capacitor of capacitance C0 is charged to a potential V0 and is connected with another capacitor of capacitance C as Shown . After closing the switch S, the common potential across the two capacitors becomes V . The capacitance C is given by
 AC0(V0−V)V0BC0(V−V0)V0CC0(V+V0)VDC0(V0−V)V
AC0(V0−V)V0BC0(V−V0)V0CC0(V+V0)VDC0(V0−V)V
A 10μF capacitor is charged to 15V. It is next connected in seties with an uncharged 5μf capacitor. The series cambination is finally connected across a 30V battery, as shown in. Find the new potential differnce across the 5μF and 10μF capacitors.
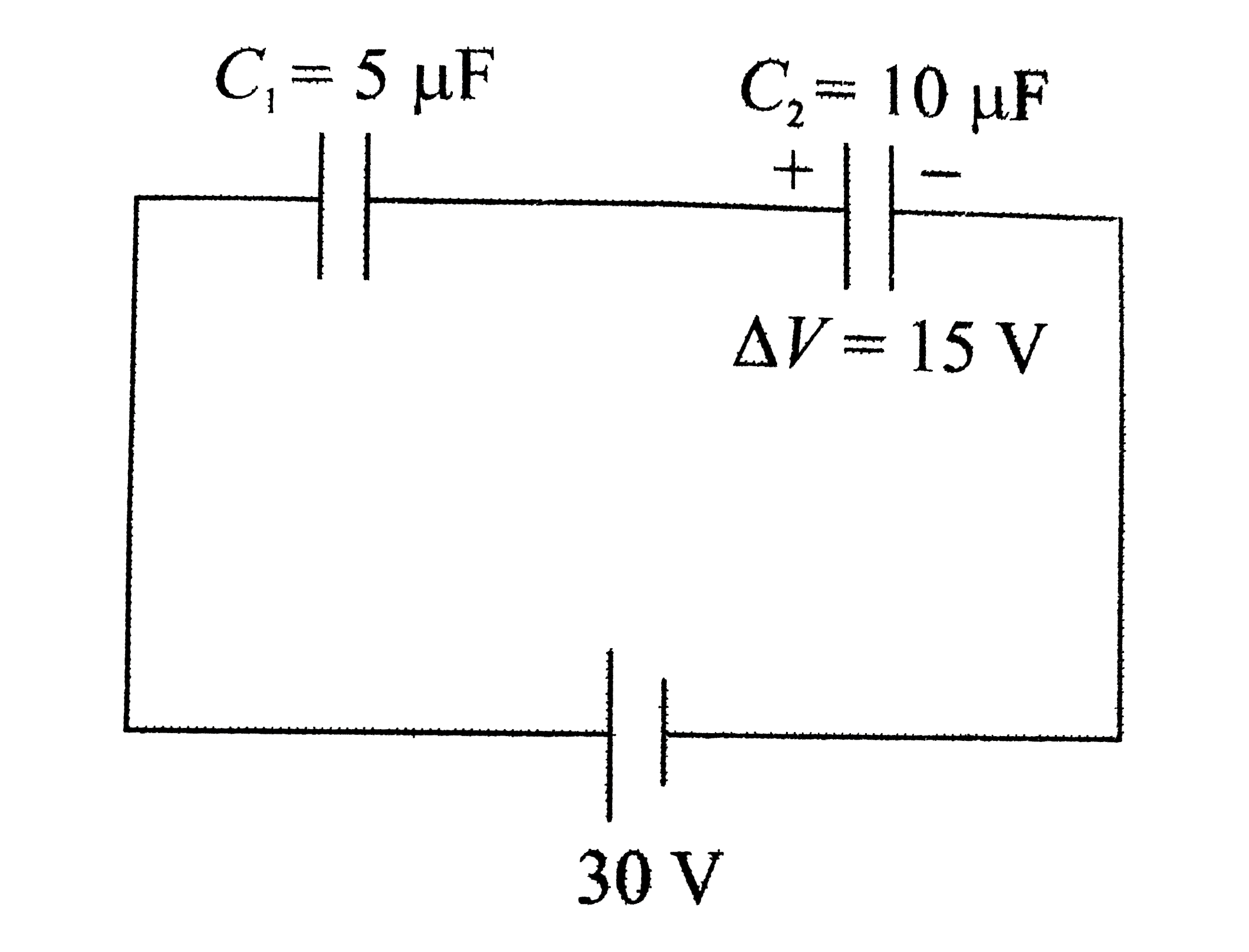
View SolutionA capacitor of capacity 2μF is charged to a potential difference of 12V. It is then connected across an inductor of inductance 6μH. What is the current (in A) in the circuit at a time when the potential difference across the capacitor is 6.0V?
View SolutionA capacitor is connected to a 12 V battery through a resistance of (10Ω).It is found that the potential difference across the capacitor rises to 4.0 V in 1(mu)s. find the capacitance of the capacitor.
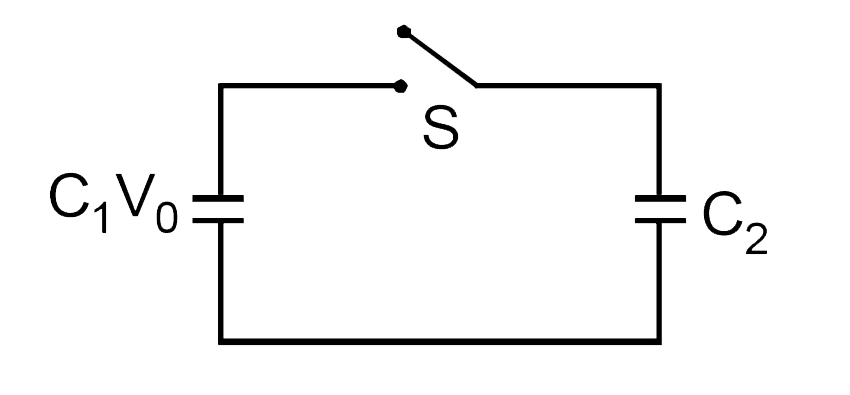
View SolutionA capacitor of capacity C1 is charged to the potential of V0. On disconnecting with the battery, it is connected with a capacitor of capacity C2 as shown in the adjoining figure. The ratio of energies before and after the connection of switch S will be
View SolutionA capacitor of capacity 2μF is charged to a potential difference of 12V. It is then connected across an inductor of inductance 0.2mH. At an instant when potential difference across the capacitor is 6v
View Solution
