Question

Pushing force making an angle $\theta$ to the horizontal is applied on a block of weight W placed on a horizontal table . If the angle of friction is $\varphi$ , magnitude of force required to move the body is equal to :

Options

A. `( w cos theta)/(cos (theta-phi))`
B. `( w sin theta)/(cos (theta+phi))`
C. `( w tan phi)/(sin (theta+phi))`
D. `( w sin phi)/(tan (theta+phi))`

Answer

B

Explanation

(b) `N=w=F sin theta`
`therefore" " f_("max")=muN=(tan phi)(w+F sin theta)`

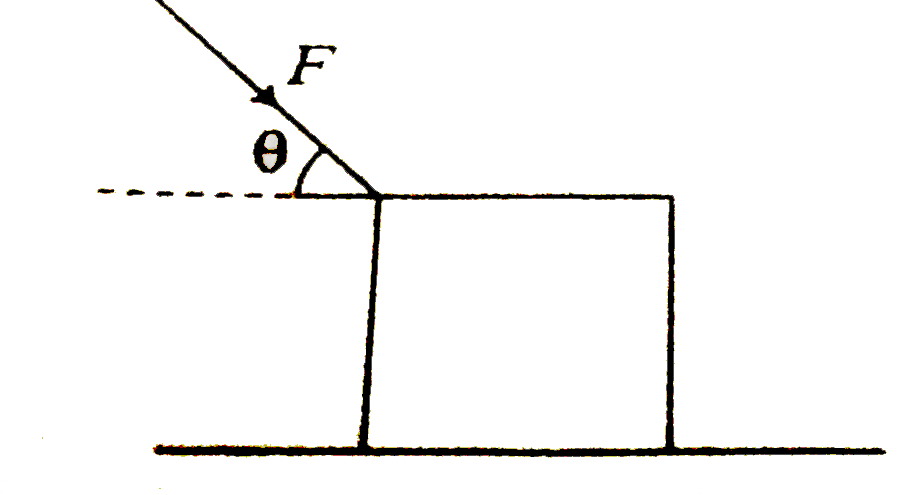
To move the body,
`F cos theta=f_("max")=(tanphi)(w+F sin theta)`
Solving this equation we get,
`F=(w sin theta)/(cos (theta+phi))`