Small sphere of mass m and radius r is kept on fixed inclined rough surface. Surface makes an angle with the horizontal. Initially centre of sphere is at a height h above the horizontal floor. Coefficient of friction between the sphere and surface is u. Sphere is released from the state of rest.
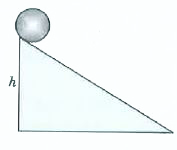
Assume that friction is sufficiet enough to provide pure rolling. Speed of the sphere as it reaches the bottom is
√2gh
√2gh5
√10gh7
√5gh7
The correct Answer is:C
If it is a case of pure rolling thon point of contact remains at rest and thus work done by friction remains zero and total mechanical energy of the system remains conserved. Let speed guined by sphere is u when it reaches the bottom then its kinetic energy can be written as follows:
K.E.=12Icmω2+12mv2
=12(25mr2)(vr)2+12mv2=710mv2
According to conservation of mechanical energy we can write the following:
Gain in KE,Loss of gravitational potential energy
⇒710mv2=mgh⇒v=√10gh7
Hence option (c) is correct
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE (OBJECTIVE TYPE QUESTIONS (ASSERTION REASON TYPE QUESTIONS) )
10 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE ( OBJECTIVE TYPE QUESTIONS (MATCHING TYPE QUESTIONS) )
3 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE ( OBJECTIVE TYPE QUESTIONS (JEE (Main) & Other State Boards for Engineering Entrance) )
48 Videos
- PHYSICAL WORLD BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)
6 Videos
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
A unifrom sphere of mass m and radius R is placed on a rough horizontal surface (figure) .the sphere is stuuck horizontally at a height h from the floor . Match the following
View SolutionA uniform sphere of mass m and radius R is placed on a rough horizontal surface. The sphere is struck horizontally at a heighth from the floor. Match the following:
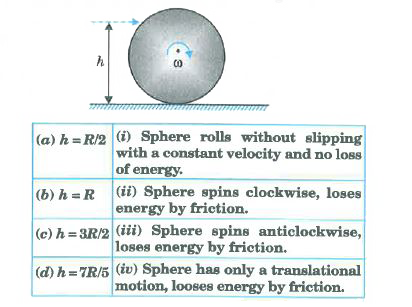
View Solution
Knowledge Check
Small sphere of mass m and radius r is kept on fixed inclined rough surface. Surface makes an angle with the horizontal. Initially centre of sphere is at a height h above the horizontal floor. Coefficient of friction between the sphere and surface is u. Sphere is released from the state of rest.
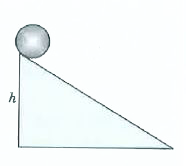
Assume that friction is sufficient engough for pure rolling. Accleeration of the shpere isAgsinθB5gsinθ7C7gsinθ5D2gsinθ5Small sphere of mass m and radius r is kept on fixed inclined rough surface. Surface makes an angle with the horizontal. Initially centre of sphere is at a height h above the horizontal floor. Coefficient of friction between the sphere and surface is u. Sphere is released from the state of rest.
Assume friction is not sufficient to provided pure rolling. Acceleration of the sphere isAgsinθ−μgcosθBgsinθCμgcosθD57gsinθ−μgcosθA solid sphere of mass M and radius R is pulled horizontally on a rough surface as shown in Fig. Choose the incorrect alternatives.
 AThe magnitude of the frictional force isF/3.BThe frictional force on the sphere acts forward.CThe acceleration of the centre of mass is 2F/3MDThe acceleration of the centre of mass is F/M.
AThe magnitude of the frictional force isF/3.BThe frictional force on the sphere acts forward.CThe acceleration of the centre of mass is 2F/3MDThe acceleration of the centre of mass is F/M.
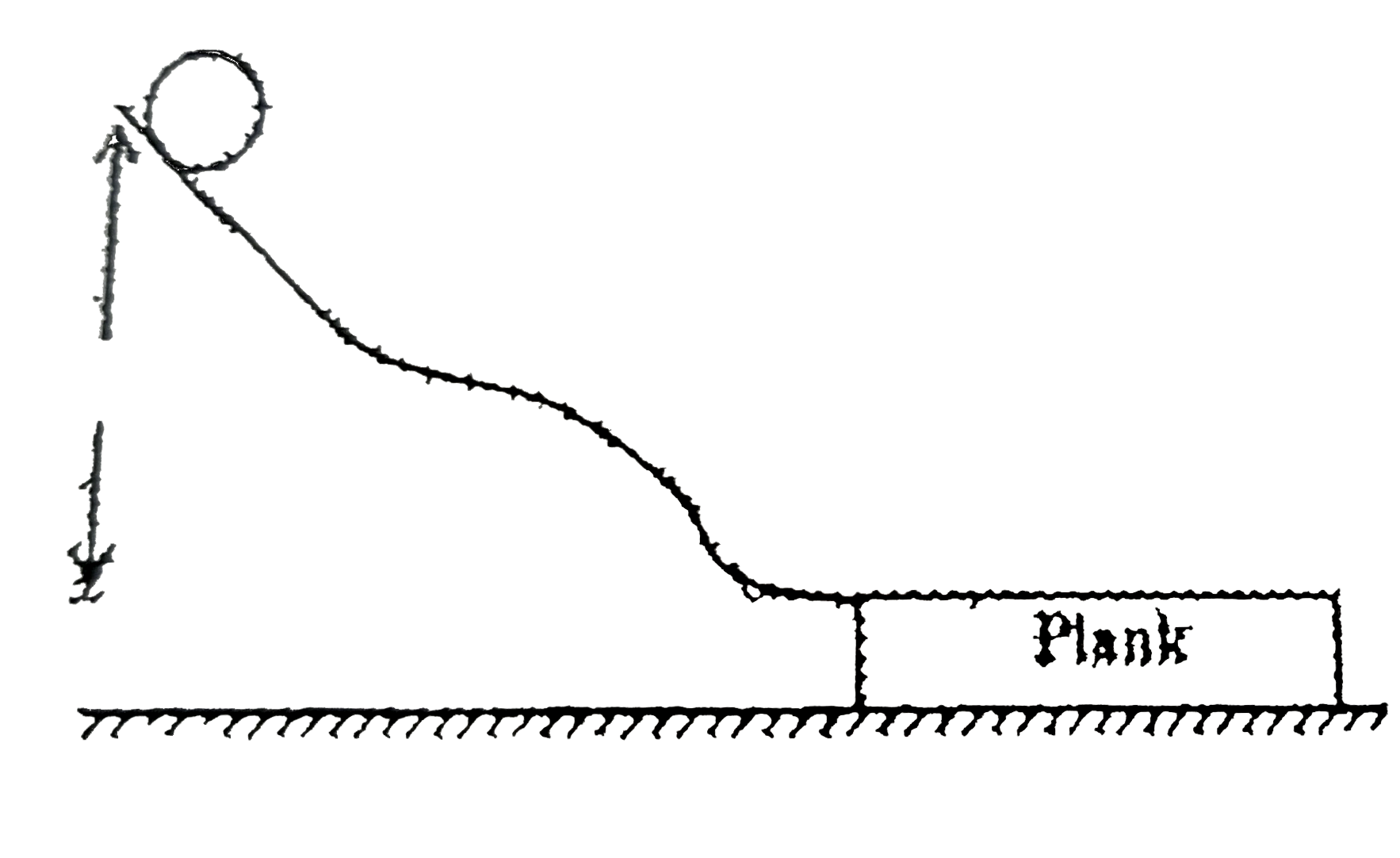
In the figure shown, a solid sphere of mass 'm' and radius r is released from a height 6r to slide down a smooth surface. A plank of same mass 'm' touches the horizontal portion of the surface at the ground. The co-efficient of friction between the plank and the sphere is μ and that between the plank and the ground is μ/4. Find the work done by the friction force between the plank and the ground till the sphere starts pure rolling on the plank. Neglect the height of the plank.
View SolutionA solid sphere of mass m is lying at rest on a rough horizontal surface. The coefficient of friction between the ground and sphere is μ. The maximum value of F, so that the sphere will not slip, is equal to

View SolutionA solid sphere, disc and hollow sphere are placed at the top of an inclined plane. Surface is sufficiently rough to provide pure rolling. All the objects are released from a state of rest
View SolutionA solid sphere of mass m is lying at rest on a rough horizontal surface. The coefficient of friction between the ground and sphere is μ. The maximum value of F, so that the sphere will not slip, is equal to

View Solution
A solid sphere of mass ma nd radis R is placed on a rough horizontal surface A horizontal force F is applied to sphere at a height h, (0≤h≤R) from centre. If sphere rolls slipping then,
View Solution
