The kinetic energy K of a particle moving along a circle of radius R depends upon the distance s as K=as2. The force acting on the particle is
(a) 2as2R
(b) 2as[1+s2R]1/2
(c) 2as
(d) 2a
The correct Answer is:B
To find the force acting on a particle moving along a circle of radius R, given that its kinetic energy K depends on the distance s as K=as2, we can follow these steps:
Step 1: Relate Kinetic Energy to Velocity
The kinetic energy K of a particle is given by the formula:
K=12mv2
where m is the mass of the particle and v is its velocity. Given that K=as2, we can set the two expressions for kinetic energy equal to each other:
as2=12mv2
Step 2: Solve for Velocity
From the equation as2=12mv2, we can solve for v2:
v2=2as2m
Step 3: Differentiate to Find Tangential Acceleration
The tangential acceleration at is defined as the rate of change of velocity with respect to time. We can differentiate v2 with respect to time t:
dvdt=ddt(√2as2m)
Using the chain rule, we have:
dvdt=12(2as2m)−1/2⋅ddt(2as2m)
Since ddt(s2)=2sdsdt, we can write:
dvdt=12(2as2m)−1/2⋅2a⋅2sdsdtm
This simplifies to:
dvdt=2asdsdtm√2as2m
Step 4: Find Tangential Force
The tangential force Ft acting on the particle is given by:
Ft=mdvdt
Substituting dvdt from the previous step, we get:
Ft=m⋅2asdsdtm√2as2m
This simplifies to:
Ft=2asdsdt√2as2m
Step 5: Find Centripetal Force
The centripetal force Fc required to keep the particle moving in a circle is given by:
Fc=mv2R
Substituting v2 from Step 2:
Fc=m⋅2as2mR=2as2R
Step 6: Calculate the Net Force
The net force F acting on the particle is the combination of tangential and centripetal forces:
F=√F2t+F2c
Substituting the expressions for Ft and Fc:
F=√(Ft)2+(2as2R)2
Final Expression
After simplifying, we can express the net force acting on the particle as:
F=√(2as)2+(2as2R)2
The kinetic energy K of a particle is given by the formula:
K=12mv2
where m is the mass of the particle and v is its velocity. Given that K=as2, we can set the two expressions for kinetic energy equal to each other:
as2=12mv2
From the equation as2=12mv2, we can solve for v2:
v2=2as2m
The tangential acceleration at is defined as the rate of change of velocity with respect to time. We can differentiate v2 with respect to time t:
dvdt=ddt(√2as2m)
Using the chain rule, we have:
dvdt=12(2as2m)−1/2⋅ddt(2as2m)
Since ddt(s2)=2sdsdt, we can write:
dvdt=12(2as2m)−1/2⋅2a⋅2sdsdtm
This simplifies to:
dvdt=2asdsdtm√2as2m
The tangential force Ft acting on the particle is given by:
Ft=mdvdt
Substituting dvdt from the previous step, we get:
Ft=m⋅2asdsdtm√2as2m
This simplifies to:
Ft=2asdsdt√2as2m
The centripetal force Fc required to keep the particle moving in a circle is given by:
Fc=mv2R
Substituting v2 from Step 2:
Fc=m⋅2as2mR=2as2R
The net force F acting on the particle is the combination of tangential and centripetal forces:
F=√F2t+F2c
Substituting the expressions for Ft and Fc:
F=√(Ft)2+(2as2R)2
After simplifying, we can express the net force acting on the particle as:
F=√(2as)2+(2as2R)2
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Multiple Correct25videos
WORK, POWER & ENERGY
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Linked Comprehension55videos
WORK, POWER & ENERGY
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Subjective23videos
WORK, POWER & ENERGY
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
The kinetic energy K of a particle moving along a circle of radius R depends upon the distance S as K=βS2 , where β is a constant. Tangential acceleration of the particle is proportional to
View SolutionThe kinetic energy of a particle moving along a circle of radius R depends on the distance covered s as K.E = KS2 where K is a constant. Find the force acting on the particle as a function of S -
View SolutionThe kinetic energy of a particle moving along a circle of radius R depends on the distance covered S as K =α.S2, where α is a constant. Find the force acting on the particle as a function of S.
View Solution(a) A ball, suspended by a thread, swings in a vertical plane so that its acceleration in the extreme and the lowest positions are equal. The angle θ of thread deflection in the extreme position will be
( b) The kinetic energy of a particle moving in a circle of radius R depens on the distance covered as T=as2 , where 'a' is a constant. Find the force acting on the particle as a function of s.View SolutionKinetic energy of a particle moving in a straight line is proportional to the time t. The magnitude of the force acting on the particle is :
View SolutionKinetic energy of a particle moving in a straight line varies with time t as K=4t2. The force acting on the particle
View SolutionThe kinetic energy K of a particle moving along x - axis varies with its position (x) as shown in figure
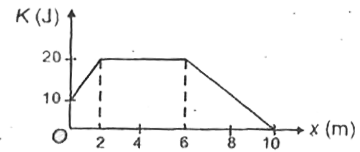
The magnitude of force acting on particle at x = 9 misView SolutionThe kinetic energy of a particle of mass m moving with speed v is given by K=12mv2. If the kinetic energy of a particle moving along x-axis varies with x as K(x)=9−x2, then The region in which particle lies is :
View SolutionA particle moves along a circular path of radius R. The distance and displacement of a particle after one completer revolution is
View SolutionA particle is moving along a circle of radius 20cm, with a linear velocity of 2m//s. Calculate the angular velocity of the particle.
View Solution

