The magnetic field vector of an electromagnetic wave is given by B=B0ˆi+ˆj√2cos(kz−ωt), where ˆi,ˆj represents unit vector along x and y - axis repectively .At t=0 s , two electric charges q1of 4π coulomb and q2 of 2π coulmb located at (0,0,πk)and(0,0,3πk), repectively ,have the same velocity of 0.5cˆi ,(where c is the velocity of light ).The ratio of the force actig on charge q1 to q2 is :
2:1
2√2:1
1:√2
√2:1
To solve the problem, we need to find the ratio of the forces acting on two charges q1 and q2 in a magnetic field generated by an electromagnetic wave. The magnetic field vector is given as:
B=B0√2(^i+^j)cos(kz−ωt)
Step 1: Evaluate the magnetic field at t=0
At t=0, the magnetic field simplifies to:
B=B0√2(^i+^j)cos(kz)
Step 2: Calculate the magnetic field at the positions of the charges
For charge q1 located at (0,0,πk):
B1=B0√2(^i+^j)cos(k⋅πk)=B0√2(^i+^j)cos(π)=−B0√2(^i+^j)
For charge q2 located at (0,0,3πk):
B2=B0√2(^i+^j)cos(k⋅3πk)=B0√2(^i+^j)cos(3π)=−B0√2(^i+^j)
Step 3: Calculate the forces on the charges
The force on a charge in a magnetic field is given by:
F=qv×B
Both charges have the same velocity:
v=0.5c^i
For charge q1=4π:
F1=4π(0.5c^i)×(−B0√2(^i+^j))
Calculating the cross product:
F1=4π(0.5c)(−B0√2)(^i×^i+^i×^j)=4π(0.5c)(−B0√2)(0+^k)=−4πB0c2√2^k
For charge q2=2π:
F2=2π(0.5c^i)×(−B0√2(^i+^j))
Calculating the cross product:
F2=2π(0.5c)(−B0√2)(^i×^i+^i×^j)=2π(0.5c)(−B0√2)(0+^k)=−2πB0c2√2^k
Step 4: Find the ratio of the forces
Now, we can find the ratio of the magnitudes of the forces:
|F1||F2|=4πB0c2√22πB0c2√2=4π2π=2
Final Answer
The ratio of the force acting on charge q1 to that on charge q2 is:
2
Topper's Solved these Questions
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Physics Section B20videos
JEE MAINS 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Physics (Section A)81videos
JEE MAINS 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - PHYSICS30videos
JEE MAINS 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2020EXERCISE - PHYSICS246videos
JEE MAINS 2020
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2022EXERCISE - PHYSICS (SECTION -B)10videos
JEE MAINS 2022
Similar Questions
A varying magnetic field exist in space as follows B=B0(ˆi+ˆj√2[cos(kz−wt)] two charge particles q1=4π C and q2=2π C placed at (0,0,πk) and (0,0,3πk) and moving with equal velocities of 0.5cˆi then find ratio of force on two charge at t= 0s is
View SolutionTwo charges q1andq2 are placed at (0,0,d) and (0,0,-d) respectively. Find locus of points where the potential is zero.
View Solution
Knowledge Check
- Question 1 - Select One
The magnetic field of a plane electromagnetic wave is given by: →B=B0ˆi−[cos(kz−ωt)]+B1ˆjcos(kz+ωt) where B0=3×10−5T and B1=2×10−6T. The rms value of the force experienced by a stationary charge Q=10−4C at z=0 is close to:
A0.1 NB3×10−2NC0.6 ND0.9 N - Question 2 - Select One
A charge particle q1, is at position (2, -1, 3). The electrostatic force on another charged particle q2 at (0, 0, 0) is :
Aq1q256π∈0(2ˆi−ˆj+3ˆk)Bq1q256√14π∈0(2ˆi−ˆj+3ˆk)Cq1q256π∈0(ˆj−2ˆi−3ˆk)Dq1q256√14π∈0(ˆj−2ˆi−3ˆk) - Question 3 - Select One
A system has two charges qA=+2.5×10−7CandqB=+2.5×10−7C located at point A: (0,0,-15 cm)and B: (0,0,+15 cm), respectively. What are the total charge and eletric dipole moment of the system?
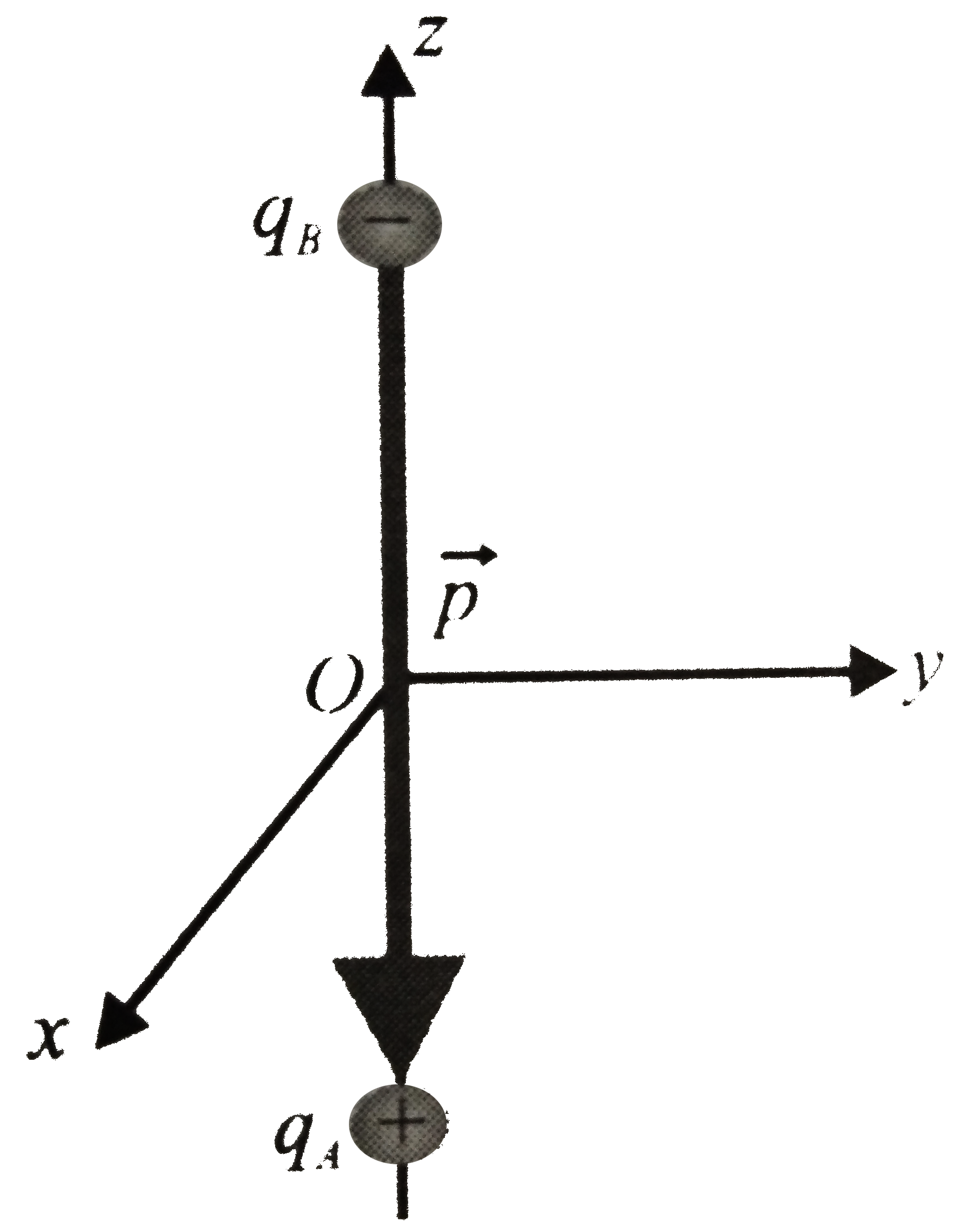 A0 , 0B7.5×10−8C , 7.5×10−8CmC7.5×10−8C , 0D0 , 7.5×10−8Cm
A0 , 0B7.5×10−8C , 7.5×10−8CmC7.5×10−8C , 0D0 , 7.5×10−8Cm
Two charges q1andq2 are placed at (0,0,d) and (0,0,-d) respectively. Find locus of points where the potential is zero.
View SolutionTwo charges q1andq2 are placed at (0,0d)and(0,0−d) respectively. Find locus of points where the potential is zero.
View SolutionA particle is moving in a plane with velocity given by u=u0i+(aωcosωt)j, where i and are j are unit vectors along x and yaxes respectively. If particle is at the origin at t=0.
(a) Calculate the trajectroy of the particle.
(b) Find the distance form the origin at time 3π/2ω.View SolutionA system has two charges qA=+2.5×10−7CandqB=−2.5×10−7C located at point A: (0,0,-15 cm)and B: (0,0,+15 cm), respectively. What are the total charge and electric dipole moment of the system?
 View Solution
View SolutionElectric field in space is given by −−→E(t)=E0i+j√2cos(ωt+Kz). A positively charged particle at (0,0,πK) is given velocity v0ˆk at t = 0. Direction of force acting on particle is
View Solution

