The magnetic flux across a loop of resistance 10Ω is given by ϕ=5t2−4t+1Wb. How much current is induced in the loop after 0.2 s?
0.4 A
0.2 A
0.04 A
0.02 A
The correct Answer is:B
To find the induced current in the loop after 0.2 seconds, we can follow these steps:
Step 1: Write down the expression for magnetic flux.
The magnetic flux (φ) across the loop is given by:
ϕ=5t2−4t+1Wb
Step 2: Differentiate the magnetic flux to find the induced EMF.
According to Faraday's law of electromagnetic induction, the induced electromotive force (EMF) (ε) is given by the negative rate of change of magnetic flux:
ϵ=−dϕdt
Now, we differentiate φ with respect to time (t):
dϕdt=ddt(5t2−4t+1)=10t−4
Thus, the induced EMF becomes:
ϵ=−(10t−4)=−10t+4
Step 3: Substitute the time value into the induced EMF equation.
We need to find the induced EMF at t=0.2 seconds:
ϵ=−10(0.2)+4=−2+4=2V
Step 4: Calculate the induced current using Ohm's law.
Ohm's law states that the current (I) can be calculated using the formula:
I=ϵR
where R is the resistance of the loop. Given that the resistance R=10Ω:
I=2V10Ω=0.2A
Conclusion:
The induced current in the loop after 0.2 seconds is:
I=0.2A
---
The magnetic flux (φ) across the loop is given by:
ϕ=5t2−4t+1Wb
According to Faraday's law of electromagnetic induction, the induced electromotive force (EMF) (ε) is given by the negative rate of change of magnetic flux:
ϵ=−dϕdt
Now, we differentiate φ with respect to time (t):
dϕdt=ddt(5t2−4t+1)=10t−4
Thus, the induced EMF becomes:
ϵ=−(10t−4)=−10t+4
We need to find the induced EMF at t=0.2 seconds:
ϵ=−10(0.2)+4=−2+4=2V
Ohm's law states that the current (I) can be calculated using the formula:
I=ϵR
where R is the resistance of the loop. Given that the resistance R=10Ω:
I=2V10Ω=0.2A
The induced current in the loop after 0.2 seconds is:
I=0.2A
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Taking it together119videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrance special format questions17videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Example71videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery97videos
CURRENT ELECTRICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C22videos
ELECTROMAGNETIC WAVES
Similar Questions
The magnetic flux linked with a coil (in Wb) is given by the equation ϕ=5t2+3t+16 . The magnetic of induced emf in the coil at fourth second will be
View SolutionThe magnetic flux linked with a coil, in webers is given by the equation ϕ=3t2+4t+9. Then, the magnitude of induced emf at t = 2 s
View Solution
Knowledge Check
- Question 1 - Select One
The magnetic flux through a coil perpendicluar to its plane and directed into paper is varying according to the relation ϕ=(2t2+4t+6)mWb. The emf induced in the loop at t=4 s is
A0.12 VB2.4 VC0.02 VD1.2 V - Question 2 - Select One
The magnetic flux (ϕ) in a closed circuit of resistance 20Ω varies with time (t) according to the equation ϕ=7t2−4t where ϕ is in weber and t is in seconds. The magnitude of the induced current at t =0.25s is
A25mAB0.025mAC47 mAD175 mA
A time varying magnetic flux passing through a coil is given by ϕ=xt2, if at t=3s, the emf induced is 9 V, then the value of x is
View SolutionA plane loop, shaped as two squares of sides a=1m and b=0.4m is introduced into a uniform magnetic field ⊥ to the plane of loop . The magnetic field varies as B=10(−3)sin(100t)T. The qamplitude of the current induced in the loop if its resistance per unit length is r=5mΩm−1 is
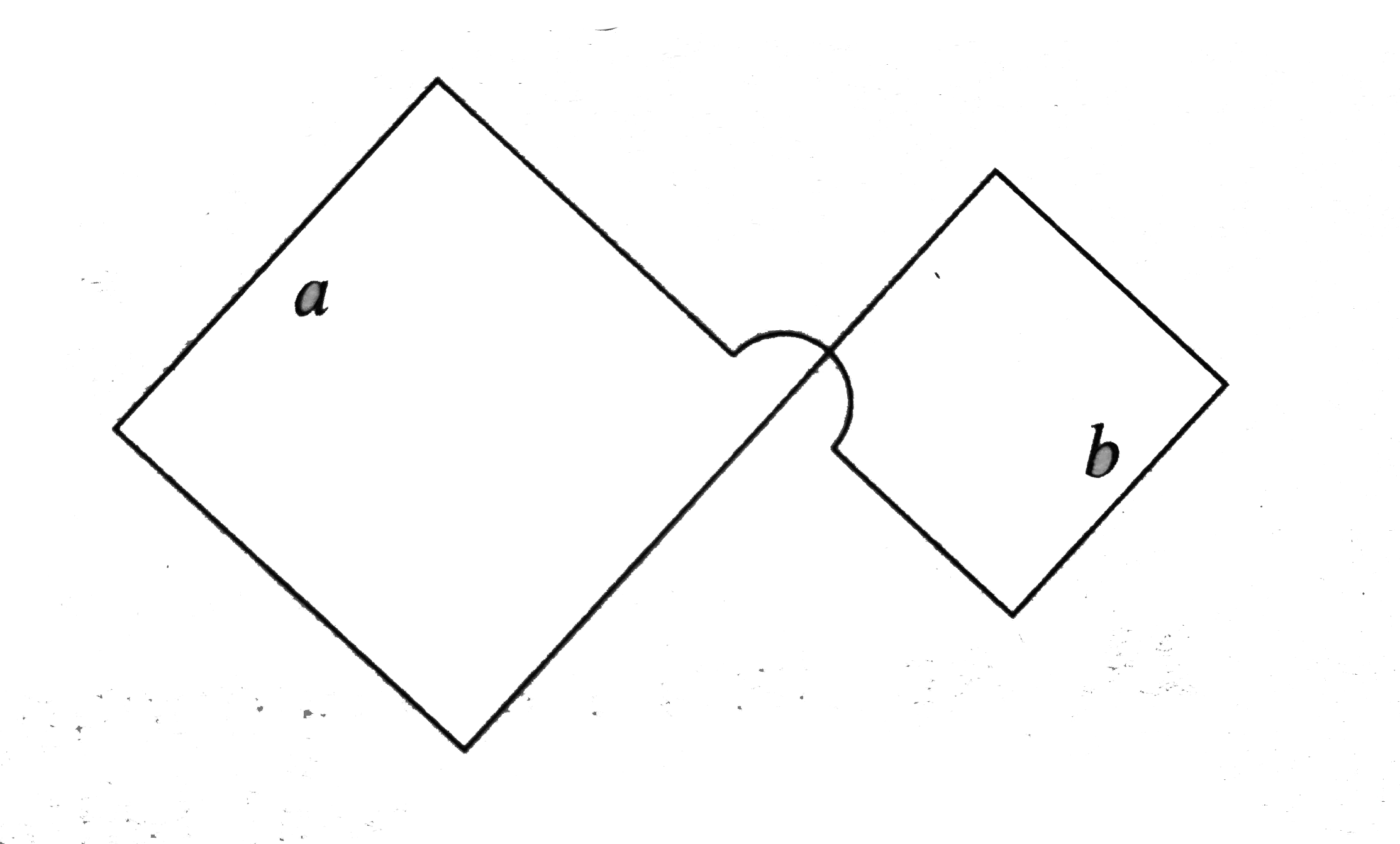 View Solution
View SolutionThe flux of magnetic field through a closed conducting loop of resistance 0.4Ω changes with time according to the equation Φ=0.20t2+0.40t+0.60 where t is time in seconds.Find (i)the induced emf at t=2s.(ii)the average induced emf in t=0 to t=5s.(iii)charge passed through the loop in t=0 to t=5s (iv)average current.In time interval t=0 to t=5s (v) heat produced in t=0 to t=5s
View SolutionA circular loop is placed in magnetic field B=2t. Find the direction of induced current produced in the loop.
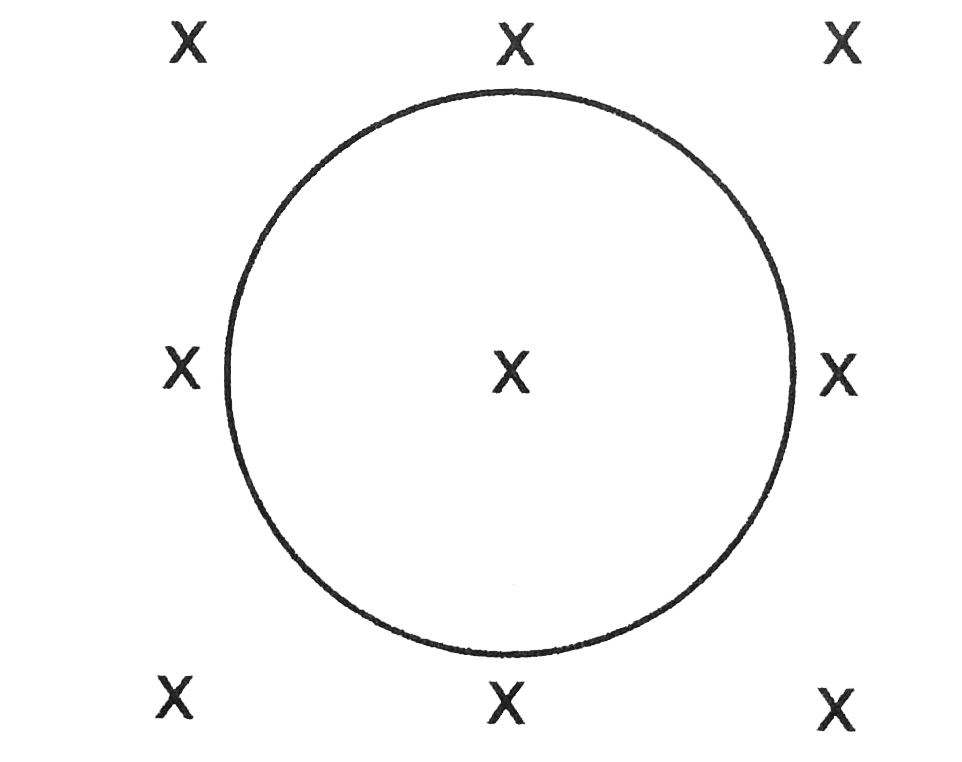 View Solution
View SolutionA square conductind loop, 20.0cm on a side placed in the same magnetic field as shown in Fig. 3.195, center of the magnetic field region, where dB/dt=0.035Ts−1.

The current induced in the loop if its resistance is 2.00Ω isView SolutionThe flux linked with a coil at any instant 't' is given by ϕ=10t2−50t+250
The induced emf at t=3s isView Solution

