Trajectory of particle in projectile motion is y=(x−x2/80), x and y are in metre.
Projectile range is on horizontal plane.
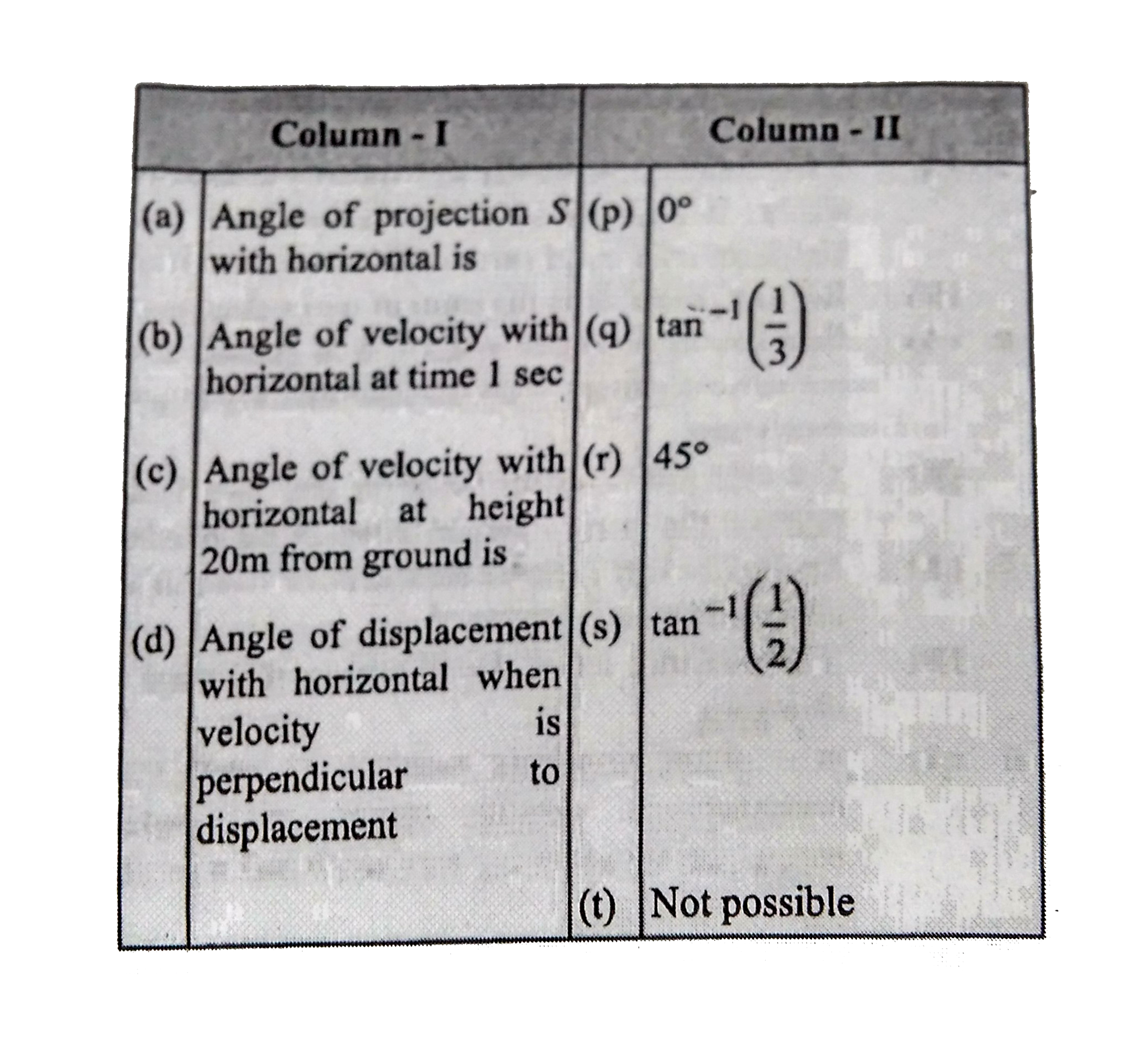
The correct Answer is:a→r;b→s;c→p;d→t
y=xtanθ−gx22u2cos2θ
y=(x−x280),θ=45∘
u2=800
u=√800
uy=uy−>
uy=20−10
uy=10,vx=20
tanθ=12⇒θ=tan−1(12)
Topper's Solved these Questions
Similar Questions
Trajectory of a particle in a projectile motion is given as y=x−x280. Here x and y are in metre. For this projectile motion the following with g=10m/s2.
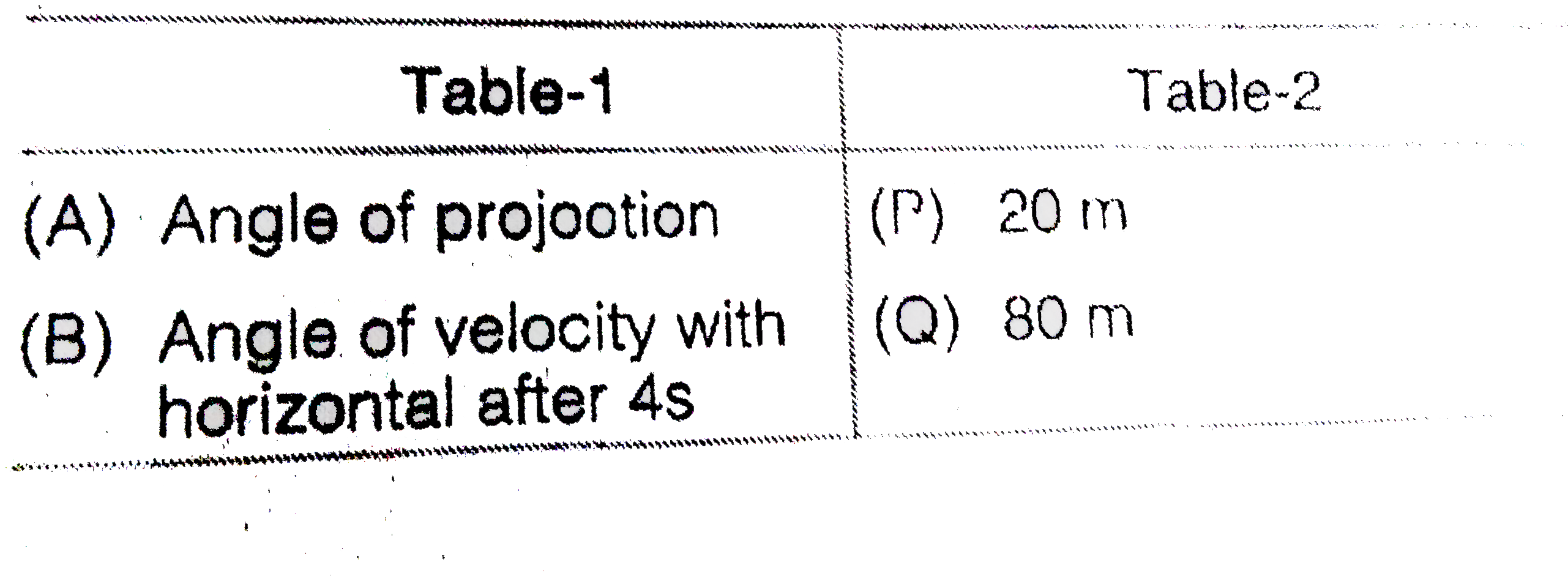
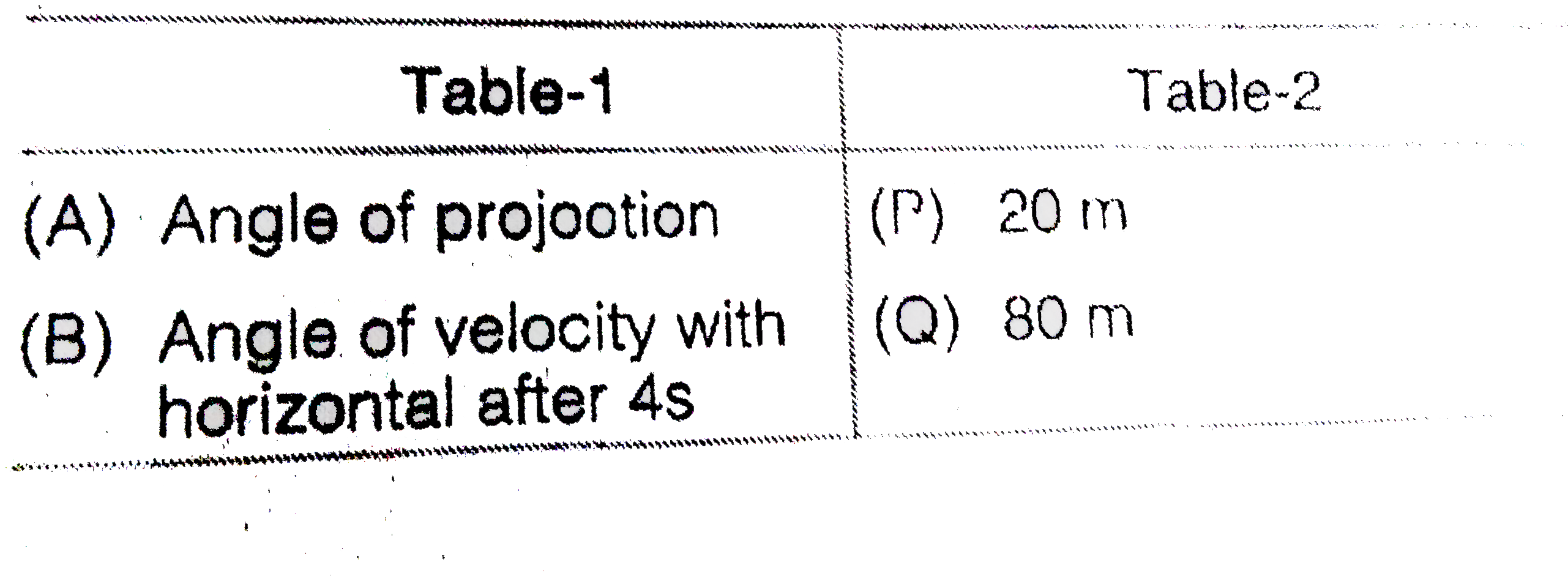
View SolutionTrajectory of particle in a projectile motion is given as y=x−x280. Here, x and y are in meters. For this projectile motion, match the following with g=10m/s2.
Column-IColumn-II(A)Angle of projection (in degrees)(P)20(B)Angle of velocity with horizontal after 4s (in degrees)(Q)80(C)Maximum height (in meters)(R)45(D)Horizontal range (in meters)(S)30(T)60
View Solution
Knowledge Check
The trajectory of a projectile in a vertical plane is y=ax−bx2, where a and b are constants and x and y are respectively horizontal and vertical distances of the projectile from the point of projection. The maximum height attained by the particle and the angle of projection form the horizontal are:
Ab22a,tan−1(b)Ba2b,tan−1(2b)Ca24b,tan−1(a)D2a2b,tan−1(a)
Trajectory of particle in a projectile motion is given as y=x−x280. Here, x and y are in meters. For this projectile motion, match the following with g=10m/s2.
Column-IColumn-II(A)Angle of projection (in degrees)(P)20(B)Angle of velocity with horizontal after 4s (in degrees)(Q)80(C)Maximum height (in meters)(R)45(D)Horizontal range (in meters)(S)30(T)60
View SolutionTrajectory of particle in a projectile motion is given as: y=x−(x2/80) (x and y are in meters). Take g=10m/s2.

View SolutionTrajectory of particle in a projectile motion is given as y=x−x280. Here x and y are in metre. For this projectile motion match the following with g=10ms−2.

View SolutionTrajectory of particle in a projectile motion is given as y=x−x280. Here x and y are in metre. For this projectile motion match the following with g=10ms−2.

View SolutionTrajectory of a particle in a projectile motion is given by y=x−x280 where x and y are in meters. Match the column-1 and column-2.
Column-IColumn-II(A)x coordinate at height of 15 m(P) 20 m(B)vertical distnce of particle frompoint of projection at x= 100 m(Q) 80 m(C)Horizontal range(R) 60 m(S) 25 m
View Solution[" The equation of a trajectory of a "],[" projectile is "],[[y=3x-0.02x^(2)," where "'x'" and "'y'],[" are in meters,range of projectile is "],[" (A) "150m],[" (B) "300m],[" (C) "450m],[" (D) "600m]]
View SolutionThe equation of a trajectory of a projectile is y=8x−4x2. The maximum height of the projectile is ?
View Solution
