Two concentric circular loops of radius 1 cm and 20 cm are placed coaxially.
(i) Find mutual inductance of the arrangement
(ii) If the current passed through the outer loop is changed at a rate of 5 A/ms, find the emf induced in the inner loop. Assume the magnetic field on the inner loop to be uniform.
(i) Let there be two concentric circular coils of radii r and R respectively. Where R>>r. If a current I1 , flows through the outer coil, then magnetic field developed at its centre is
B=μ0I12R
As r is very small, magnetic field on the inner loop may be assumed to be uniform at
B=μ0I12R
∴ Magnetic flux linked with inner loop ϕ2=B(πr2)=μ0I1πr22R
∵ϕ2=MI1
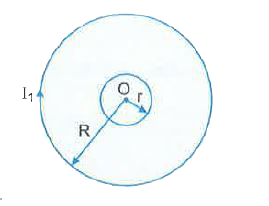
∴ Mutual inductance of the given pair of coils M=ϕ2I1=μ0πr22R
As per question R =20 cm = 0.2 m and r =1 cm = 0.01 m
∴M=(4π×10−7)×π×(0.01)22×0.2=9.87×1010 H
(ii) If rate of change of current in outer loop i.e., dI1dt=5A/ms=5×103As−1 then magnitude of induced emf in the inner loop is
ε2=MdI1dt=(9.87×10−10)×(5×10−3)=4.935×10−6V=4.9μV
Topper's Solved these Questions
- CBSE EXAMINATION PAPER 2020 (SOLVED)BOOK - U-LIKE SERIESCHAPTER - CBSE EXAMINATION PAPER 2020 (SOLVED)EXERCISE - SECTION C
11 Videos
- CBSE EXAMINATION PAPER 2020 BOOK - U-LIKE SERIESCHAPTER - CBSE EXAMINATION PAPER 2020 EXERCISE - SECTION D
12 Videos
- CBSE EXAMINATION PAPER 2020 (SOLVED) BOOK - U-LIKE SERIESCHAPTER - CBSE EXAMINATION PAPER 2020 (SOLVED) EXERCISE - SECTION - D
6 Videos
Similar Questions
Figure shows two concentric loops of radii R and r (R gtgt r). A current i is passed through the outer loop. Find the flux linked with inner loop and the mutual inductance of the arrangement.
View SolutionFigure shows two concentric loops of radii R and r (R gtgt r). A current i is passed through the outer loop. Find the flux linked with inner loop and the mutual inductance of the arrangement.
View Solution
Knowledge Check
Two different wire loops are concentric and lie in the same plane. The current in the outer loop is clockwise and increasing with time. The induced current in the inner loop then is
AclockwiseBzeroCcounter clockwiseDin a direction that depends on the ratio of the, loop radii
A circular loop of radius a, carrying a current I, is placed in a tow dimensional magnetic field. The centre of the loop coincides with the centre of the filed The strenght of the magnetic field at the pariphery of the loop is B. find the magnetic force on the wire.

View SolutionA circular loop of radius a, carrying a current I, is placed in a tow dimensional magnetic field. The centre of the loop coincides with the centre of the filed The strenght of the magnetic field at the pariphery of the loop is B. find the magnetic force on the wire.

View SolutionA circular loop of radius 9.7 cm carries a current 2.3 A. Obtain the magnitude of the magnetic field at the centre of the loop .
View SolutionTwo different wire loops are concentric and lie in the same plane. The current in the outer loop (I) is clockwise and increases with time. The induced current in the inner loop

View SolutionTwo different wire loops are concentric and lie in the same plane. The current in the outer loop is clockwise and increasing with time. The induced current in the inner loop then is
View SolutionA conducing circular loop is placed in a uniform magnetic field of 0.02 T, woth its plane perpendicular to the field. If the redius of the loop starts shrinking at a constant rate of 1.0mm/s, then find the emf induced in the loop, at the instant when radius is 4 cm.
View SolutionA semi circular current loop is placed in an uniform magnetic field of 1 tesla as shown. If the radius of loop is 1 m, the magnetic force on the loop is
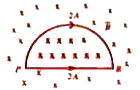
View Solution
