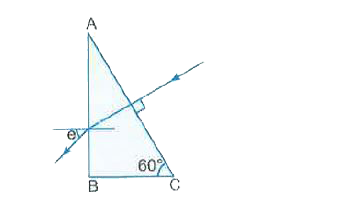
Calculate the angle of emergence (e) of the ray of light incident normally on the face AC of a glass prism ABC of refractive index $\sqrt{3}$ . How will the angle of emergence change qualitatively, if the ray of light emerges from the prism into a liquid of refractive index 1.3 instead of air ?
$Calculate the angle of emergence (e) of the ray of light incident normally on the face AC of a glass prism ABC of refractive index sqrt(3). How will the angle of emergence change qualitatively, if the ray of light emerges from the prism into a liquid of refractive index 1.3 instead of air ?$

Text Solution

Verified by Experts

As shown in the diagram, the angle of incidence Zi at the face AB of glass prism ABC is $30^{\circ}$ and refractive index of glass prism n = $\sqrt{3}$ .
If $∠ e$ be the angle of emergence in air medium then as per snell.s law:
$\frac{sin i}{sin e} = n_{a g} = \frac{1}{n_{g n}} \Rightarrow \frac{{sin 30}^{\circ}}{sin e} = \frac{1}{\sqrt{3}}$
$\Rightarrow sin e = \sqrt{3} \times {sin 30}^{\circ} = \frac{\sqrt{3}}{2} \Rightarrow e = {sin}^{- 1} (\frac{\sqrt{3}}{2}) = 60^{\circ}$

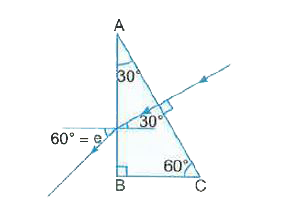
If the prism is immersed in a liquid of refractive index $n_{g}, = 1.3$ , then new angle of emergence e will be given by :
$\frac{sin i}{sin e} = \frac{1}{n_{g}} or sin e = n_{g l} \times sin I = \frac{\sqrt{3}}{1.3} \times {sin 30}^{\circ}$
$\Rightarrow sin e = \frac{\sqrt{3}}{1.3} \times \frac{1}{2} = \frac{1}{1.3} (\frac{\sqrt{3}}{2})$
Obviously sin e. $<$ sin e and hence e. $<$ e. So the angle of emergence decreases when the prism is immersed in the liquid.

Updated on:21/07/2023

Light falls at normal incidence on one face of a glass prism of refractive index $\sqrt{2}$ . Then the angle of emergence when the angle of the prism is $45^{\circ}$
A45°
B60°
C15°
D90°
A ray of light is incident normally on one of the equal faces of a right isosceles prism of refractive index 1.5. The angle between incident and emergent rays is
A $45^{\circ}$
B $90^{\circ}$
C $180^{\circ}$
D $0^{\circ}$
A monochromatic ray of light is incident normally on one of the refracting surfaces of a prism . The emergent ray grazes the second refracting surface . What is the angle of the prism if the refractive index of the material of the prism is $1.6$ ?
A $A < 2 C$
BA = 2C
C $A > 2 C$
D $A < \frac{C}{2}$