Two concentric rings placed in a gravity free region in yz-plane one of radius R carries a+Q and second of radius 4R and charge –8Q distributed uniformly over it. Find the velocity with which a point charge of mass m and charge +q should be projected from a a distance 3R from the centre of rings on its axis so that it will reach to the centre of the rings.
The correct Answer is:A, B, C
To solve the problem, we will use the principle of conservation of energy. We need to find the velocity v with which a point charge +q should be projected from a distance 3R from the center of the concentric rings so that it reaches the center of the rings.
1. Identify the Charges and Distances:
- The inner ring has a charge +Q and radius R.
- The outer ring has a charge −8Q and radius 4R.
- The point charge +q is projected from a distance 3R from the center of the rings.
2. Calculate Initial Potential Energy (PE_initial):
- The distance from the charge +q to the charge +Q when it is at 3R:
d1=√(3R)2+R2=√9R2+R2=√10R2=√10R
- The potential energy due to the inner ring:
PE1=14πϵ0⋅Qq√10R
- The distance from the charge +q to the charge −8Q:
d2=√(3R)2+(4R)2=√9R2+16R2=√25R2=5R
- The potential energy due to the outer ring:
PE2=14πϵ0⋅−8Qq5R
- Total initial potential energy:
PEinitial=PE1+PE2=14πϵ0(Qq√10R−8Qq5R)
3. Calculate Final Potential Energy (PE_final):
- When the charge +q reaches the center of the rings, the distances are:
- To the inner ring: R
- To the outer ring: 4R
- The potential energy at the center:
PEfinal=14πϵ0(QqR−8Qq4R)=14πϵ0(QqR−2QqR)=14πϵ0(−QqR)
4. Apply Conservation of Energy:
- The total mechanical energy is conserved:
KEinitial+PEinitial=KEfinal+PEfinal
- The initial kinetic energy KEinitial=12mv2 and KEfinal=0 (since it stops at the center):
12mv2+PEinitial=0+PEfinal
5. Set Up the Equation:
12mv2=−PEinitial+PEfinal
12mv2=−(14πϵ0(Qq√10R−8Qq5R))+(14πϵ0(−QqR))
6. Simplify and Solve for v:
- Rearranging and simplifying the equation will allow us to isolate v:
12mv2=14πϵ0(Qq√10R−8Qq5R+QqR)
- After simplification, solve for v:
v=
⎷2m⋅14πϵ0(Qq√10R−8Qq5R+QqR)
- The inner ring has a charge +Q and radius R.
- The outer ring has a charge −8Q and radius 4R.
- The point charge +q is projected from a distance 3R from the center of the rings.
- The distance from the charge +q to the charge +Q when it is at 3R:
d1=√(3R)2+R2=√9R2+R2=√10R2=√10R
- The potential energy due to the inner ring:
PE1=14πϵ0⋅Qq√10R
d2=√(3R)2+(4R)2=√9R2+16R2=√25R2=5R
- The potential energy due to the outer ring:
PE2=14πϵ0⋅−8Qq5R
PEinitial=PE1+PE2=14πϵ0(Qq√10R−8Qq5R)
- When the charge +q reaches the center of the rings, the distances are:
- To the inner ring: R
- To the outer ring: 4R
PEfinal=14πϵ0(QqR−8Qq4R)=14πϵ0(QqR−2QqR)=14πϵ0(−QqR)
- The total mechanical energy is conserved:
KEinitial+PEinitial=KEfinal+PEfinal
- The initial kinetic energy KEinitial=12mv2 and KEfinal=0 (since it stops at the center):
12mv2+PEinitial=0+PEfinal
12mv2=−PEinitial+PEfinal
12mv2=−(14πϵ0(Qq√10R−8Qq5R))+(14πϵ0(−QqR))
- Rearranging and simplifying the equation will allow us to isolate v:
12mv2=14πϵ0(Qq√10R−8Qq5R+QqR)
- After simplification, solve for v:
v=
⎷2m⋅14πϵ0(Qq√10R−8Qq5R+QqR)
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Check point 1.1
10 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Check point 1.2
15 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Subjective
15 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
A ring of radius a contains a charge q distributed. uniformly ober its length. Find the electric field at a point. on the axis of the ring at a distance x from the centre.
View SolutionA thin semi-circular ring of radius r has a positive charge q distributed uniformly over it. The net field →E at the centre O is

View SolutionThe minimum velocity v with which charge q should be projected so that it manages to reach the centre of the ring starting from the position shown in figure is
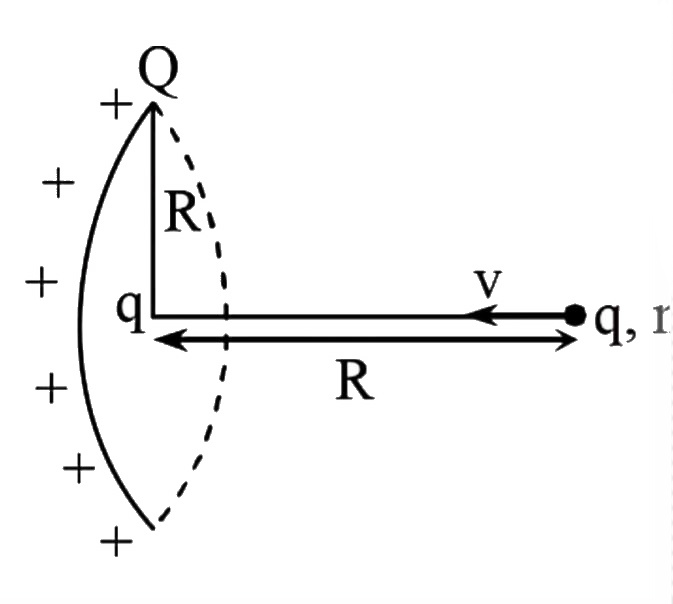
View SolutionA ring of radius R has charge −Q distributed uniformly over it. Calculate the charge that should be placed at the center of the ring such that the electric field becomes zero at apoint on the axis of the ring at distant R from the center of the ring.
View SolutionThe electrostatic potential of a uniformly charged thin spherical shell of charge Q and radius R at a distance r from the centre
View SolutionA point charge q , is placed at a distance r from the centre of an aluminium sphere, of radius R (r>R) . Selectr the correct alternatives.
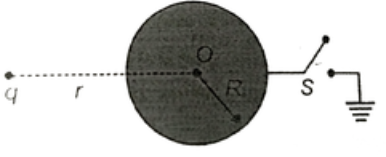
View SolutionA non-conducting ring of radius R has charge Q distributed unevenly over it. If it rotates with an angular velocity ω the equivalent current will be:
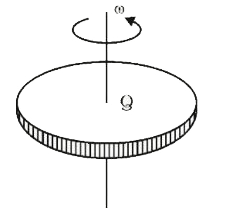
View SolutionConsider the force F on a charge 'q' due to a uniformlycharged spherical shell of radius R carrying charge Q distributed uniformly over it. Which one of the following statements is true for F, if 'q' is placed at distacen r from the centre of the shell?
View SolutionElectric charge Q is uniformly distributed around a thin ring of radius a. find the potential a point P on the axis of the ring at a distance x from the centre of the ring .

View SolutionCharge q is uniformly distributed over a thin half ring of radius R. The electric field at the centre of the ring is
View Solution
