Two identical magnetic dipoles of magnetic moments 2Am2 are placed at a separation of 2m with their axes perpendicular to each other in air. The resultant magnetic field at a mid point between the dipole is
4√5×10−5 T
2√5×10−5 T
4√5×10−7 T
2√5×10−7 T
The correct Answer is:D
To find the resultant magnetic field at the midpoint between two identical magnetic dipoles with their axes perpendicular to each other, we can follow these steps:
Step 1: Understand the Configuration
We have two identical magnetic dipoles, each with a magnetic moment M=2Am2. They are separated by a distance of 2m, and we need to find the magnetic field at the midpoint between them.
Step 2: Determine the Distance to the Midpoint
Since the dipoles are 2m apart, the distance from each dipole to the midpoint P is:
R=22=1m
Step 3: Calculate the Magnetic Field Due to Each Dipole
The magnetic field B due to a magnetic dipole at a distance R is given by the formula:
B=μ0M4πR3
where μ0=4π×10−7T m/A.
For each dipole, substituting M=2Am2 and R=1m:
B=(4π×10−7)×24π×(1)3=2×10−71=2×10−7T
Step 4: Determine the Direction of the Magnetic Fields
Since the axes of the dipoles are perpendicular, the magnetic field B1 due to dipole 1 will be in one direction (say along the x-axis), and the magnetic field B2 due to dipole 2 will be in the perpendicular direction (along the y-axis).
Step 5: Calculate the Resultant Magnetic Field
The resultant magnetic field BP at point P can be calculated using the Pythagorean theorem:
BP=√B21+B22
Substituting B1=2×10−7T and B2=2×10−7T:
BP=√(2×10−7)2+(2×10−7)2=√2×(2×10−7)2=2×10−7√2
Step 6: Final Calculation
Calculating the resultant:
BP=2×10−7√2≈2×10−7×1.414=2.828×10−7T
Conclusion
The resultant magnetic field at the midpoint between the two dipoles is approximately:
BP≈2.828×10−7T
We have two identical magnetic dipoles, each with a magnetic moment M=2Am2. They are separated by a distance of 2m, and we need to find the magnetic field at the midpoint between them.
Since the dipoles are 2m apart, the distance from each dipole to the midpoint P is:
R=22=1m
The magnetic field B due to a magnetic dipole at a distance R is given by the formula:
B=μ0M4πR3
where μ0=4π×10−7T m/A.
B=(4π×10−7)×24π×(1)3=2×10−71=2×10−7T
Since the axes of the dipoles are perpendicular, the magnetic field B1 due to dipole 1 will be in one direction (say along the x-axis), and the magnetic field B2 due to dipole 2 will be in the perpendicular direction (along the y-axis).
The resultant magnetic field BP at point P can be calculated using the Pythagorean theorem:
BP=√B21+B22
Substituting B1=2×10−7T and B2=2×10−7T:
BP=√(2×10−7)2+(2×10−7)2=√2×(2×10−7)2=2×10−7√2
Calculating the resultant:
BP=2×10−7√2≈2×10−7×1.414=2.828×10−7T
The resultant magnetic field at the midpoint between the two dipoles is approximately:
BP≈2.828×10−7T
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - MAGNETISM AND GAUSS S LAW2videos
MAGNETISM AND MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - THE EARTH S MAGNETISM19videos
MAGNETISM AND MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Assertion And Reason15videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - Assertion And Reason15videos
MOVING CHARGES AND MAGNETISM
Similar Questions
Two identical magnetic dipoles of magnetic moments 1⋅0Am2 each are placed at a separation of 2m with their axes perpendicular to each other. What is the resultant magnetic field at a point midway between the dipoles?
View SolutionFigure shows two identical magnetic dipoles aandb of magnetic moments M each, placed at a separation d, with their axes perpendicular to each other. Find the magnetic field at the point P midway between the dipoles.
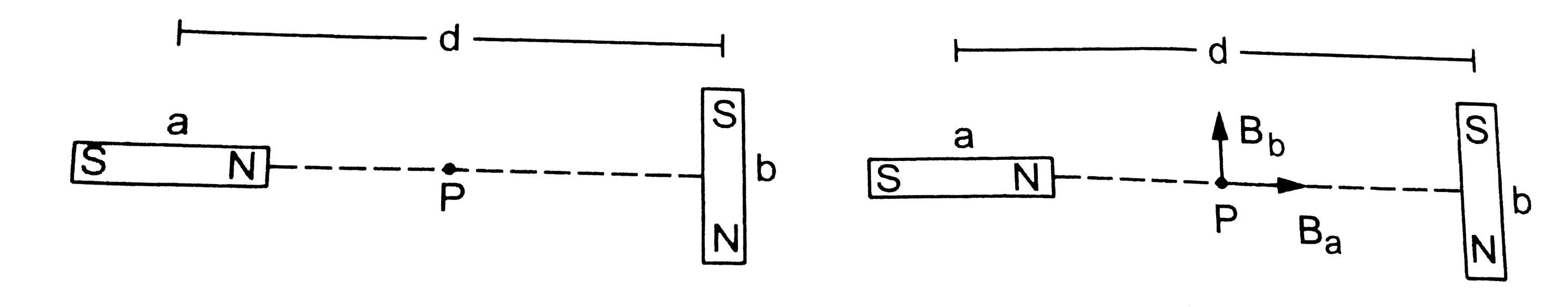 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Two identical magnetic dipoles of magnetic moments 1⋅0Am2 each are placed at a separation of 2m with their axes perpendicular to each other. What is the resultant magnetic field at a point midway between the dipoles?
A√5×10−7TB5×10−7TC√2×10−7TD10−7T
Two identical short bar magnets, each having magnetic moment M, are placed a distance of 2d apart with axes perpendicular to each other in a horizontal plane. The magnetic induction at a point midway between them is
View SolutionTwo identical bar magnets, each of magnetic moment M, are placed as shown in figure. Magnetic field at point
View SolutionTwo identical short bar magnets of magnetic moment M each are placed at distance r apart in end-on position, then magnetic force between both is
View SolutionThree identical bar magnets each of magnetic moment M are arranged in the form of an equilateral triangle such that at two vertices like poles are in contact. The resultant magnetic moment will be
View SolutionA magnetic dipole of magnetic moment 6Am2 is lying in a horizontal plane with its north pole pointing toward 60∘ East of North. Find the net horizontal magnetic field at a point· on the axis of the magnet 0.2m away from it. Horizontal Component of earth field at this place is 30μT.
View SolutionA bar magnet of magnetic moment M1 is axially cut into two equal parts. If these two pieces are arranged perpendiucular to each other, the resultant magnetic moment is M2.
Then the vale of M1M2 isView SolutionA bar magnet of magnetic moment M1 is axially cut into two equal parts. If these two pieces are arranged perpendiucular to each other, the resultant magnetic moment is M2.
Then the vale of M1M2 isView Solution

