Two spherical conductors each of capacity C are charged to potetnial V and −V. These are then conneted by means of a fine wire. The loss of energy will be
zero
12CV2
CV2
2CV2
The correct Answer is:C
To solve the problem of finding the loss of energy when two spherical conductors, each with a capacity C, charged to potentials V and −V respectively, are connected by a fine wire, we can follow these steps:
1. Identify Initial Charges:
Each conductor has a capacitance C. The charge on the first conductor (charged to potential V) is given by:
Q1=CV
The charge on the second conductor (charged to potential −V) is:
Q2=−CV
2. Connect the Conductors:
When the two conductors are connected by a wire, the potential of both conductors will equalize. Let the final potential of both conductors be Vf.
3. Conservation of Charge:
The total initial charge before connecting the conductors is:
Qinitial=Q1+Q2=CV−CV=0
After connecting, since the total charge is conserved, the final charges on both conductors will be equal:
Q1f+Q2f=0
Let Q1f=Q2f=Qf. Therefore:
Qf+Qf=0⟹2Qf=0⟹Qf=0
4. Calculate Initial Potential Energy:
The initial potential energy Ui of the system is the sum of the potential energies of both conductors:
Ui=12CV2+12C(−V)2=12CV2+12CV2=CV2
5. Calculate Final Potential Energy:
After connecting the conductors, since both have zero charge, the final potential energy Uf is:
Uf=0
6. Calculate Loss of Energy:
The loss of energy ΔU is given by:
ΔU=Ui−Uf=CV2−0=CV2
Conclusion:
The loss of energy when the two spherical conductors are connected is:
ΔU=CV2
Each conductor has a capacitance C. The charge on the first conductor (charged to potential V) is given by:
Q1=CV
The charge on the second conductor (charged to potential −V) is:
Q2=−CV
When the two conductors are connected by a wire, the potential of both conductors will equalize. Let the final potential of both conductors be Vf.
The total initial charge before connecting the conductors is:
Qinitial=Q1+Q2=CV−CV=0
After connecting, since the total charge is conserved, the final charges on both conductors will be equal:
Q1f+Q2f=0
Let Q1f=Q2f=Qf. Therefore:
Qf+Qf=0⟹2Qf=0⟹Qf=0
The initial potential energy Ui of the system is the sum of the potential energies of both conductors:
Ui=12CV2+12C(−V)2=12CV2+12CV2=CV2
After connecting the conductors, since both have zero charge, the final potential energy Uf is:
Uf=0
The loss of energy ΔU is given by:
ΔU=Ui−Uf=CV2−0=CV2
The loss of energy when the two spherical conductors are connected is:
ΔU=CV2
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - HOTS
5 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - EXEMPLAR PROBLEMS
3 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason
15 Videos
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - NCERT Exemplar
5 Videos
Similar Questions
The capacities of two conductors are C1 and C2 and their respectively potentials are V1 and V2. If they are connected by a thin wire, then the loss of energy will be given by
View SolutionTwo conductors of capacitance 1μF and 2μF are charged to +10V and −20V They are now connected by a conducting wire. Find
(a) their common potential
(b) the final charges on them
(c) the loss of energy during, redistribution of charges.
View SolutionTwo capacitors of capacitances 3μF and 6μF are charged to a potential of 12V each. They are now connected to each other, with the positive plate of each joined to the negative plate of the other.
(A) the common potential will be 12V
(B) the loss in energy will be 576μJ
(c) the common potential will be 4V
(D) the loss in energy will be 144μJ
View SolutionTwo spherical conductor A and B of radii a and b (b>a) are placed concentrically in air. The two are connected by a copper wire as shown in figure. The equivalent capacitance of the system is

View SolutionTwo capacitors with capacities C1 and C2 ,are charged to potentials V1 and V2 respectively.When they are connected in parallel,the ratio of their respective charges is ....?
View SolutionA 2μF capacitor C1 is charge to a voltage 100 V and a 4μF capacitor C2 is charged to a voltage 50 V. The capacitors are then connected in parallel What is the loss of energy due to parallel connection?
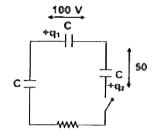
View SolutionTwo capacitor each of capacitance C=1μF each charged to potential 100 V and 50 V respectively and connected across a uncharged capacitor 'C' = 1μF as shown in the figure if switch 'S' is closed then charge in the uncharged capacitor at equilibrium will be
View SolutionA number of spherical conductors of different radii are charged to same potential. The surface charge density of each conductor is related with its radius as
View SolutionA capacitor having capacity of 2μF is charged to 200V and then the plates of the capacitor are connected to a resistance wire. The heat produced in joule will be
View SolutionThe energy stored in a capacitor of capacity C and potential V is given by
View Solution
