Write down the value of obsolute permittivity of free space.
9×109NC2/m2
8.85×10−12N−m2/C2s
8.85×10−12C2/N−m2
9×109C2/N−m2
The correct Answer is:C
To find the value of the absolute permittivity of free space (ε₀), we can follow these steps:
Step 1: Understand the Concept
The absolute permittivity of free space (ε₀) is a fundamental physical constant that describes how electric fields interact with a vacuum. It is also related to Coulomb's law and the electrostatic force between charges.
Step 2: Recall the Relationship with Coulomb's Law
Coulomb's law states that the force (F) between two point charges (Q₁ and Q₂) separated by a distance (r) is given by:
F=kQ1Q2r2
where k is the Coulomb's constant.
Step 3: Express Coulomb's Constant in Terms of ε₀
The Coulomb's constant k can also be expressed in terms of the permittivity of free space:
k=14πϵ0
Step 4: Substitute k into the Equation
From the above relationship, we can rearrange it to find ε₀:
ϵ0=14πk
Step 5: Use the Value of k
The value of Coulomb's constant k is approximately 9×109N m2/C2. Plugging this value into the equation gives:
ϵ0=14π×9×109
Step 6: Calculate ε₀
Calculating this gives:
ϵ0≈8.85×10−12C2/(N m2)
Step 7: Write the Final Answer
Thus, the value of the absolute permittivity of free space is:
ϵ0≈8.85×10−12F/m
The absolute permittivity of free space (ε₀) is a fundamental physical constant that describes how electric fields interact with a vacuum. It is also related to Coulomb's law and the electrostatic force between charges.
Coulomb's law states that the force (F) between two point charges (Q₁ and Q₂) separated by a distance (r) is given by:
F=kQ1Q2r2
where k is the Coulomb's constant.
The Coulomb's constant k can also be expressed in terms of the permittivity of free space:
k=14πϵ0
From the above relationship, we can rearrange it to find ε₀:
ϵ0=14πk
The value of Coulomb's constant k is approximately 9×109N m2/C2. Plugging this value into the equation gives:
ϵ0=14π×9×109
Calculating this gives:
ϵ0≈8.85×10−12C2/(N m2)
Thus, the value of the absolute permittivity of free space is:
ϵ0≈8.85×10−12F/m
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Check point 1.3
10 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Check point 1.4
10 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Check point 1.1
10 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
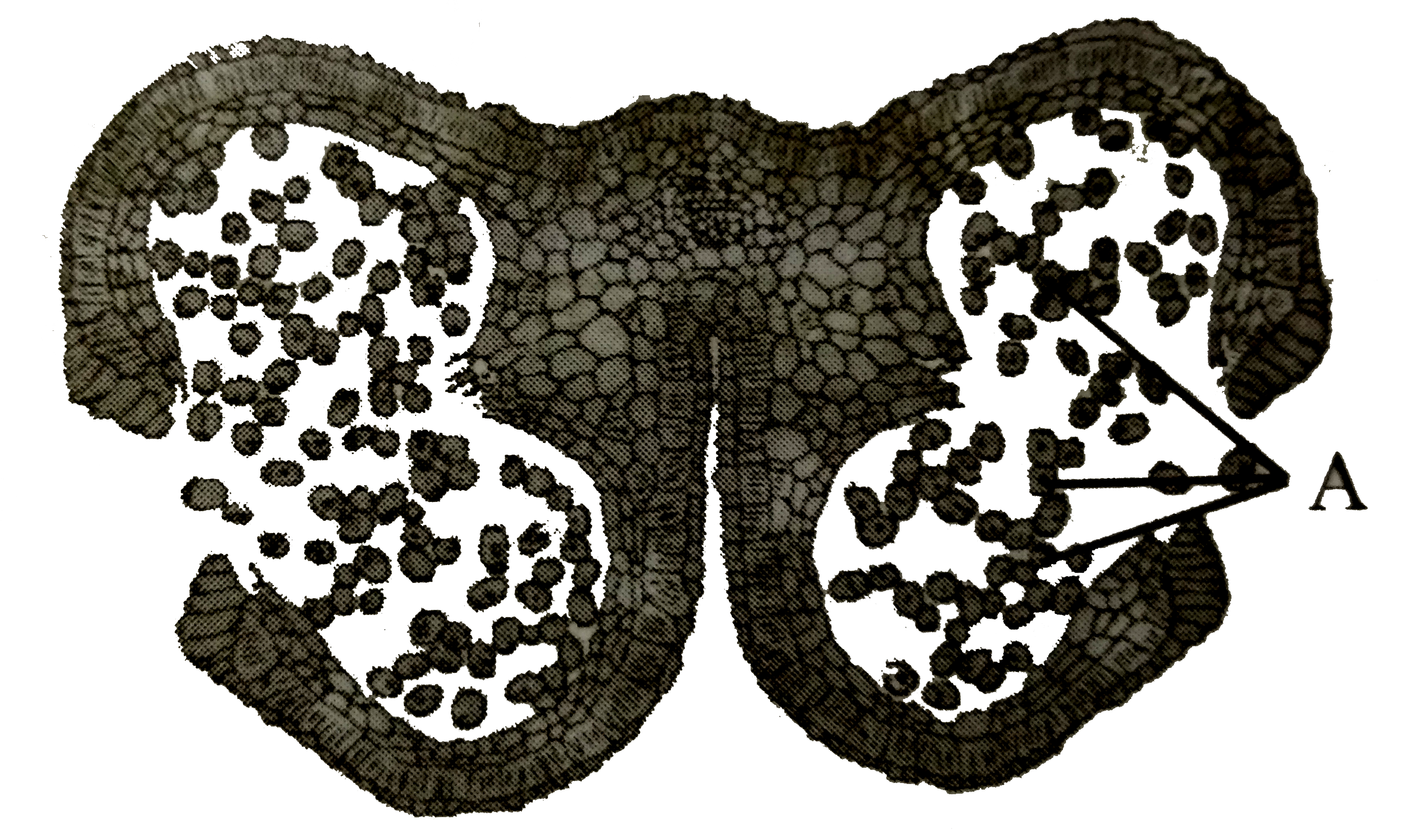
Write down the name of point A
View SolutionA parallel plate capacitor of capacitance has spacing between two plates having area The region between the plates is filled with dielectric layers, parallel to its plates, each with thickness δ=dN. The dielectric constant of the mth layer is Km=K(1+mN). For a very large N(>103), the capacitance Cisα(K∈0Adln2). Find the value of α.[∈0 is the permittivity of free space]
View Solution
Knowledge Check
The unit of permittivity of free space ε0 is:
AFaradBWeberCC2N−1m−2DC2N−1m−1
Three parallel plate air capacitors are connected in parallel. Each capacitor has plate area A3and the separation between the plates is d, 2d and 3d respectively. The equivalent capacity of combination is (ε0 = absolute permittivity of free space)
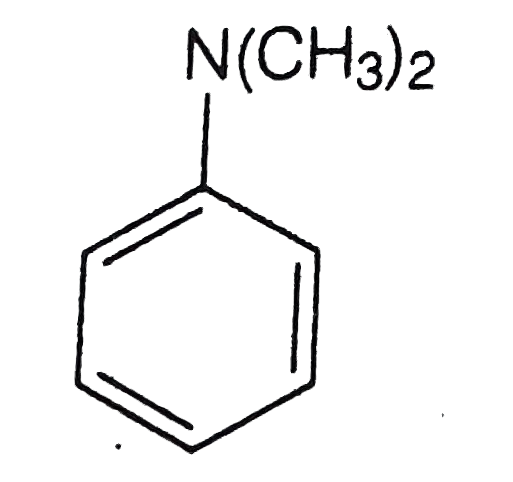
View SolutionWrite down the IUPAC name of
View SolutionAssertion : When a dielectric is placed in an electric field, the electric field with in the dielectric as well as. Potential difference across the capacitor plates are reduced by a factor K (battery is not connected).
Reason : The dielectric constant is the ratio of the absolute permittivity of the dielectric to the to the permittivity of the free space.
View SolutionThe electric field on two sides of a large charged plate is shown in figure. The charge density on the plate in SI units is given by (ε0 is the permittivity of free space in SI units).
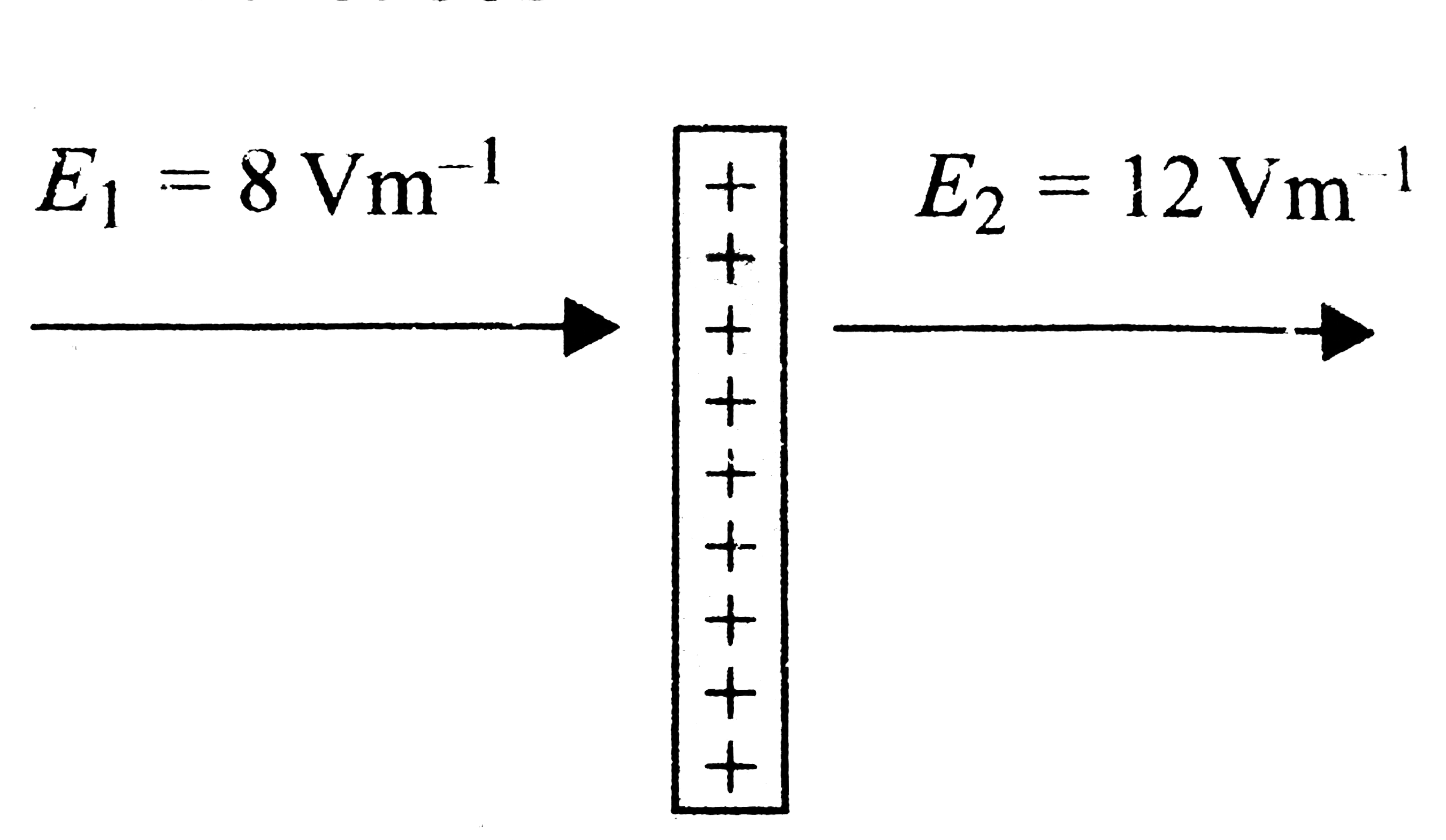
View SolutionWhich of the following does not have the dimensions of velocity ? ( Given ε0 is the permittivity of free space , μ0 is the permeability of free space , v is frequency , λ is wavelength , P is the pressure , and ρ is density , k is wave number , ω is the the angular frequency) (1)ω k (2)vλ (3)1/√ε0μ0 (4)√Pρ
View SolutionIn fiinding the electric field using Gauses law the formula ∣∣→E∣∣=qenc∈0|A| is applicable. In the Formula. ∈0 is permittivity of free space, A is the area of Gaussian surface and qenc is charge enclosed by the Gaussion surface. This equation can be used in which of the following situation?
View SolutionWrite down the smallest whole number.
View Solution
