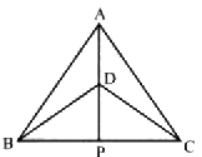
ABC and DBC are both isosceles triangles on a common base BC such that A and D lie on the same side of BC. Are triangles ADB and ADC congruent? Which condition do you use? If ∠BAC=400 and ∠BDC =1000; then find ∠ADB
To solve the problem step by step, we will analyze the given information and use the properties of congruent triangles and isosceles triangles.
Step 1: Understand the Problem
We have two isosceles triangles, △ABC and △DBC, sharing a common base BC. Points A and D are on the same side of line BC. We need to determine if triangles △ADB and △ADC are congruent and find the measure of angle ADB.
Step 2: Identify Given Angles
We are given:
- ∠BAC=40∘
- ∠BDC=100∘
Step 3: Analyze the Isosceles Triangles
1. In triangle ABC:
- Since it is isosceles, AB=AC.
- The angles opposite these sides are equal: ∠ABC=∠ACB.
2. In triangle DBC:
- Since it is isosceles, DB=DC.
- The angles opposite these sides are equal: ∠DBC=∠DCB.
Step 4: Find Angles in Triangles
1. For triangle DBC:
- The sum of angles in a triangle is 180∘.
- Therefore, ∠DBC+∠DCB+∠BDC=180∘.
- Let ∠DBC=∠DCB=x.
- Thus, x+x+100∘=180∘.
- This simplifies to 2x+100∘=180∘.
- Solving for x, we get 2x=80∘ so x=40∘.
- Therefore, ∠DBC=∠DCB=40∘.
2. For triangle ABC:
- Let ∠ABC=∠ACB=y.
- Thus, y+y+40∘=180∘.
- This simplifies to 2y+40∘=180∘.
- Solving for y, we get 2y=140∘ so y=70∘.
- Therefore, ∠ABC=∠ACB=70∘.
Step 5: Determine Congruence of Triangles
Now we check the triangles △ADB and △ADC:
- We have:
- AB=AC (sides of isosceles triangle ABC)
- DB=DC (sides of isosceles triangle DBC)
- AD is common to both triangles.
Thus, by the Side-Side-Side (SSS) congruence criterion:
- AB=AC
- DB=DC
- AD=AD
Therefore, △ADB≅△ADC.
Step 6: Find Angle ADB
Since △ADB≅△ADC, the corresponding angles are equal:
- ∠ADB=∠ADC.
Now, we know:
- ∠ABC=70∘
- ∠DBC=40∘
Using the triangle sum property in triangle ADB:
- ∠ADB+∠ABD+∠BAD=180∘
- ∠ADB+70∘+20∘=180∘ (since ∠BAD=∠BAC=40∘)
- Therefore, ∠ADB+90∘=180∘
- Thus, ∠ADB=90∘.
Final Answer
The angle ∠ADB=90∘.
---
We have two isosceles triangles, △ABC and △DBC, sharing a common base BC. Points A and D are on the same side of line BC. We need to determine if triangles △ADB and △ADC are congruent and find the measure of angle ADB.
We are given:
- ∠BAC=40∘
- ∠BDC=100∘
1. In triangle ABC:
- Since it is isosceles, AB=AC.
- The angles opposite these sides are equal: ∠ABC=∠ACB.
- Since it is isosceles, DB=DC.
- The angles opposite these sides are equal: ∠DBC=∠DCB.
1. For triangle DBC:
- The sum of angles in a triangle is 180∘.
- Therefore, ∠DBC+∠DCB+∠BDC=180∘.
- Let ∠DBC=∠DCB=x.
- Thus, x+x+100∘=180∘.
- This simplifies to 2x+100∘=180∘.
- Solving for x, we get 2x=80∘ so x=40∘.
- Therefore, ∠DBC=∠DCB=40∘.
- Let ∠ABC=∠ACB=y.
- Thus, y+y+40∘=180∘.
- This simplifies to 2y+40∘=180∘.
- Solving for y, we get 2y=140∘ so y=70∘.
- Therefore, ∠ABC=∠ACB=70∘.
Now we check the triangles △ADB and △ADC:
- We have:
- AB=AC (sides of isosceles triangle ABC)
- DB=DC (sides of isosceles triangle DBC)
- AD is common to both triangles.
- AB=AC
- DB=DC
- AD=AD
Since △ADB≅△ADC, the corresponding angles are equal:
- ∠ADB=∠ADC.
- ∠ABC=70∘
- ∠DBC=40∘
- ∠ADB+∠ABD+∠BAD=180∘
- ∠ADB+70∘+20∘=180∘ (since ∠BAD=∠BAC=40∘)
- Therefore, ∠ADB+90∘=180∘
- Thus, ∠ADB=90∘.
The angle ∠ADB=90∘.
Topper's Solved these Questions
Similar Questions
ABC and DBC are two isosceles triangles on the same bas BC and vertices A and D are on the same side of BC . If AD is extended to intersect BC at P, show that ABD ≅ACD (ii) ABP ≅ACP
View SolutionABC and DBC are two isosceles triangles on the same bas BC and vertices A and D are on the same side of BC . If AD is extended to intersect BC at P, show that AP bisects \ ∠A as well as ∠D AP is the perpendicular bisector of BC
View SolutionABC is a triangle. D is a point on AB such that D=14AB and E is a point on AC such that AE=14AC. Prove that DE=14BC
View SolutionIn Fig. 71, if ABCD and CDEF, find ∠ACE
View SolutionABC and DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC . If AD is extended to intersect BC at P, show that ABD≅ACD (ii) ABP≅ACP AP bisects ∠A as well as ∠D AP is perpendicular bisector of BC.
 View Solution
View SolutionD is a point on the side BC of ABC. A line PDQ , though D , meets side AC in P and AB produced at Q . If ∠A=800, ∠ABC=600 and ∠PDC=150 find (i) ∠AQD (ii) ∠APD.
View SolutionThe diagonals of quadrilateral ABCD,ACandBD intersect in O. Prove that if BO=OD, the triangles ABCandADC are equal in area.
View SolutionIn Figure, BM and DN are both perpendiculars to the segments AC and BM=DN . Prove that AC bisects BD
 View Solution
View Solutionp AND q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that ar(APB)=ar (BQC).
 View Solution
View SolutionIf ABC is an isosceles triangle such that AB=AC and AD is an altitude from A on BC . Prove that (i) ∠B=∠C (ii) AD bisects BC (iii) AD bisects ∠A
View Solution

