A plano-convex lens is silvered on its plane side. The radius of curvature of the other face is 12cm and the refractive index of the material of the lens is 1.5. An object is placed 24 cm in front of the silvered lens. Where will the image be formed?
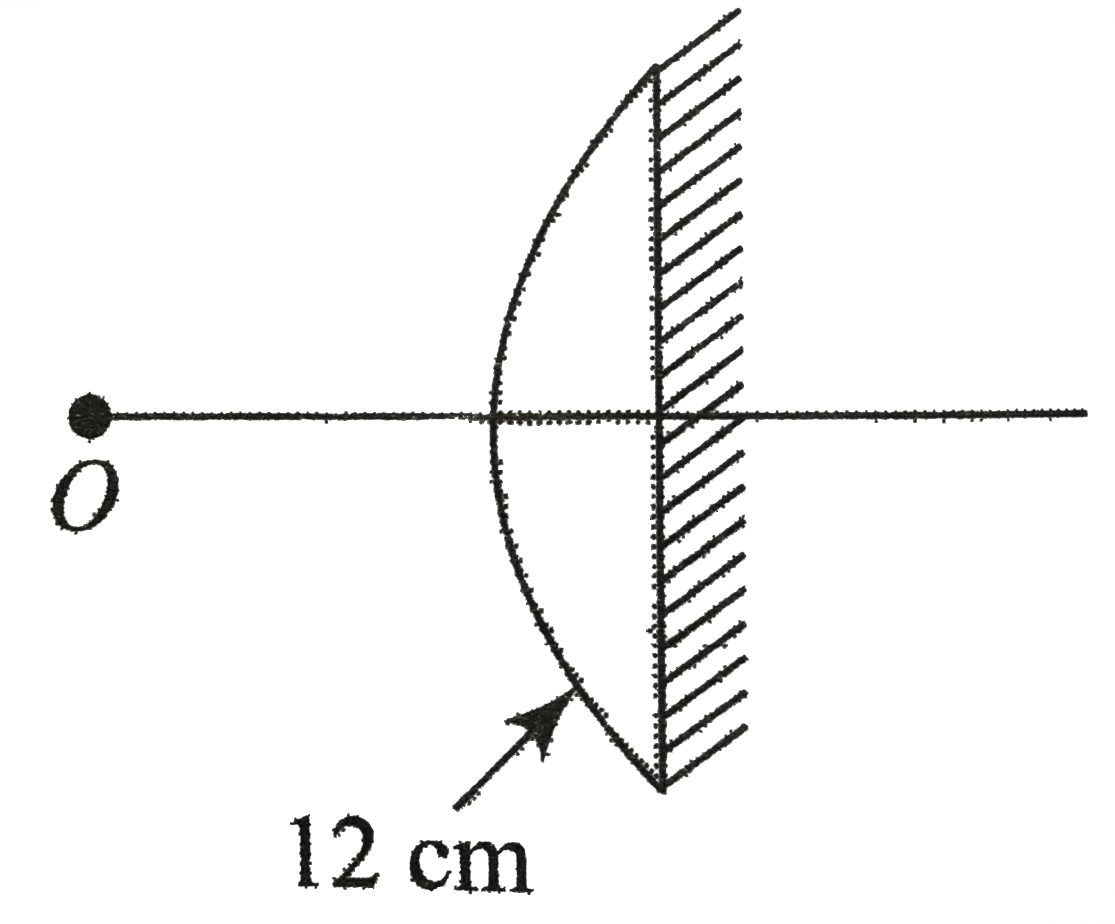
$A plano-convex lens is silvered on its plane side. The radius of curvature of the other face is 12cm and the refractive index of the material of the lens is 1.5. An object is placed 24 cm in front of the silvered lens. Where will the image be formed?$

Video Solution

Text Solution

Verified by Experts

Here, $R_{1} = + 12 c m, R_{2} = \infty, μ_{p} = 1.5, μ_{a} = 1,$ therefore
Focal length of the lens, $\frac{1}{f_{1}} = (1.5 - 1) [\frac{1}{12} - \frac{1}{\infty}]$
or $f_{1} = 24 c m$
The plane side of the lens is silvered, focal length of the mirror
$f_{m} = \frac{R_{2}}{2} = \frac{\infty}{2} = \infty$
Therefore, effective focal length of the silvered lens is
$\frac{1}{f_{e}} = \frac{1}{f_{m}} - \frac{2}{f_{1}} or f_{e} = - 12 c m$
The object is placed 24 cm in front of the lens.
$u = - 24 c m, f = - 12 c m$
And from the mirror Eq. (i), we have
$\frac{1}{v} + \frac{1}{u} = \frac{1}{f}$ (i)
or $v = - 24 c m$
The final image is formed on the object itself. The behavior is like that of a concave mirror.

Updated on:21/07/2023