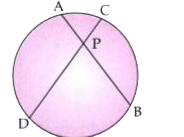
AB and CD are two equal chords of a circle with centre O which intersect each other at right angle at P. If OM ⊥ AB and ON ⊥ CD, show that OMPN is a square.
To show that OMPN is a square, we will follow these steps:
Step 1: Understand the Given Information
We are given that AB and CD are two equal chords of a circle with center O. They intersect each other at point P at right angles (90 degrees). OM is perpendicular to AB and ON is perpendicular to CD.
Step 2: Establish the Perpendicular Relationships
Since OM is perpendicular to AB and ON is perpendicular to CD, we can conclude that:
- OM⊥AB
- ON⊥CD
Step 3: Show that AB is Perpendicular to CD
Since OM is perpendicular to AB and ON is perpendicular to CD, we can infer that:
- AB∥ON
- CD∥OM
This means that AB and CD are perpendicular to each other:
- AB⊥CD
Step 4: Identify the Properties of the Quadrilateral OMPN
Now, since:
- MP lies on AB, therefore MP is parallel to ON.
- PN lies on CD, therefore PN is parallel to OM.
This implies:
- MP∥ON
- PN∥OM
Since both pairs of opposite sides are parallel, OMPN is a parallelogram.
Step 5: Show that OMPN is a Rectangle
In parallelogram OMPN, we know:
- ∠ONP=90∘ (given)
- ∠OMP=90∘ (given)
Since both angles are 90 degrees, OMPN is a rectangle.
Step 6: Show that OMPN is a Square
Since AB and CD are equal chords, the distances from the center O to the chords are equal:
- OM=ON
In a rectangle, if the adjacent sides are equal, it is a square. Therefore:
- OM=ON
Thus, OMPN is a square.
Conclusion
We have shown that OMPN is a square based on the properties of the chords, the perpendicular relationships, and the equal lengths from the center to the chords.
---
We are given that AB and CD are two equal chords of a circle with center O. They intersect each other at point P at right angles (90 degrees). OM is perpendicular to AB and ON is perpendicular to CD.
Since OM is perpendicular to AB and ON is perpendicular to CD, we can conclude that:
- OM⊥AB
- ON⊥CD
Since OM is perpendicular to AB and ON is perpendicular to CD, we can infer that:
- AB∥ON
- CD∥OM
- AB⊥CD
Now, since:
- MP lies on AB, therefore MP is parallel to ON.
- PN lies on CD, therefore PN is parallel to OM.
- MP∥ON
- PN∥OM
In parallelogram OMPN, we know:
- ∠ONP=90∘ (given)
- ∠OMP=90∘ (given)
Since AB and CD are equal chords, the distances from the center O to the chords are equal:
- OM=ON
- OM=ON
We have shown that OMPN is a square based on the properties of the chords, the perpendicular relationships, and the equal lengths from the center to the chords.
Topper's Solved these Questions
Similar Questions
AB and CD are two equal chords of a circle with centre O which intersect each other at right angle at point P. If OM ⊥ AB and ON ⊥ CD, show that OMPN is a square.
View SolutionIn the figure, two equal chords AB and CD of a circle with centre O, intersect each other at E, Prove that AD=CB
View SolutionTwo equal chord AB and CD of a circle with centre O, intersect each other at point P inside the circle.

Prove that:
BP=DPView SolutionAB and CD are two chords of a circle intersecting at P. Prove that AP×PB=CP×PD
 View Solution
View SolutionChords AB and CD of a circle with centre O, intersect at a point E. If OE bisects angle AED, prove that chord AB = chord CD.
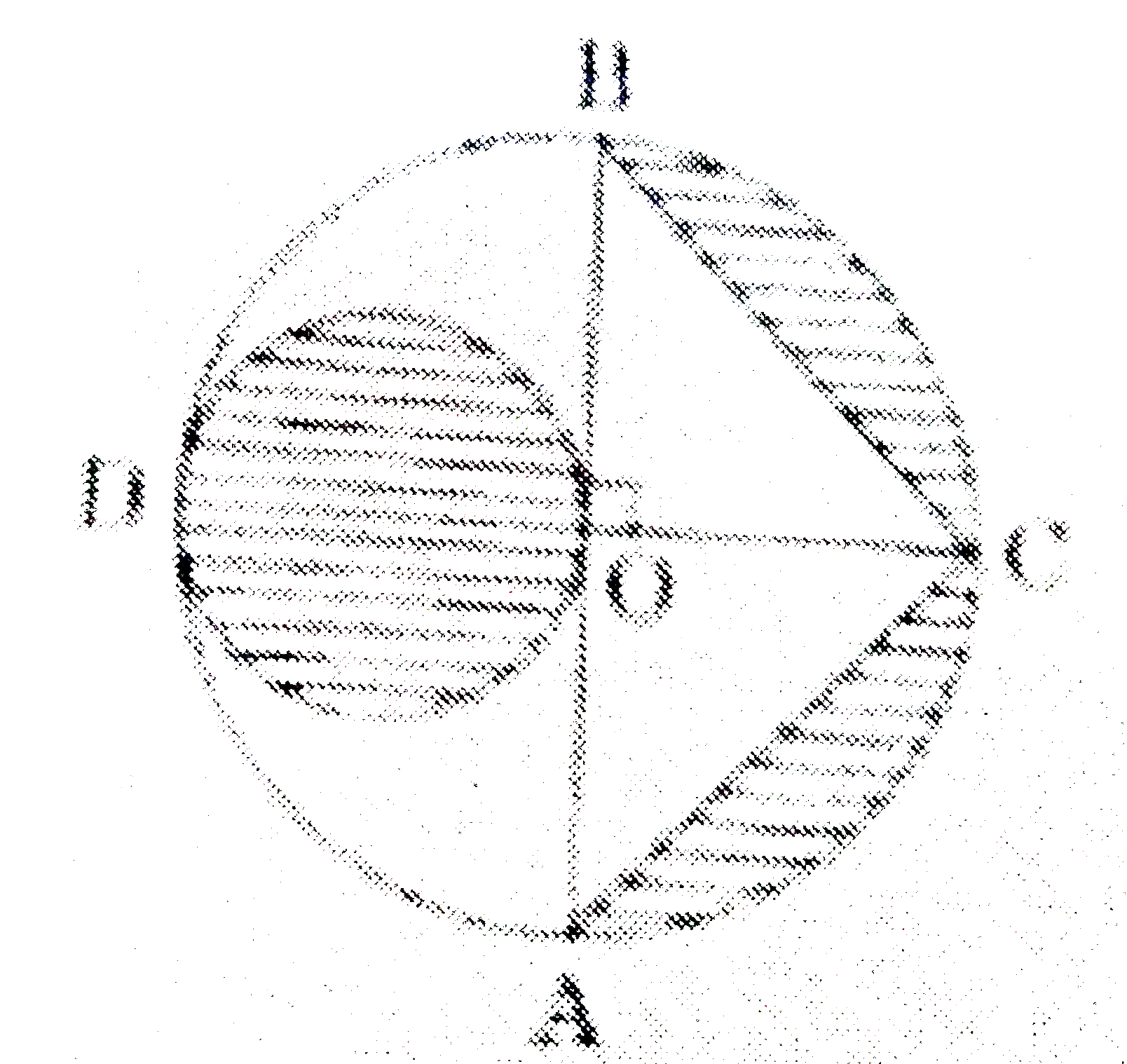
View SolutionIn Fig. 12.27, AB and CD are two diameters of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. IfOA=7 cm, find the area of the shaded region
View SolutionIn Fig. 12.27, AB and CD are two diameters of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. IfOA=7 cm, find the area of the shaded region
View SolutionIn Figure -4 AB, and CD are two diameters of a circle with centre O perpendiclualr to each other and OD is the diameter of the circle . If OA = 7 cm , then find the carea of the shaded region .
 View Solution
View SolutionIn the given figure, AB and CD are two equal chords of a circle, with centre O. If P is the mid-point of chord AB, Q is the mid-point of chord CD and ∠POQ=150∘ find angleAPQ
View SolutionThe equal chords AB and CD of circle with centre O, when produced, meet at P outside the circle. Prove that :
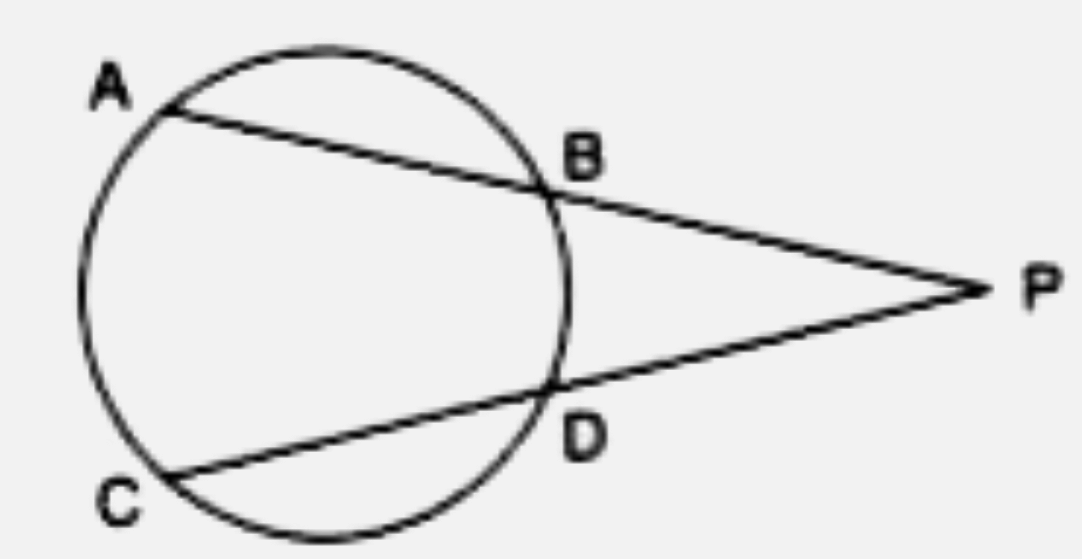
PB=PDView Solution

