AB is a vertical pole resting at the end A on the level ground. P is a point on the level ground such that AP = 3AB and C Is the midpoint of AB. If AC and CB subtend angles α and β, respectively, at P, then the value of tanβ is Solution in Bengali
1819
319
16
13
On the basis of the given information , following diagram is obtained .
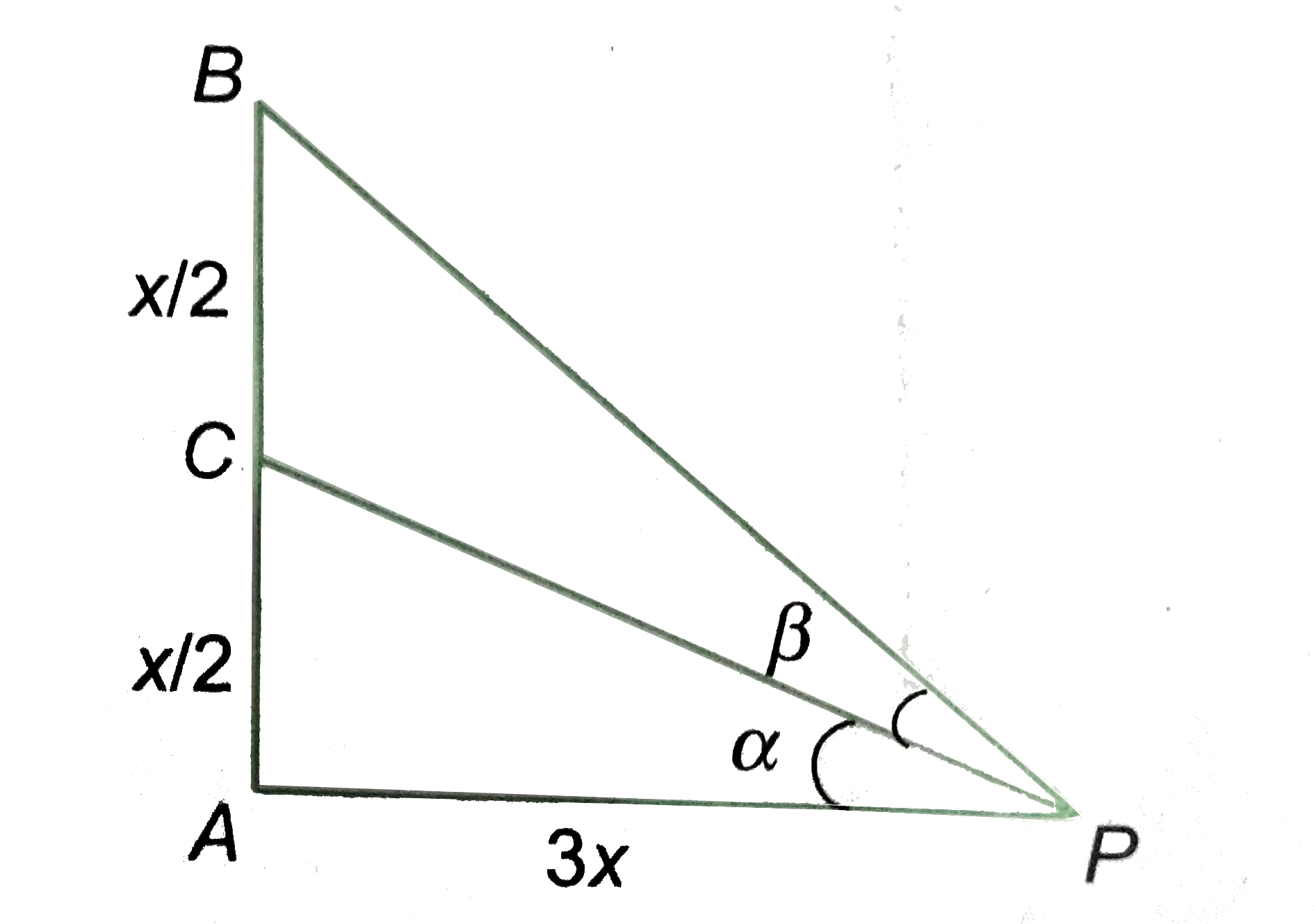
tanβ=tan((α+β)−α)
=13−161+13.16=319
Topper's Solved these Questions
- HIGHT AND DISTANCE BOOK - CENGAGE PUBLICATIONCHAPTER - HIGHT AND DISTANCE EXERCISE - Archives
3 Videos
- HIGHT AND DISTANCE BOOK - CENGAGE PUBLICATIONCHAPTER - HIGHT AND DISTANCE EXERCISE - Archives
3 Videos
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercises
22 Videos
- HYPERBOLABOOK - CENGAGE PUBLICATIONCHAPTER - HYPERBOLAEXERCISE - COMPREHENSION TYPE
2 Videos
Similar Questions
A bird is sitting on the top of a vertical pole 20 m high and its elevation from a point O on the ground is 45o . It flies off horizontally straight away from the point O. After one second, the elevation of the bird from O is reduced to 30o . Then the speed (in m/s) of the bird is (1) 40(√2−1) (2) 40(√3−2) (3) 20√2 (4) 20(√3−1)
View SolutionA bird is sitting on the top of a vertical pole 20 m high and its elevation from a point O on the ground is 45o . It flies off horizontally straight away from the point O. After one second, the elevation of the bird from O is reduced to 30o . Then the speed (in m/s) of the bird is (1) 40(√2−1) (2) 40(√3−2) (3) 20√2 (4) 20(√3−1)
View SolutionDraw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
View SolutionThe circle x2+y2−8x=0 and hyperbola x29−y24=1 I intersect at the points A and B. Equation of a common tangent with positive slope to the circle as well as to the hyperbola is (A) 2x−√5y−20=0 (B) 2x−√5y+4=0 (C) 3x−4y+8=0 (D) 4x−3y+4=0
View SolutionAB is a vertical pole resting at the end A on the level ground. P is a point on the level ground such that AP = 3AB and C Is the midpoint of AB. If AC and CB subtend angles α and β, respectively, at P, then the value of tanβ is
View SolutionLet a vertical tower AB have its end A on the level ground. Let C be the mid-point of AB and P be a point on the ground such that AP = 2AB. If √BPC=β, then tan β is equal to
View SolutionAB is a vertical pole. The end A is on the level ground .C is the middle point of AB. P is a point on the level ground . The portion BC subtends an angles beta at P. If AP = nAB, then tan beta =
View SolutionAB is a vertical pole and C is its mid point. The end A is on the level ground and P is any point on the level ground other than A the portion CB substends an angle β at P. If AP:AB=2:1, then β=
View SolutionA vertical pole subtends an angle Tan−1(12) at a point P on the ground. IF the angles subtended by the upper half and the lower half of the pole at P are respectively αandβ, then (tanα,tanβ)=
View SolutionAB एक ऊर्ध्वाधर खम्भा है | सिरा 'A' एक समतल मैदान पर है | C, AB का मध्य - बिन्दु है | समतल मैदान पर एक बिन्दु P है | भाग CB, बिन्दु P पर कोण β बनाता है | यदि AP = nAB तो दिखाइए कि tanβ=n2n2+1
View Solutionমনে করো, AB একটি টাওয়ার যার A প্রান্তটি ভূমির ওপর অবস্থিত। মনে করো, C,AB এর মধ্যবিন্দু এবং ভূমির ওপর অবস্থিত P একটি বিন্দু যেখানে AP=2AB। যদি ∠BPC=β হয়, তাহলে tanβ=
View Solutionमाना एक ऊर्ध्वाधर मीनार AB ऐसी हैं कि उसका सिरा A भूमि पर हैं। माना AB का मध्य-बिन्दु C हैं तथा भूमि पर स्थिर बिन्दु P ऐसा हैं कि AP = 2 AB| यदि ∠BPC=β हैं, तो tanβ बराबर हैं
View SolutionAB is a vertical pole resting at the end A on the level ground. P is a point on the level ground such that AP = 3AB and C Is the midpoint of AB. If AC and CB subtend angles α and β, respectively, at P, then the value of tanβ is
View SolutionAB is a vertical pillar that stands on the earth surface A. Another point on the earth surface is p such that AP = 3AB if the midpoint of AB is C and on CB. beta Who transfers? tan beta The value of.
View SolutionA vertical pole subtends an angle Tan−1(12) at a point P on the ground. On the ground .If the angles subtends by the upper half and the lower half of the pole at P are respectively αandβ then (tanα,tanβ)
View SolutionAB एक ऊर्ध्वाधर मीनार है बिंदु A जमीन पर है और C,AB का मध्य बिंदु है । भाग CB जमीन पर एक बिंदु P पर α कोण अंतरित करता है। यदि AP=nAB है तब सही संबंध है
View Solution
