Find the area bounded by the curve x2=4y and the straight line x=4y−2.
The correct Answer is:98 sq. units
Given curves x2=4yandx=4y−2 intersect, when
x2=x+2
or x2−x−2=0
or x=2,−1
⇒ y=1,1/4
Hence, points of intersection area A(−1,1/4),B(2,1)
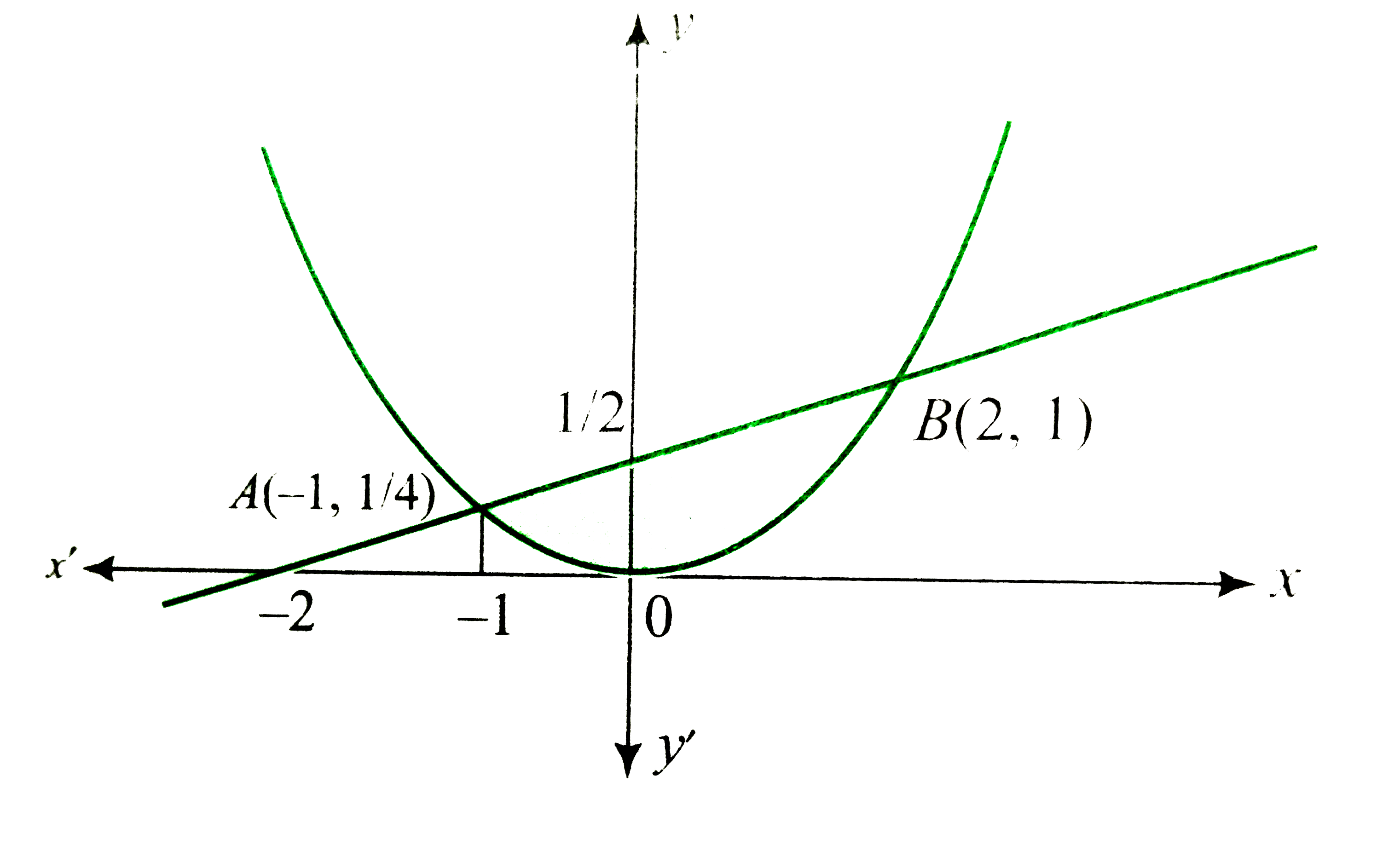
Required area = Shaded region in the figure
=∫2−1(x+24−x24)dx
=14[x22+2x−x33]2−1
=14[(2+4−83)−(12−2+13)]
=14[103−(−76)]=14[276]=9/8 sq. units.
Topper's Solved these Questions
- AREABOOK - CENGAGE ENGLISHCHAPTER - AREAEXERCISE - Concept Application Exercise 9.3
7 Videos
- AREABOOK - CENGAGE ENGLISHCHAPTER - AREAEXERCISE - Exercises - Single Correct Answer Type
40 Videos
- AREABOOK - CENGAGE ENGLISHCHAPTER - AREAEXERCISE - Concept Application Exercise 9.1
9 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGE ENGLISHCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Comprehension Type
5 Videos
- BINOMIAL THEOREMBOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Matrix
4 Videos
Similar Questions
The area of the region bounded by the curve x2=4y and the straight line x=4y−2 is
View SolutionThe area of the region bounded by the curve x2=4y and the straight line x=4y−2 is
View SolutionThe area of the region bounded by the curve x2=4y and the straight line x=4y−2 is
View SolutionFind the area bounded by the curves y=2x−x2 and the straight line y=−x.
View SolutionFind the area bounded by the parabola y2=4x and the straight line x+y=3.
View SolutionThe area bounded by the curve x2=4ay and the line y=2a is
View SolutionFind the area bounded by the parabola y=x2+1 and the straight line x+y=3.
View SolutionFind the area bounded by the parabola y=x2+1 and the straight line x+y=3.
View SolutionFind the area bounded by the parabola y=x2+1 and the straight line x+y=3.
View SolutionFind the area bounded by the curves x+2|y|=1 and x=0 .
View Solution
