Find the volume of the solid generated by revolving about the x-axis the figure bounded by the straight lines .
To find the volume of the solid generated by revolving the region bounded by the lines y=x+1, y=2x+1, and x=2 about the x-axis, we will follow these steps:
Step 1: Find the points of intersection of the lines
We need to find the points where the lines y=x+1 and y=2x+1 intersect.
Set the equations equal to each other:
x+1=2x+1
Subtract x+1 from both sides:
0=x
Thus, the lines intersect at x=0.
Step 2: Determine the region of integration
Next, we need to find the x-coordinates where the lines intersect the vertical line x=2.
1. For y=x+1 at x=2:
y=2+1=3
So, the point is (2,3).
2. For y=2x+1 at x=2:
y=2(2)+1=5
So, the point is (2,5).
Step 3: Set up the volume integral
The volume V of the solid of revolution about the x-axis can be found using the formula:
V=π∫ba(f(x)2−g(x)2)dx
where f(x) is the upper function and g(x) is the lower function in the interval (a,b).
In our case:
- f(x)=2x+1 (the upper line)
- g(x)=x+1 (the lower line)
The limits of integration are from x=0 to x=2.
Step 4: Write the volume integral
Now, we can write the integral:
V=π∫20((2x+1)2−(x+1)2)dx
Step 5: Expand the integrand
Expanding the squares:
(2x+1)2=4x2+4x+1
(x+1)2=x2+2x+1
Now, substituting back into the integral:
V=π∫20((4x2+4x+1)−(x2+2x+1))dx
Simplifying the integrand:
4x2+4x+1−x2−2x−1=3x2+2x
Thus, we have:
V=π∫20(3x2+2x)dx
Step 6: Integrate
Now we can integrate:
V=π(33x3+22x2)20=π(x3+x2)20
Calculating the limits:
=π((23+22)−(03+02))=π(8+4)=12π
Conclusion
The volume of the solid generated by revolving the region about the x-axis is:
12π
We need to find the points where the lines y=x+1 and y=2x+1 intersect.
x+1=2x+1
Subtract x+1 from both sides:
0=x
Thus, the lines intersect at x=0.
Next, we need to find the x-coordinates where the lines intersect the vertical line x=2.
y=2+1=3
So, the point is (2,3).
y=2(2)+1=5
So, the point is (2,5).
The volume V of the solid of revolution about the x-axis can be found using the formula:
V=π∫ba(f(x)2−g(x)2)dx
where f(x) is the upper function and g(x) is the lower function in the interval (a,b).
- f(x)=2x+1 (the upper line)
- g(x)=x+1 (the lower line)
Now, we can write the integral:
V=π∫20((2x+1)2−(x+1)2)dx
Expanding the squares:
(2x+1)2=4x2+4x+1
(x+1)2=x2+2x+1
Now, substituting back into the integral:
V=π∫20((4x2+4x+1)−(x2+2x+1))dx
Simplifying the integrand:
4x2+4x+1−x2−2x−1=3x2+2x
Thus, we have:
V=π∫20(3x2+2x)dx
Now we can integrate:
V=π(33x3+22x2)20=π(x3+x2)20
Calculating the limits:
=π((23+22)−(03+02))=π(8+4)=12π
The volume of the solid generated by revolving the region about the x-axis is:
12π
Topper's Solved these Questions
- BOOK - IA MARONCHAPTER - APPLICATIONS OF THE DEFINITE INTEGRALEXERCISE - Computing Pressure, Work and Other Physical Quantities by the Definite Integrals7videos
APPLICATIONS OF THE DEFINITE INTEGRAL
- BOOK - IA MARONCHAPTER - APPLICATIONS OF THE DEFINITE INTEGRALEXERCISE - Computing Static Moments and Moments of Inertia. Determining Coordinates of the Centre of Gravity15videos
APPLICATIONS OF THE DEFINITE INTEGRAL
- BOOK - IA MARONCHAPTER - APPLICATIONS OF THE DEFINITE INTEGRALEXERCISE - Area of Surface of Revolution10videos
APPLICATIONS OF THE DEFINITE INTEGRAL
- BOOK - IA MARONCHAPTER - APPLICATION OF DIFFERENTIAL CALCULUS TO INVESTIGATION OF FUNCTIONSEXERCISE - ADDITIONAL PROBLEMS9videos
APPLICATION OF DIFFERENTIAL CALCULUS TO INVESTIGATION OF FUNCTIONS
- BOOK - IA MARONCHAPTER - BASIC CLASSES OF INTEGRABLE FUNCTIONS EXERCISE - 5.8 INTEGRATION OF OTHER TRANSCENDENTAL FUNCTIONS5videos
BASIC CLASSES OF INTEGRABLE FUNCTIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
The volume of solid generated by revolving about the y-axis the figure bounded by the parabola y=x2andx=y2 is
A215πB245πC215πD524π
Compute the volume of the solid generated by revolving about the x-axis the figure bounded by the parabola y=0.25x2+2 and the straight line 5x−8y+14=0
View SolutionFind the volume of the solid generated by revolving about the line y=−2a the figure bounded by the parabola y2=4ax and the straight line x=a
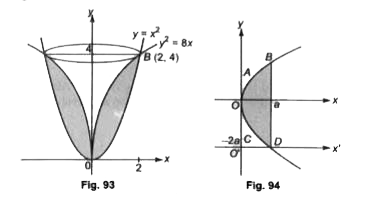 View Solution
View SolutionCompute the volume of the solid generated by revolving about the x-axis the area bounded by the axes of coordinates and the parabola x12+y12=a12.
View SolutionCompute the area of the figure bounded by the lines y=x+1,y=cosx and the x-axis
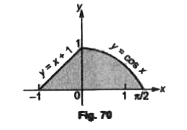 View Solution
View SolutionFind the volume of the solid generated by revolving, about the x-axis, the infinite branch of the curve y=2(1x−1x2)forx≥1.
View SolutionFind the area of the surface generated by revolving about the x-axis a closed contour OABCO formed by the curves y=x2andx=y2
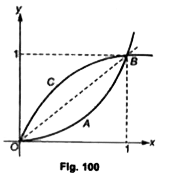 View Solution
View SolutionCompute the area of the figure bounded by the straight lines x=0, x=2 and the curves y=2x,y=2x−x2
View Solution

