Question

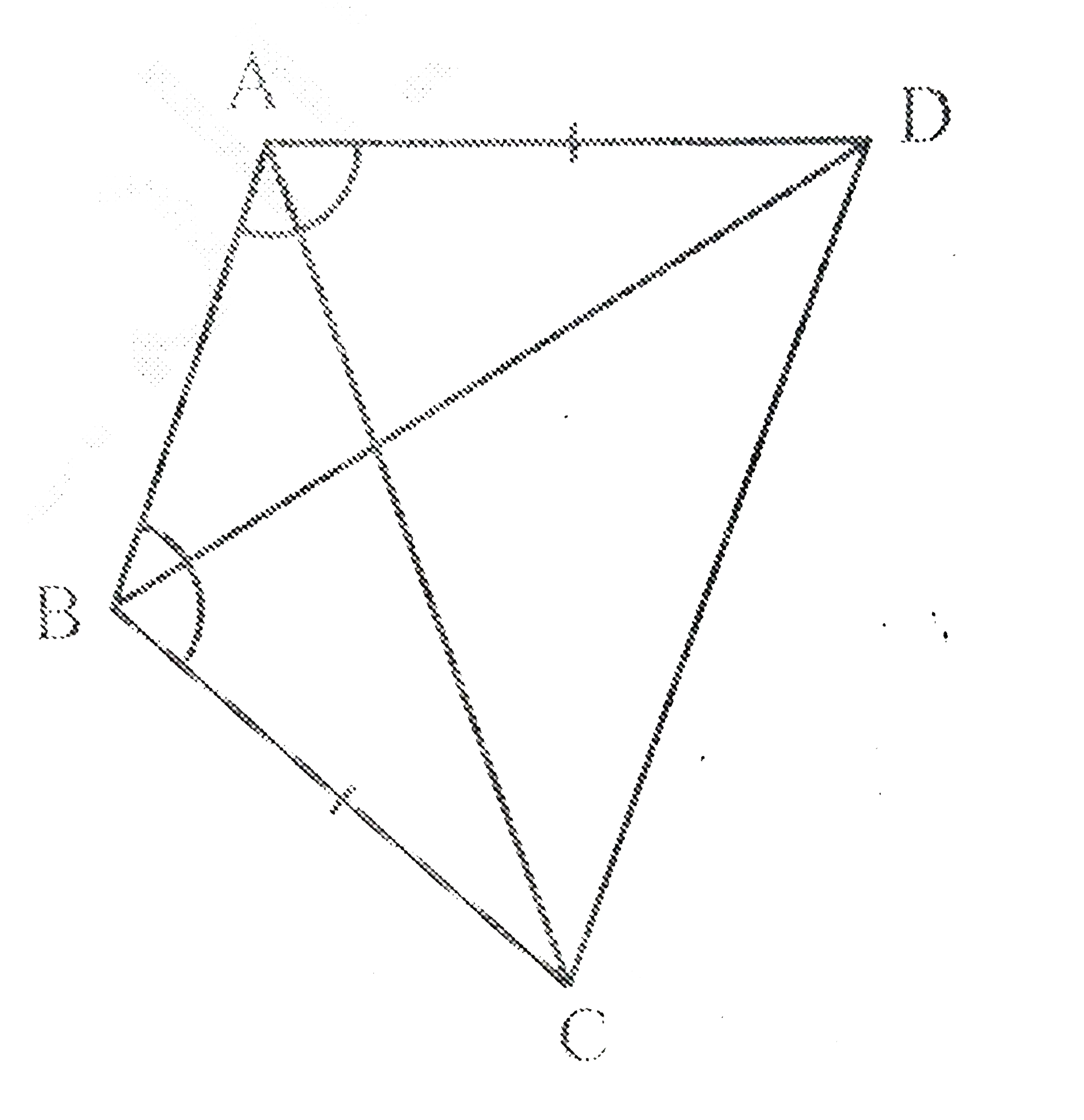
If $ABCD$
is a quadrilateral in which $ABCD$
and $AD=BC,$
prove that $\angle A$
=$\angle B$.

Explanation

To prove that ∠A = ∠B in the quadrilateral ABCD where AB is parallel to CD and AD = BC, we can follow these steps:

Step 1: Identify the given information
We are given that:
- AB is parallel to CD (AB || CD)
- AD = BC

Step 2: Draw a line parallel to AD
Draw a line CE parallel to AD. This means that CE || AD.

Step 3: Establish relationships using parallel lines
Since AB || CD and CE || AD, we can identify the following angles:
- ∠ABE and ∠CDE are corresponding angles, so ∠ABE = ∠CDE.
- ∠A and ∠E are co-interior angles, so ∠A + ∠E = 180°.

Step 4: Use the property of triangles
In triangle BCE, since AD = BC, we have:
- BC = AD
Thus, triangle BCE is isosceles, which means:
- ∠BCE = ∠CBE.

Step 5: Relate angles
From the isosceles triangle BCE, we have:
- ∠BCE = ∠CBE (let's denote this angle as x).

Step 6: Set up the equation
Now, we know that:
- ∠A + ∠E = 180° (from step 3)
- ∠E = 180° - ∠A (from the above equation)
- Therefore, we can write: ∠CBE + ∠B = 180° (since they are a linear pair).

Step 7: Substitute and simplify
Substituting the values:
- x + ∠B = 180° (where x = ∠CBE)
- Also, from step 4, we have x = 180° - ∠A.

Step 8: Equate and conclude
Setting the two expressions for x equal gives us:
- 180° - ∠A = 180° - ∠B
This simplifies to:
- ∠A = ∠B.

Conclusion
Thus, we have proved that ∠A = ∠B.

---