If from any point on the common chord of two intersecting circles, tangents be drawn to the circles, prove that they are equal.
To prove that the tangents drawn from any point on the common chord of two intersecting circles are equal, we can follow these steps:
Step 1: Understand the Configuration
Let the two intersecting circles be denoted as Circle 1 and Circle 2. Let the points of intersection of these circles be A and B. The line segment AB is the common chord of the two circles. Let P be any point on the common chord AB.
Step 2: Draw Tangents
From point P, draw tangents PM to Circle 1 and PN to Circle 2. We need to prove that PM = PN.
Step 3: Use the Tangent-Secant Theorem
According to the tangent-secant theorem, if a tangent is drawn from an external point to a circle, the square of the length of the tangent segment is equal to the product of the lengths of the whole secant segment and its external part.
For Circle 1:
- Let the points where the secant (line through P) intersects Circle 1 be A and B.
- According to the theorem, we have:
PM2=PA⋅PB
For Circle 2:
- Let the points where the secant intersects Circle 2 also be A and B.
- Similarly, we have:
PN2=PA⋅PB
Step 4: Equate the Two Results
From the two equations derived from the tangent-secant theorem, we have:
PM2=PA⋅PB
PN2=PA⋅PB
Since both PM² and PN² are equal to PA · PB, we can conclude:
PM2=PN2
Step 5: Conclude the Proof
Taking the square root of both sides, we find:
PM=PN
Thus, we have proved that the lengths of the tangents drawn from point P to both circles are equal.
Let the two intersecting circles be denoted as Circle 1 and Circle 2. Let the points of intersection of these circles be A and B. The line segment AB is the common chord of the two circles. Let P be any point on the common chord AB.
From point P, draw tangents PM to Circle 1 and PN to Circle 2. We need to prove that PM = PN.
According to the tangent-secant theorem, if a tangent is drawn from an external point to a circle, the square of the length of the tangent segment is equal to the product of the lengths of the whole secant segment and its external part.
- Let the points where the secant (line through P) intersects Circle 1 be A and B.
- According to the theorem, we have:
PM2=PA⋅PB
- Let the points where the secant intersects Circle 2 also be A and B.
- Similarly, we have:
PN2=PA⋅PB
From the two equations derived from the tangent-secant theorem, we have:
PM2=PA⋅PB
PN2=PA⋅PB
PM2=PN2
Taking the square root of both sides, we find:
PM=PN
Thus, we have proved that the lengths of the tangents drawn from point P to both circles are equal.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Number of tangents drawn from a point inside the circle is :
A1B2C4D0
Prove that the length of the tangents drawn from an external point to a circle are equal.
View SolutionProve that the lengths of tangents drawn from an external point to a circle are equal.
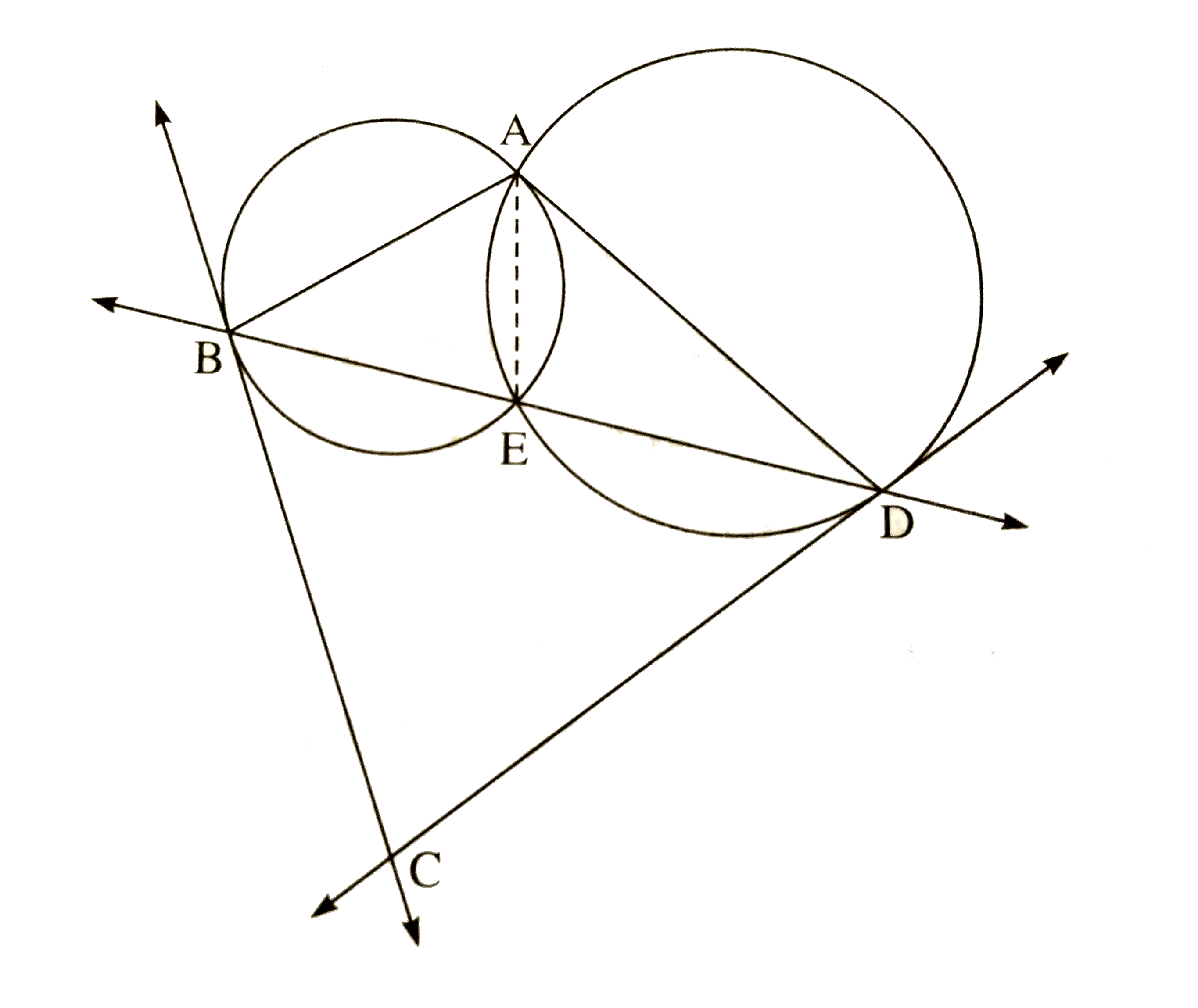
View SolutionIn figure, two circles intersect each other at points A and E. Their common secant through E intersects the circles at points B and D. The tangents of the circles at points B and D intersect each other at point C. Prove that □ ABCD is cyclic.
View SolutionTheorem: The length of two tangents drawn from an external point to a circle are equal.
View SolutionPA and PB are the two tangents drawn on a circle from and external point P. The centre of the circle is O and the tangents touches the circles at points A and B. Then quadrilateral OAPB is-
View SolutionPerpendiculars are drawn, respectively, from the points PandQ to the chords of contact of the points QandP with respect to a circle. Prove that the ratio of the lengths of perpendiculars is equal to the ratio of the distances of the points PandQ from the center of the circles.
View SolutionIn the adjoining figure, common tangents AB and CD to two circles intersect at P.
Prove that AB=CD.

View Solution
