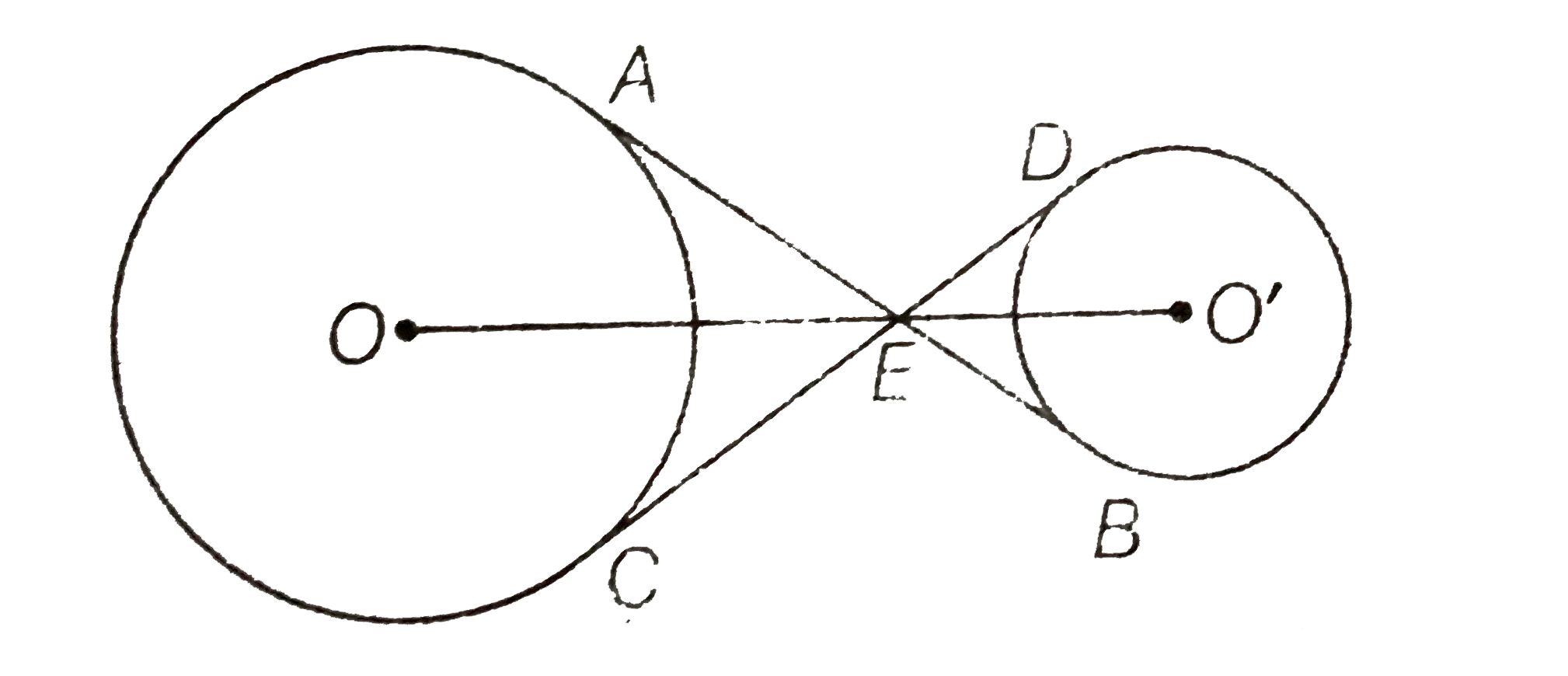
In Figure, two congruent circles with centers O and O' intersect at A and B. If ∠AO′B=500, then find ∠APB
two congruent circles with centers O and O'
intersect at A and B.
∠AOB=500
OA=AB=O'A=O'B(radii of congruent circles)
OAO'B is a rhombus
∠AOB=∠AO'B=500
Now, arc AB subtends ∠AOB
at the Centre and ∠APB
at the remaining part of the circle.
∠APB=12∠AOB=12×500=250
Topper's Solved these Questions
Similar Questions
In Figure, two congruent circles with centers O and O' intersect at A and B. If ∠AO′B=500, then find ∠APB
View SolutionThe congruent circles with centres Oand O' intersect at two points A and B. Then, ∠AOB=∠AO'B.
View SolutionIn the figure, two congruent circles intersect in A and B. If ∠AO'B=50∘ then find ∠APB
View SolutionIn a figure the common tangents, AB and CD to two circles with centers O and O' intersect at E. Prove that the points O, E and O' are collinear.
 View Solution
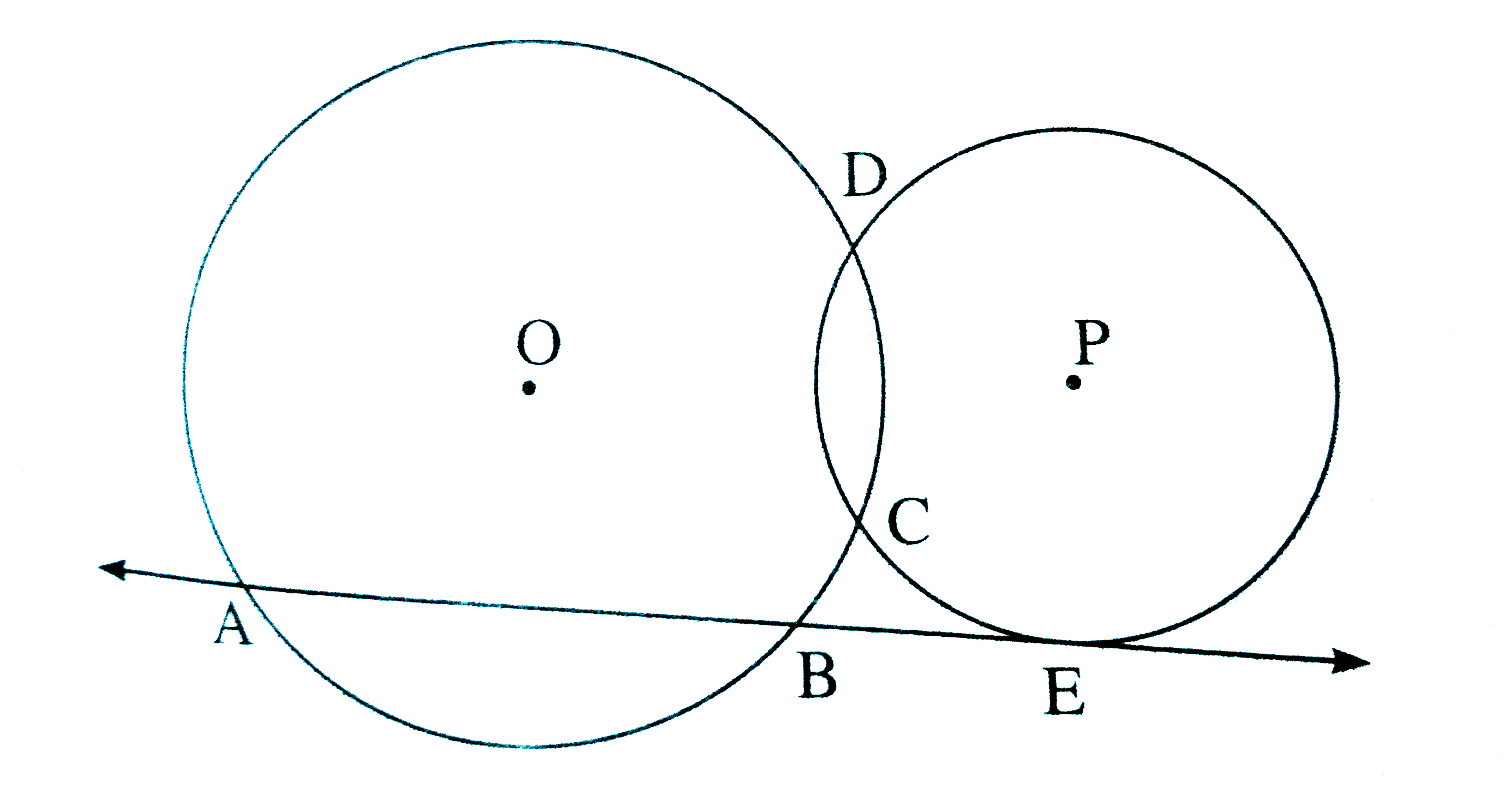
View SolutionIn the figure, two circles with centers O and P intersect each other at points D and C. Line AB intersects the circle with centre O at points A and B and touches the circle with centre P at point E. Prove ∠ADE+∠BCE=180∘.
 View Solution
View SolutionIn Figure, O and O' are centres of two circles intersecting at B and C.ACD is a straight line, find x
View SolutionIn Figure, chords AD and BC intersect each other at right angles at a point P. If ∠DAB=350, then ∠ADC= 350 (b) 450 (c) 550 (d) 650
View SolutionIn a ΔABC, if b=√3, c=1 and ∠A=300, then find a
View SolutionIn a quadrilateral ABCD, bisectors of angles A and B intersect at O such that ∠AOB=75∘, then write the value of ∠C+ ∠D
View SolutionIf AB is a chord of a circle, Pand Q are the two points on the circle different from A and B, then ∠APB= ∠AQB ∠APB+∠AQB=180∘ or∠APB= ∠AQB
View Solution

