In quadrilateral ABCD, the diagonals AC and BD intersect each other at point O.
If AO = 2CO and BO = 2DO, show that:
OA×OD=OB×OC.
To prove that OA×OD=OB×OC in quadrilateral ABCD where the diagonals AC and BD intersect at point O, and given that AO=2CO and BO=2DO, we can follow these steps:
Step 1: Express AO and BO in terms of CO and DO
Given:
- AO=2CO
- BO=2DO
Let:
- CO=x (then AO=2x)
- DO=y (then BO=2y)
Step 2: Write the segments in terms of x and y
From the definitions:
- AO=2x
- CO=x
- BO=2y
- DO=y
Step 3: Set up the ratios
Now we can set up the ratios based on the segments:
- The ratio of AO to CO is:
AOCO=2xx=2
- The ratio of BO to DO is:
BODO=2yy=2
Step 4: Equate the ratios
Since both ratios are equal to 2, we can write:
AOCO=BODO
Step 5: Cross-multiply the ratios
Cross-multiplying gives us:
AO⋅DO=BO⋅CO
Step 6: Substitute back the expressions for AO, BO, CO, and DO
Substituting the expressions we defined earlier:
(2x)⋅y=(2y)⋅x
Step 7: Simplify the equation
This simplifies to:
2xy=2xy
This confirms that the equation holds true.
Conclusion
Thus, we have shown that:
OA×OD=OB×OC
Given:
- AO=2CO
- BO=2DO
- CO=x (then AO=2x)
- DO=y (then BO=2y)
From the definitions:
- AO=2x
- CO=x
- BO=2y
- DO=y
Now we can set up the ratios based on the segments:
- The ratio of AO to CO is:
AOCO=2xx=2
- The ratio of BO to DO is:
BODO=2yy=2
Since both ratios are equal to 2, we can write:
AOCO=BODO
Cross-multiplying gives us:
AO⋅DO=BO⋅CO
Substituting the expressions we defined earlier:
(2x)⋅y=(2y)⋅x
This simplifies to:
2xy=2xy
This confirms that the equation holds true.
Thus, we have shown that:
OA×OD=OB×OC
Topper's Solved these Questions
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(B)
17 Videos
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(C)
24 Videos
- SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)BOOK - ICSECHAPTER - SIMILARITY (WITH APPLICATIONS TO MAPS AND MODELS)EXERCISE - EXERCISE 15(E)
61 Videos
- SIMILARITY BOOK - ICSECHAPTER - SIMILARITY EXERCISE - MULTIPLE CHOICE QUESTIONS (COMPETENCY BASED QUESTIONS)
10 Videos
- SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)BOOK - ICSECHAPTER - SOLVING (SIMPLE) PROBLEMS (BASED ON QUADRATIC EQUATIONS)EXERCISE - Exercise 6(e )
18 Videos
Similar Questions
Knowledge Check

The given figure shows a parallelogram ABCD whose diagonals intersect each other at point O. if OB=6 cm and AC is 6 cm more than BD, find OC.A12cmB10cmC9cmD11cm
In a trapezium ABCD, side AB is parallel to side DC, and the diagonals AC and BD intersect each other at point P. Prove that :
PA×PD=PB×PC.
View Solution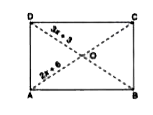
In the given figure, the diagonals AC and BD of a rectangle ABCD intersect each other at point O.
If OA=2x+6 and OD=3x+3, find the value of x.
View SolutionIn the quadrilateral ABCD, the diagonals AC and BD are equal and perpendicular to each other. What type of a quadrilateral is ABCD?
View SolutionIn rhombus ABCD, diagonals AC and BD intersect each other at point O.
If cosine of angle CAB is 0.6 and OB = 8 cm, find the lengths of the side and the diagonals of the rhombus.
View SolutionIn a rhombus ABCD the diagonals AC and BD intersect at the point (3,4) . If the point A is (1,2) the diagonal BD has the equation
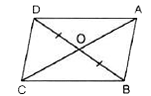
View SolutionIn the given figure, the diagonals AC and BD intersects at point O. If OB=OD and AB//DC, prove that :
(i) Area(ΔDOC)=Area(ΔAOB).
(ii) Area(ΔDCB)=Area(ΔACB).
(iii) ABCD is a parallelogram.
View SolutionIn a quadrilateral ABCD, it is given that AB∣∣CD and the diagonals ACandBD are perpendicular to each other. Show that AD.BC≥AB.CD.
View Solution
