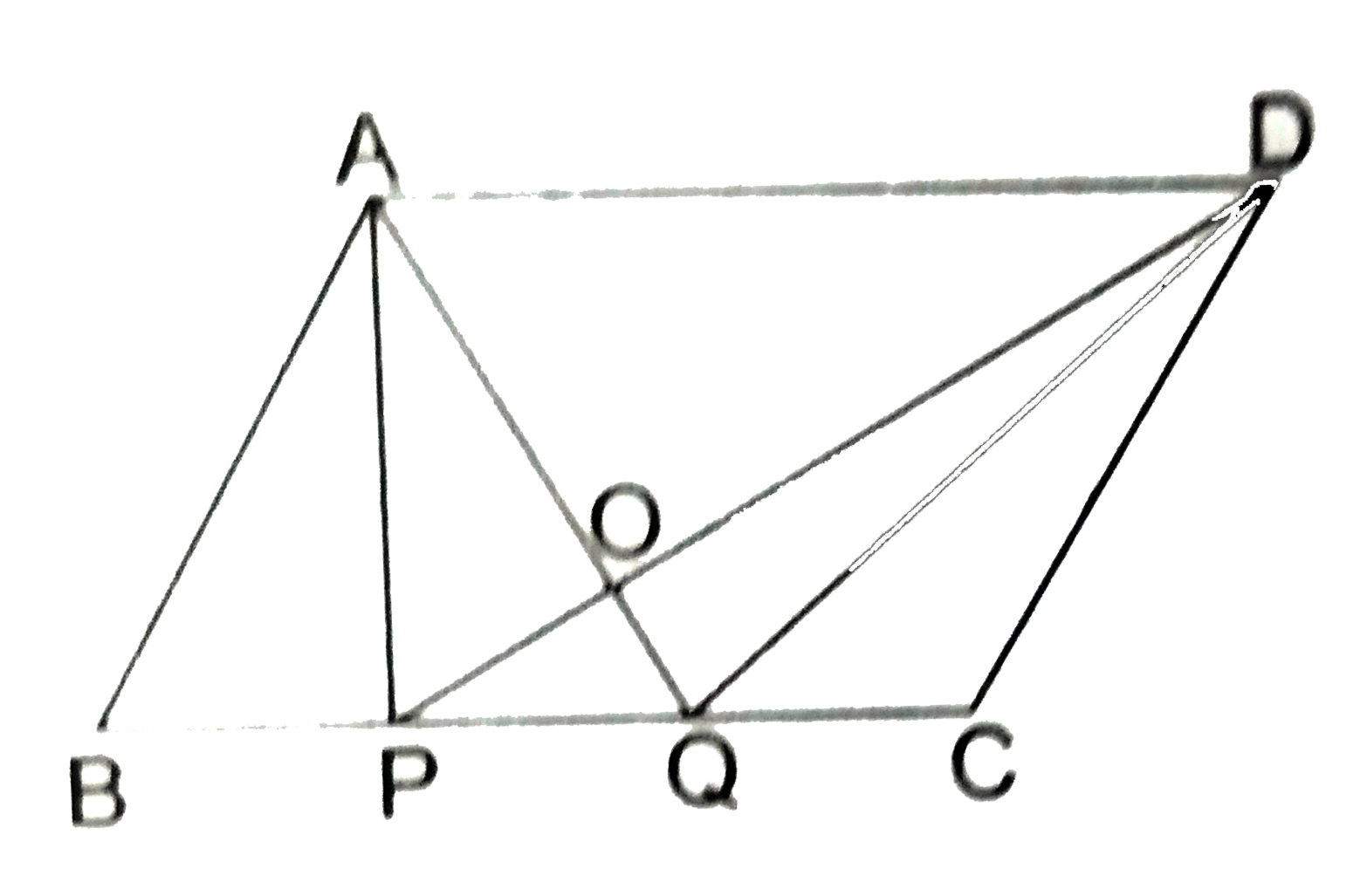
In the figure, PSDA is a parallelogram. Points Q and R are taken on PS such that PQ=QR=RS and PA||QB||RC. Prove that ar(PQE)=ar(CFD).
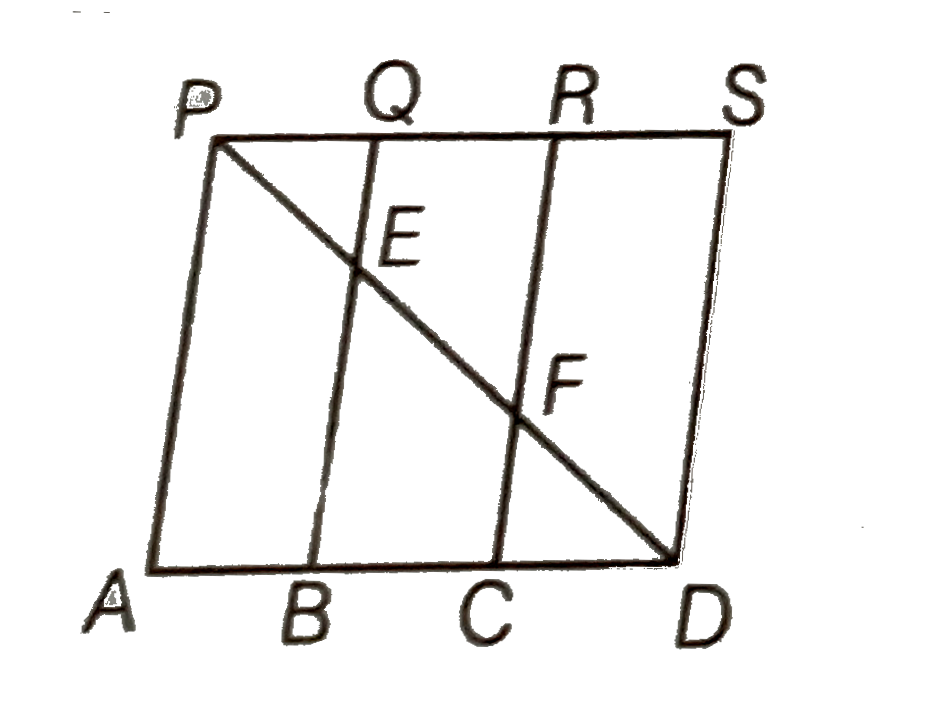
Given IN a parallelogram PSDA, points Q and R are on PS such that
PQ=QR=RS and PA||QB||RC.
To prove ar(PQE)=ar(CFD)
Proof In parallelogram PABQ,
PQ∣∣AB [∵ in parallelogram PSDA,PS∣∣AD]
and PA∣∣QB [given]
So, PABQ is a parallelogram.
∴ PQ = AB ...(i)
Similalry, QBCR is also a parallelogram.
∴ QR = BC ...(ii)
and RCDS is a parallelogram.
∴ RS = CD ...(iii)
Now, PQ = QR = RS ...(iv)
From Eqs. (i), (ii) (iii) and (iv),
PQ = QR = RS =AB = BC = CD ...(v)
In ΔPQE and ΔDCF, ∠QPE=∠FDC
[since, PS∣∣AD and PD is transversal, then alternate interior angles are equal]
PQ = CD [from Eq. (v)]
and ∠PQE=∠FCD
[∵∠PQE=∠PRC corresponding angles and ∠PRC=∠FCD alternate interior angles]
∴ ΔPQE≅ΔDCF [by ASA congruence rule]
∴ ar(ΔPQE)=ar(ΔCFD) [ since, congruent figures have equal area]
Hence proved.
Topper's Solved these Questions
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Exercise-9.4 Long Answer Type Questions
10 Videos
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Exercise-9.2 Very Short Answer Type Questions
5 Videos
- CIRCLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise 10.4
14 Videos
Similar Questions
In the figure, ABCD is a parallelogram and BC is produced to a point Q such that AD = CQ. If AQ intersect DC at P, show that ar(ΔBPC) = ar(ΔDPQ).
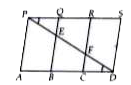
View SolutionIn the given figure PSDA is a parallelogram in which PQ =QR= RS and AP II BQ II CR II VS.Prove that ar(△PQE) = ar(△DCF).
View SolutionIn the given figure, QR is parallel to AB and DR is parallel to QB.
Prove that : PQ2=PD×PA.
View SolutionIn the figure, PQRS and ABRS are parallelograms and X is any point on side BR. Show that
ar(PQRS) = ar(ABRS)
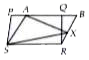
View SolutionIn the given figure, Q is a point on the side SR of ΔPSR such that PQ = PR. Prove that PS>PQ.
View SolutionIn the given figure, ABCD is a parallelogram. E and F are any two points on AB and BC, respectively. Prove that ar(△ADF)=ar(△DCE).
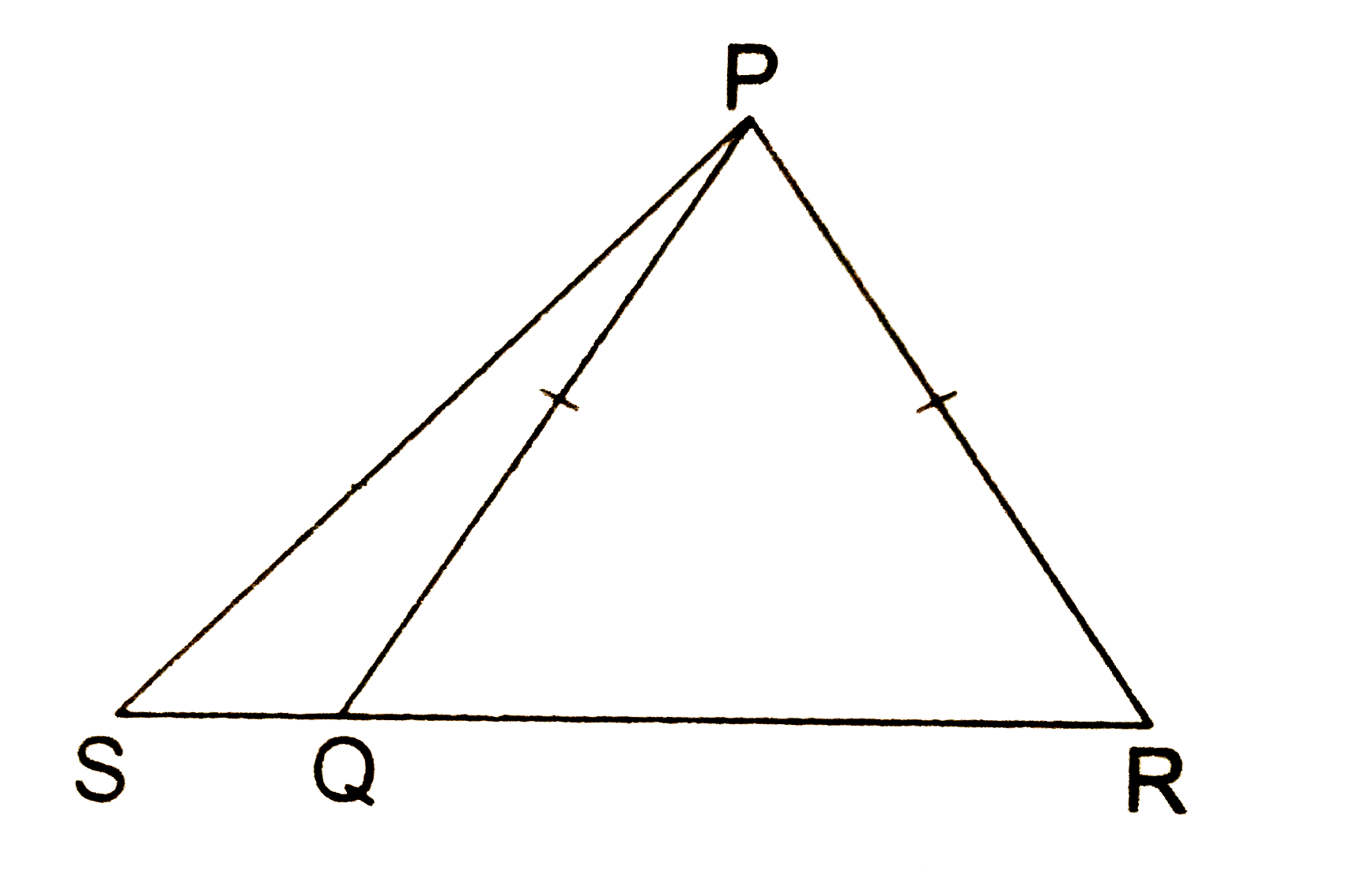
View SolutionIn the adjoining figure, ABCD is a parallelogram. Points P and Q on BC trisect BC. Prove that
ar(△APQ)=ar(△DPQ)=16ar(△ABCD).
View SolutionIn the adjoining figure, PQRS is a parallelogram. A and B are the mid-points of PQ of SR respectively. If PS= BR, then prove that quadrilateral ADBC is a reactangle.

View SolutionIn the adjoining figure, PQRS is a parallelogram. A and B are the mid-points of PQ of SR respectively. If PS= BR, then prove that quadrilateral ADBC is a reactangle.

View SolutionP is the mid-point of the side CD of a parallelogram ABCD. A line through C parallel to PA intersects AB at Q and DA produced at R. Prove that DA = AR and CQ = QR.
View Solution
