In the given figure, ∠A=60∘ and ∠ABC=80∘
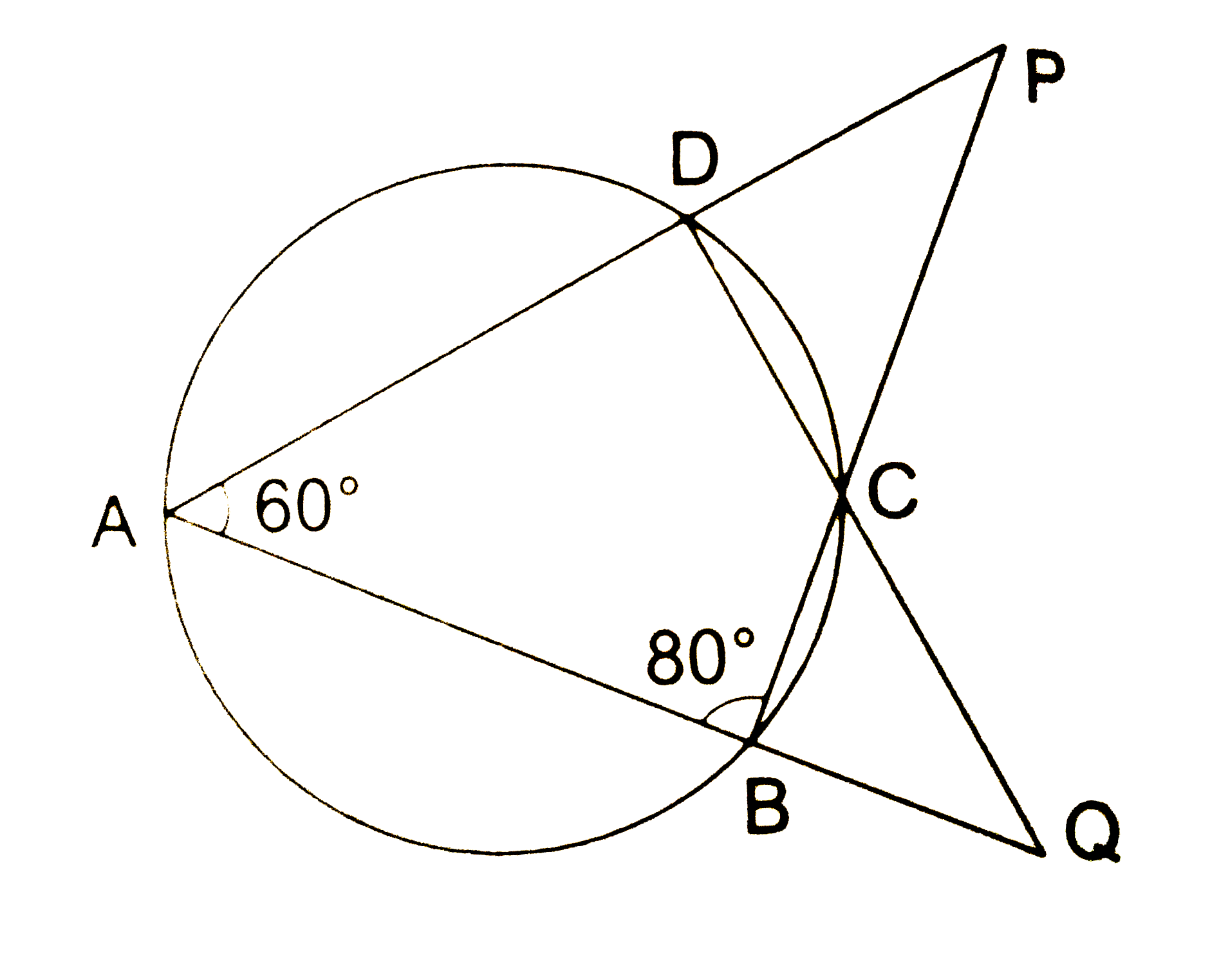 Then find ∠BQC
Then find ∠BQC
Video Solution
Text Solution
(i) Side AD of cyclic quad. ABCD has been produced to P.
∴∠PDC=∠ABC=80(∘)
[∵ ext. ∠PDC= int. opp. ∠ABC] .
Again, side of BC of quad . ABCD has been produced to P.
∴∠PCD=∠BAD=60∘[∵ ext. ∠PCD= int. opp ∠BAD].
In ΔPCD , we have
∠PDC+∠PCD+∠DPC=180∘
[∵ sum of the angles of a Δ is 180∘].
⇒80∘+60∘+∠DPC=180∘.
⇒∠DPC=180∘−140∘=40∘
Hence, ∠DPC=40∘
(ii) Since ABCD is a cyclic quadrilateral , we have
∠ABC+∠ADC=180∘
⇒80∘+∠ADC=180∘
⇒∠ADC=180∘−80∘=100∘
Again ,side DC of cyclic quad. ABCDis produced to Q.
∴∠QCB=∠BAD=60∘
[∵ ext. ∠QCB=int. opp. ∠BAD].
Also, side AB of cyclic quad. ABCD has been produced to Q.
∴∠QBC=∠ADC=100∘
[∵ ext. ∠QBC= int. opp. ∠ADC].
We know that the sum of the angles of a triangle is 180∘.
InΔQCB, we have
∠QCB+∠QBC+∠BQC=180∘
⇒60∘+100∘+∠BQC=180∘
⇒∠BQC=180∘−160∘=20∘
Hence, ∠BQC=20∘
|
Topper's Solved these Questions
- CIRCLES BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - Exercise 12A
22 Videos
- CIRCLES BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - EXERCISE 12B
18 Videos
- BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGONBOOK - RS AGGARWALCHAPTER - BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGONEXERCISE - EXERCISE 17B
16 Videos
- CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEBOOK - RS AGGARWALCHAPTER - CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEEXERCISE - Multiple Choice Questions (Mcq)
20 Videos
Similar Questions
Knowledge Check
In the given figure, <A=60∘ and <ABC=80∘, then find <BPC.
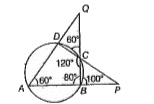 A40∘B45∘C20∘D30∘
A40∘B45∘C20∘D30∘In the given figure, ∠A=60∘and∠ABC=80∘, then find ∠BPC.
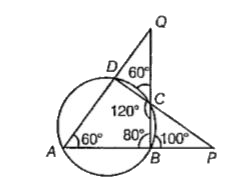 A40∘B45∘C20∘D30∘
A40∘B45∘C20∘D30∘In the given figure, ∠AOB=90∘ and ∠ABC=30∘ . Then ∠CAO=?
 A30∘B45∘C60∘D90∘
A30∘B45∘C60∘D90∘
In Figure, if ∠AOB=800and ∠ABC=300, then find ∠CAO
View SolutionIn Figure, ∠A=600 and ∠ABC=800, find ∠DPC and ∠BQC
View SolutionIn the figure, if ∠ABC=60∘ , then ∠AOC=
View Solution
In the given figure, ∠ACB=60∘,∠CAB=50∘andBC=BD. Find ∠BDC.
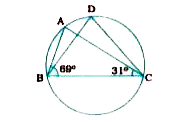
View SolutionIn the given figure, ∠ABC=69∘,∠ACB=31∘,find ∠BDC
View Solution