Lines L1≡ax+by+c=0 and L2≡lx+my+n=0 intersect at the point P and make an angle θ with each other. Find the equation of a line different from L2 which passes through P and makes the same angle θ with L1.Solution in Tamil
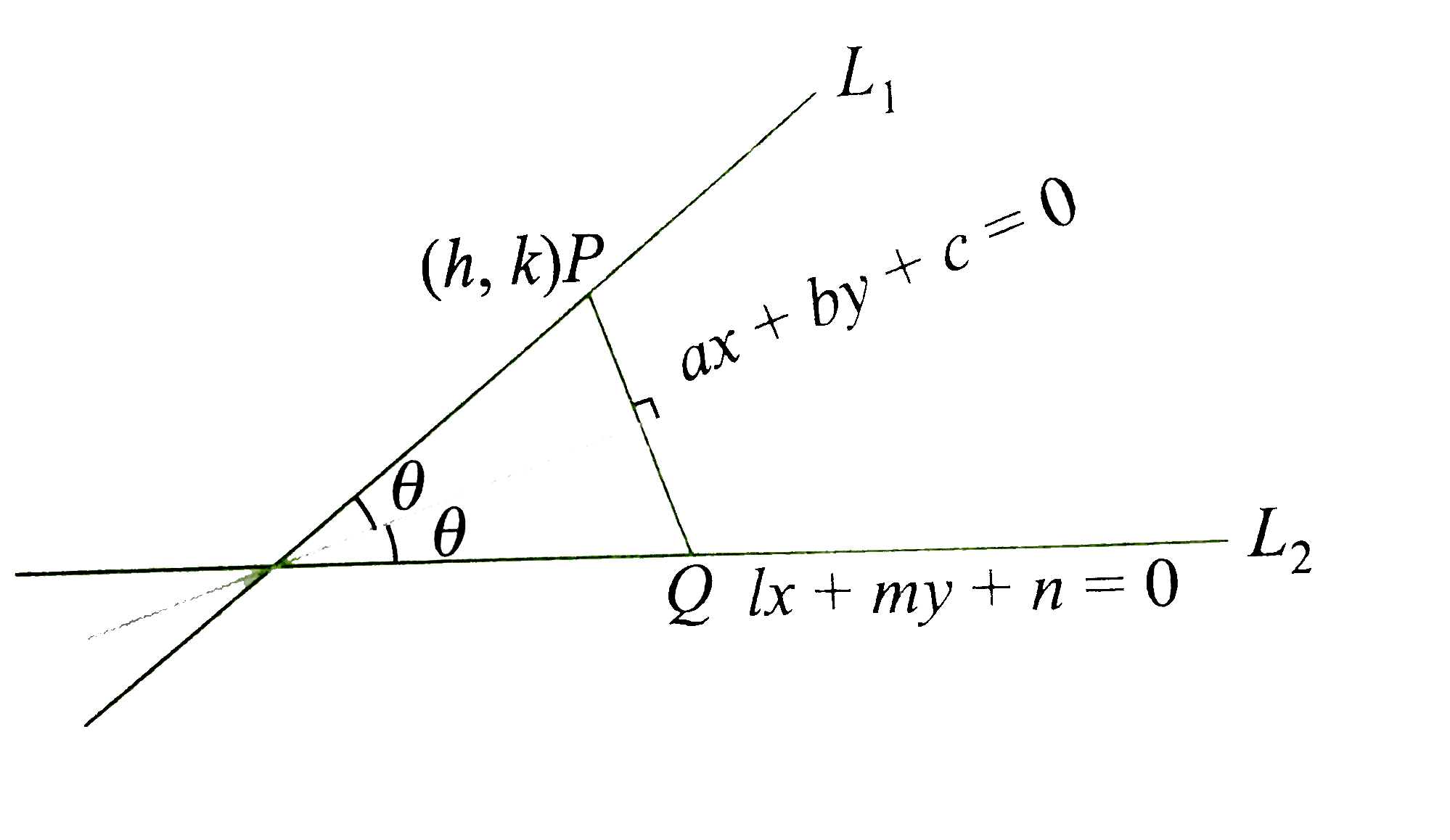
From the figure. Image of point P(h,k) on required line in the line L1 lies on line L2.
So, equation of required line is locus of point P such that its image in line L1 lies on line L2.
Let image of point P in line L1 be Q(x1,y1).
⇒x1−ha=y1−kb=2(ah+bk+c)a2+b2
⇒x1=−2a(ah+bk+c)a2+b2+h
and y1=−2b(ah+bk+c)a2+b2+k
Now ,Q(x1,y1)lies on the lineL2.
∴l[−2a(ah+bk+c)a2+b2+h]+m[−2b(ah+bk+c)a2+b2+k]+n=0
Therefore, equation of required line is
2(al+mb)(ax+by+c)−(a2+b2)(lx+my+n)=0
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.1
23 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.2
4 Videos
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year
9 Videos
Similar Questions
Find the equation of the right bisector of the line segment joining the points (3,4) and (−1,2).
View SolutionFind the points on the line x + y = 1 that lie at a distance 3 units from the line 5x+12y = 3.
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionQ. Let p and q real number such that p≠0,p2≠q and p2≠−q. if α and β are non-zero complex number satisfying α+β=−p and α3+β3=q, then a quadratic equation having αβ and βα as its roots is
View SolutionLines L1≡ax+by+c=0 and L2≡lx+my+n=0 intersect at the point P and make an angle θ with each other. Find the equation of a line different from L2 which passes through P and makes the same angle θ with L1.
View Solutionlines L_(1):ax+by+c=0 and L_(2):lx+my+n=0 intersect at the point P and make a angle theta between each other.find the equation of a line L different from L_(2) which passes through P and makes the same angle theta with L_(1)
View SolutionLet L_1 :x+y-3= 0 and L_2:7x -y +5 = 0 be two given lines. Let image of every point on L_1, with respect to a line L lies on L_2 then possible equation of L can be :
View Solutionमूलबिंदु से होकर जानेवाली उन रेखाओं के समीकरण निकालें जो रेखा x+ y = 0 से α कोण बनाती है।
View SolutionLines L1=ax+by+c=0andL2≡lx+μ+n=0 intersect at the point P and makes an angle θ with each other. Find the eqauation of a line L different from L2 which passes through P and makes the same angle θ with L1.
View SolutionLines L1=ax+by+c=0andL2=lx+my+n=0 intersect at the point P and make an anlge θ with each other. Find the equation of a line different from L2 with pass through P and makes the same anlge θ with L1.
View SolutionLet L1 be a tangent to the parabola y2=4(x+1)andL2 be a tangent to the parabola y2=8(x+2) such that L1andL2 intersect at right angles. Then L1andL2 meet on the straight line :
View SolutionLines L1≡ax+by+c=0 and L2≡lx+my+n=0 intersect at the point P and make an angle θ with each other. Find the equation of a line different from L2 which passes through P and makes the same angle θ with L1.
View SolutionThe equations of bisectors of two lines L1&L2 are 2x−16y−5=0 and 64x+8y+35=0. lf the line L1 passes through (−11,4), the equation of acute angle bisector of L1 & L2 is:
View Solutionθ1andθ2 are the inclination of lines L1andL2 with the x-axis. If L1andL2 pass through P(x1,y1), then the equation of one of the angle bisector of these lines is
View Solutionlines L1:ax+by+c=0 and L2:lx+my+n=0 intersect at the point P and make a angle θ between each other. find the equation of a line Ldifferent from L2 which passes through P and makes the same angle θ with L1
View SolutionA straight line L through the origin meet the lines x+y=1 and x+y=3 at P and Q, respectively. Lines L1andL2 are drawn , parallel to 2x-y=5 and 3x=y=5 respectively lines L1andL2 intersect at R.Then that the locus of R, as L varies, is a staright line.
View Solution
