Question

Prove that two lines that are respectively perpendicular to two intersecting lines intersect each other .

Explanation

Given Let lines l and m two interscting lines. Again, let n and p be another two lines which are perpendicular to the inersecting lines meet at point D.

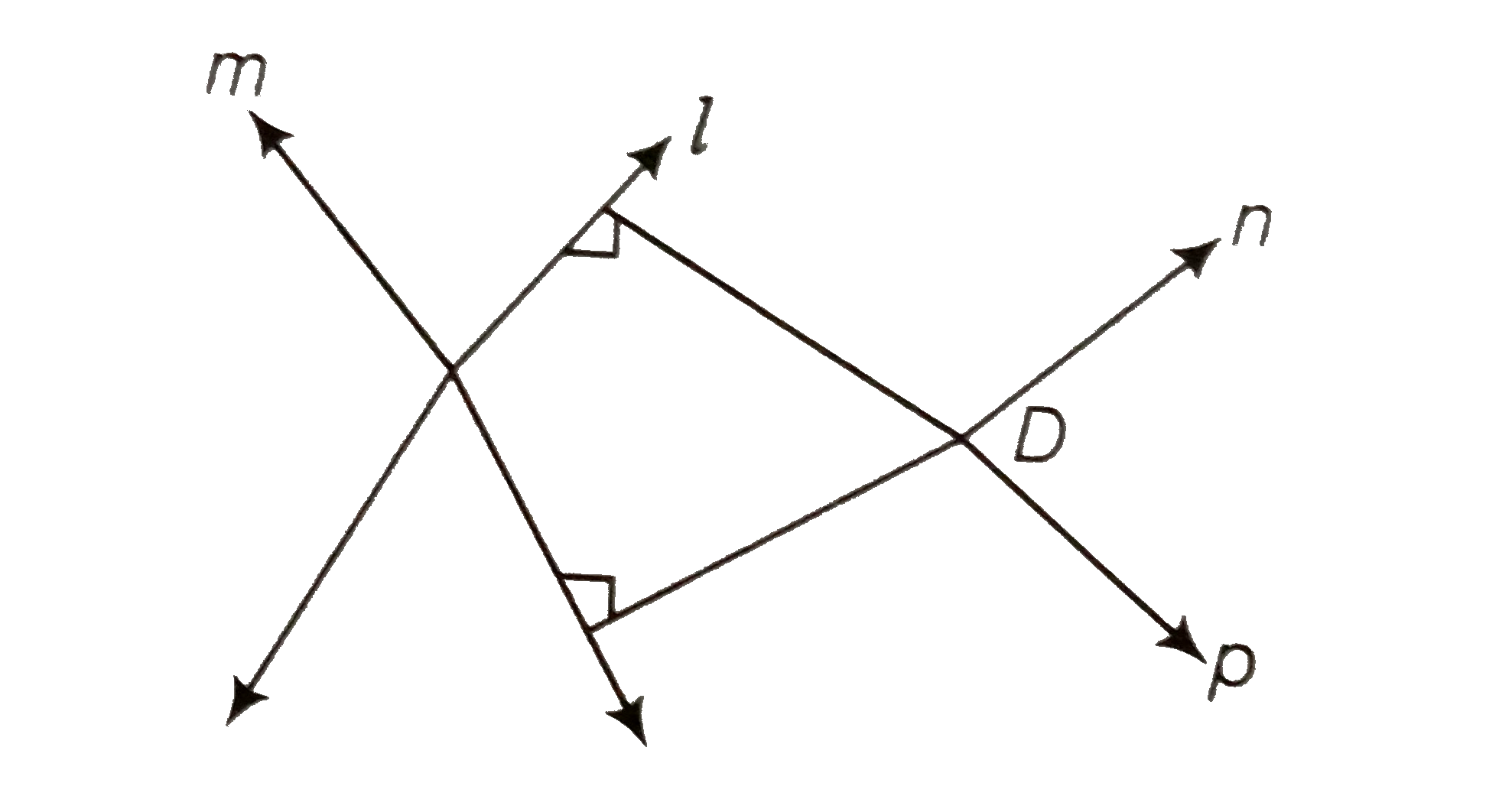
To prove Two lines n and p intersecting at a point.
Proof Suppose we consider lines n and p are not intersecting, then it means they are parallel to each other i.e.. n`|""|`p.
Since, lines n and p are perpendicular to m and l, respectievly .
But from Eq. (i) n`|""|`p, it implies that l`|""|`m.
Thus, our assumption is wrong.
Therefore, lines n and p intersect at a point.