Complete Solutions to TOPIC-1 BASIC PROPORTIONALITY THEOREM (MULTIPLE CHOICE QUESTIONS) of chapter TRIANGLES of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | TRIANGLES |
| OSWAAL PUBLICATION | TOPIC-1 BASIC PROPORTIONALITY THEOREM (MULTIPLE CHOICE QUESTIONS) |
| Number Of Questions | 30 |
| Academic Year | 2023-24 |
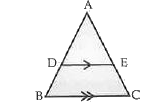
In the adjoining figure, D and E are the mid-points of AB and AC respectively. If `DE = 4 cm`, then BC is equal to :
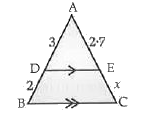
View SolutionIn `Delta ABC`, `DE || BC`, If `AD = 3 cm`, `BD = 2 cm` and `AE = 2.7`, then AC is equal to :
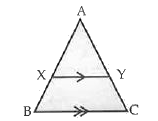
View SolutionIn the adjoining figure, `XY || BC`, then `(AX)/(AB)` is equal to :
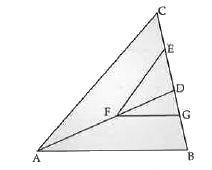
View SolutionIn the given figure, `EF || CA` and `FG || AB` then `(DE)/(EC)` is equal to :
View SolutionIn the given figure, `AB || PQ`. If `PQ = 1.5 cm`, `QC = 2 cm` and `RQ = 8 cm`, then measure of `AB's` :
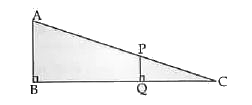
View SolutionIn the given figure, `XY || BC` and `XZ || AC` then `(AX)/(AB)` is equal to :
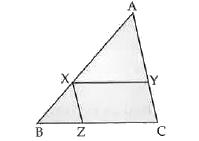
View SolutionABCD is a trapezium in which `AB||DC` and its diagonals intersect each other at the point O. Show that `(AO)/(BO) =(CO)/(DO)`
View SolutionIn `Delta ABC` , D and E are the mid-points of AB and AC respectively, then the area of `Delta ADE` is :
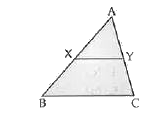
View SolutionIn the given figure, `XY || BC`, then `(AX)/(BX)`
View SolutionIn `Delta ABC`, `PQ || AB`. The correct relation is :
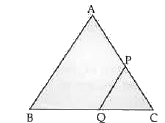
View SolutionIn the given figure `/_ ABC - /_ AYX`, then the ratio of the corresponding sides is :
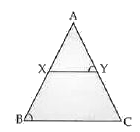
View SolutionIn the figure, `DE || BC`, `AD : AB = 1 : 2`, `BC = 6 cm`, then `DE` is :
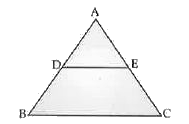
View SolutionIn a trapezium ABCD, `AB || DC`, Which of the following is a correct statement ?
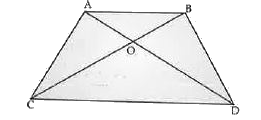
View SolutionIn the following figure `DE || AB`. If `AD = 7 cm`, `CE = 10 cm` and `CD = 5 cm`, then the length of `BE =`
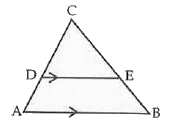
View SolutionIn the figure, `XY || BC`, `AX = 9 cm`, `XB = 4.5 cm` and `BC = 18cm`, then `XY =`
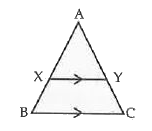
View Solutionin the figure, `MN || PR`. If `QN : NR = 2 : 3` and `PQ = 11.5 cm` then `QM` is equal to :
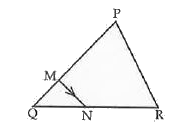
View SolutionIn a trapezium ABCD, `AB || DC`, Which of the following is a correct statement ?

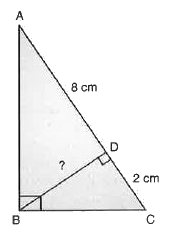
View SolutionIt the following figure, `/_ ABC = 90^(@)` and `BD _|_ AC`. If `AD = 8 cm`, CD = 2 cm, then the length of BD is :
View SolutionThe corresponding sides of two equiangular triangles are :
View SolutionIn the given `Delta ABC =~ Delta PQC`. The ratio of their corresponding sides is :
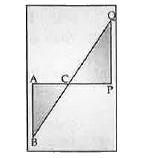
View SolutionIn the figure, `/_ ABC = /_ AQP = 90^(@)`, then `(AQ)/(AB)`
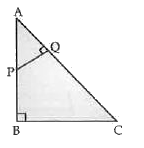
View SolutionIn `Delta ABC` and `Delta DEF` is `(AB)/(DE) = (BC)/(DF) then they will be similar if:
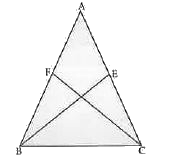
View SolutionIn the figure `Delta ABC`, `BE _|_ AC` and `CF _|_ AB,`,`AD _|_ BC,`. then AF2 + BD2 + CE2 =
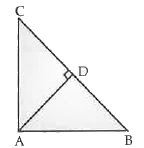
View SolutionIn a right-angled triangle ABC, `/_ CAB = 90^(@)`. If `AD _|_ BC`, then the angle equal to `/_ ACD` is :
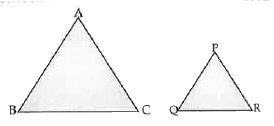
View SolutionIf the given triangles are similar, then the ratio between their sides is :
View SolutionSides of a triangle are of length 2 cm, 3 cm and 4 cm respectively. Which of the sets of number are the sides of a triangle, similar to the above trianlge ?
View SolutionIn the figure `/_ APQ = /_ ACB` and `/_ AQP = /_ ABC`. Then AP.AB is equal to :
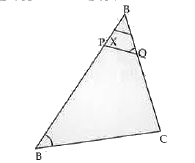
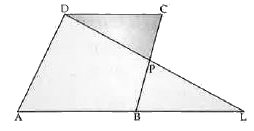
View SolutionIn parallelogram `ABCD`, P is a point on BC. In `Delta DCP` and `Delta BLP`, `DP : PL` is equal to :
View SolutionSelect the set of numbers from the following which can form the similar triangles.
View Solution`Delta ABC` has sides of length 5 cm, 6 cm and 7 cm. The perimeter of `Delta DEF` is 360 cm. If `Delta ABC ~= Delta DEF` then the ratio of the ratio of the perimeters of `Delta ABC` and `Delta DEF` is :
View Solution
TOPIC-1 BASIC PROPORTIONALITY THEOREM (MULTIPLE CHOICE QUESTIONS) questions and complete solutions for chapter TRIANGLES of OSWAAL PUBLICATION MATHS (KANNADA ENGLISH) of Class 10
All Exercises from OSWAAL PUBLICATION Class 10 MATHS TRIANGLES
- TEXTBOOK CORNER (EXERCISE 2.1)
- TEXTBOOK CORNER (EXERCISE 2.2)
- TEXTBOOK CORNER (EXERCISE 2.3)
- TEXTBOOK CORNER (EXERCISE 2.4)
- TEXTBOOK CORNER (EXERCISE 2.5)
- TEXTBOOK CORNER (EXERCISE 2.6)
- TOPIC-1 BASIC PROPORTIONALITY THEOREM (LONG ANSWER TYPE QUESTIONS)
- TOPIC-1 BASIC PROPORTIONALITY THEOREM (LONG ANSWER TYPE QUESTIONS-(II))
- TOPIC-1 BASIC PROPORTIONALITY THEOREM (MULTIPLE CHOICE QUESTIONS)
- TOPIC-1 BASIC PROPORTIONALITY THEOREM (SHORT ANSWER TYPE QUESTIONS)
- TOPIC-1 BASIC PROPORTIONALITY THEOREM (VERY SHORT ANSWER TYPE QUESTIONS)
- TOPIC-3 AREA OF SIMILAR TRIANGLES (LONG ANSWER TYPE QUESTIONS)
- TOPIC-3 AREA OF SIMILAR TRIANGLES (LONG ANSWER TYPE QUESTIONS-(II))
- TOPIC-3 AREA OF SIMILAR TRIANGLES (MULTIPLE CHOICE QUESTIONS)
- TOPIC-3 AREA OF SIMILAR TRIANGLES (SHORT ANSWER TYPE QUESTIONS)
- TOPIC-3 AREA OF SIMILAR TRIANGLES (VERY SHORT ANSWER TYPE QUESTIONS)
- TOPIC-4 PYTHAGOREAN TRIPLETS (LONG ANSWER TYPE QUESTIONS)
- TOPIC-4 PYTHAGOREAN TRIPLETS (LONG ANSWER TYPE QUESTIONS-(II))
- TOPIC-4 PYTHAGOREAN TRIPLETS (MULTIPLE CHOICE QUESTIONS)
- TOPIC-4 PYTHAGOREAN TRIPLETS (SHORT ANSWER TYPE QUESTIONS)
- TOPIC-4 PYTHAGOREAN TRIPLETS (VERY SHORT ANSWER TYPE QUESTIONS)
- TOPIC-5 PYTHAGORAS THEOREM (LONG ANSWER TYPE QUESTIONS)
- TOPIC-5 PYTHAGORAS THEOREM (LONG ANSWER TYPE QUESTIONS-(II))
- TOPIC-5 PYTHAGORAS THEOREM (SHORT ANSWER TYPE QUESTIONS)